Two circle with radii $r_{1}$ and $r_{2}$ respectively touch each other externally. Let $r_{3}$ be the radius of a circle that touches these two circle as well as a common tangents to two circles then which of the following relation is trueSolution in Tamil

A

$\frac{1}{\sqrt{a}} - \frac{1}{\sqrt{b}} = \frac{1}{\sqrt{c}}$

B

$c = \frac{2 a b}{a + b}$

C

$\frac{1}{\sqrt{a}} + \frac{1}{\sqrt{b}} = \frac{1}{\sqrt{c}}$

D

$c = \frac{2 a b}{\sqrt{a} + \sqrt{b}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:3

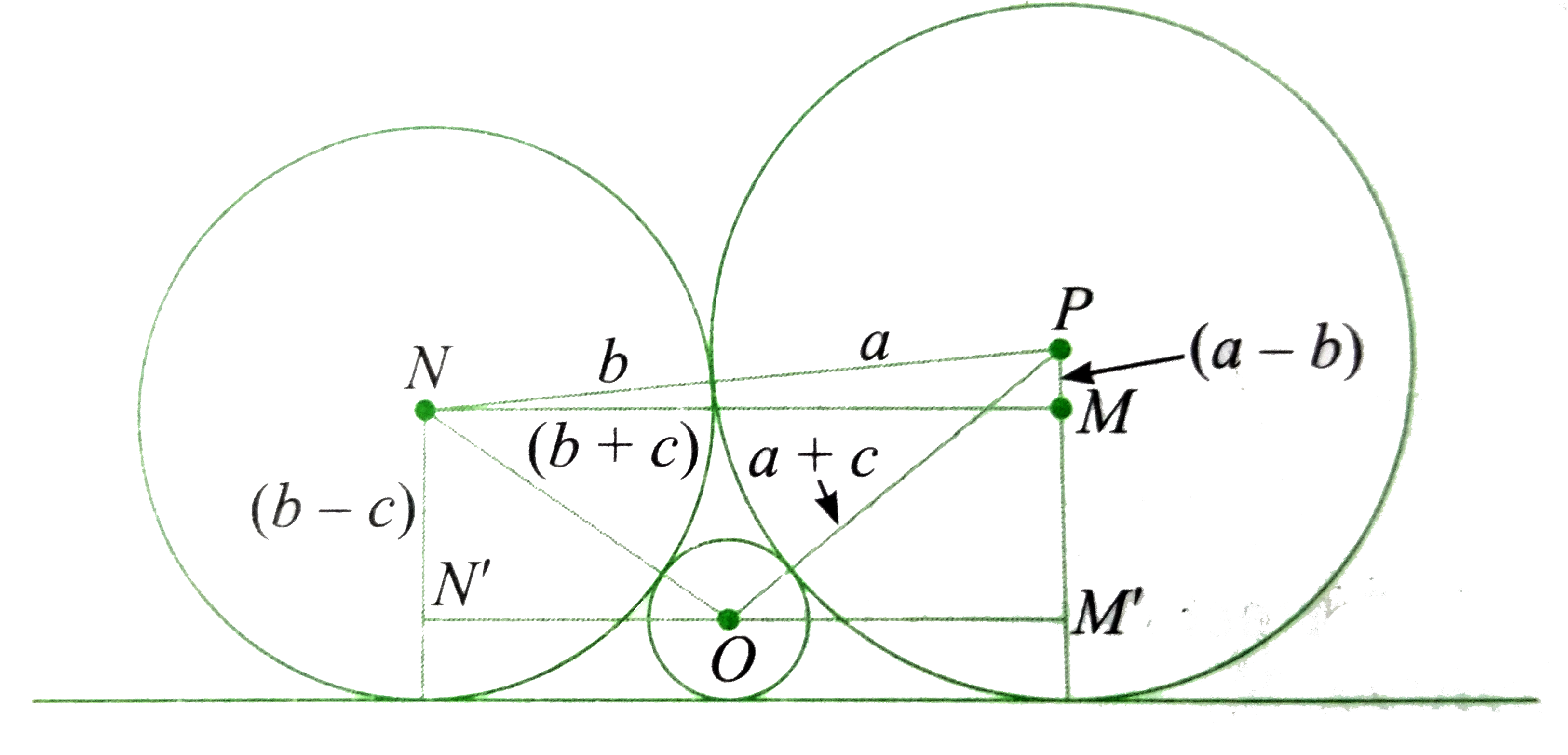
$∵ M' N' = M N$
Now, $M N = \sqrt{{(a + b)}^{2} + {(a - b)}^{2}} = \sqrt{4 a b}$
$N' O = \sqrt{{(b + c)}^{2} - {(b - c)}^{2}} = \sqrt{4 b c}$
$M' O = \sqrt{{(b + c)}^{2} - {(a - c)}^{2}} = \sqrt{4 a c}$
Now, $N' O + M' O = M N$
$\Rightarrow 2 \sqrt{a b} = 2 \sqrt{b c} + 2 \sqrt{a c}$
Dividing by $\frac{1}{\sqrt{a b c}}$ , we get
$\frac{1}{\sqrt{c}} = \frac{1}{\sqrt{a}} + \frac{1}{\sqrt{b}}$

Updated on:21/07/2023

|

Class 12 MATHS CIRCLE

Topper's Solved these Questions

Similar Questions

CENGAGE-CIRCLE -Exercise (Single)

Home
Ask Doubt
Profile