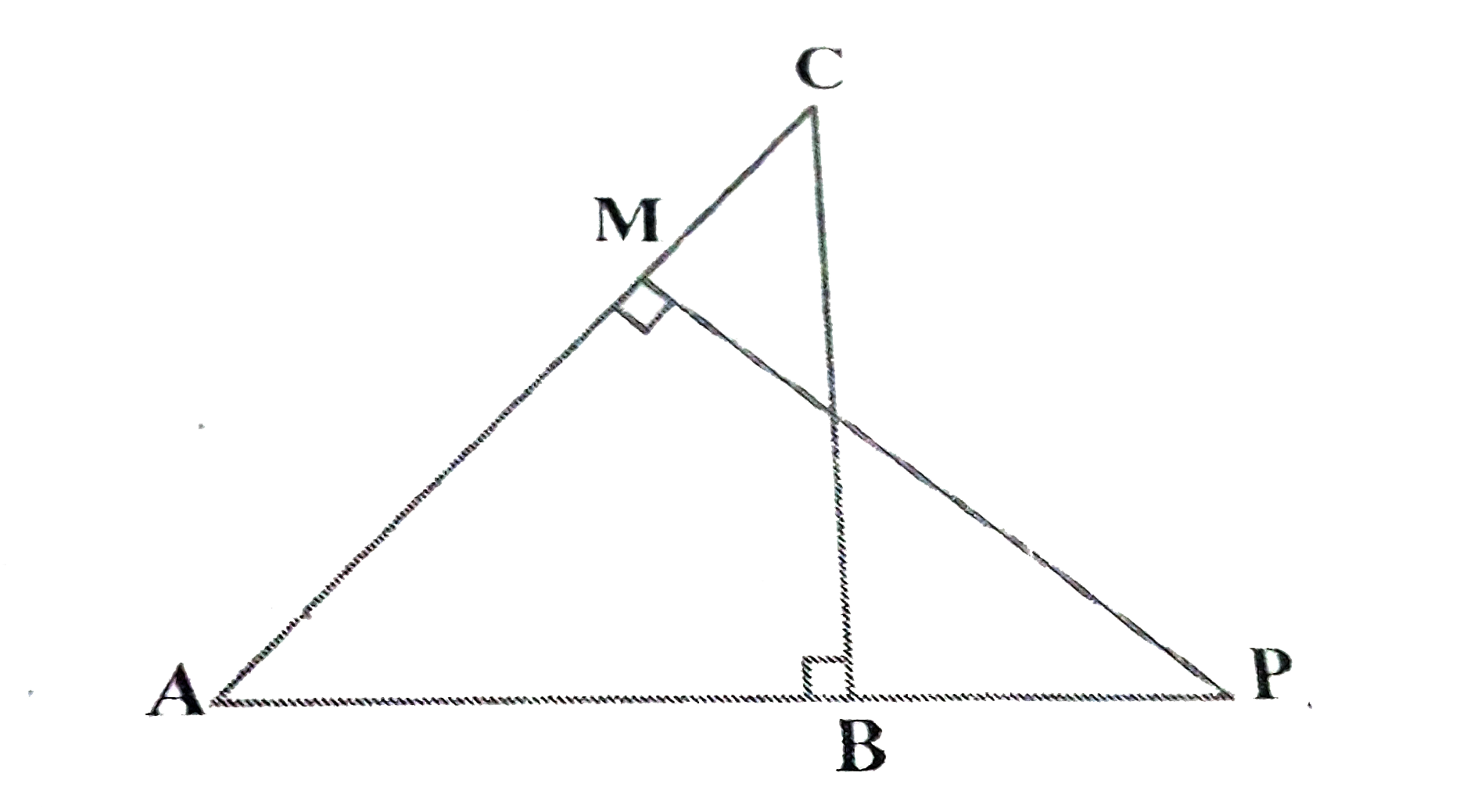
a)Find out the angles of the triangles APC and BPC in the figure.
'(##VPU_HSS_MAT_IX_C07_E04_016_Q01##)'
b) What is the relation between the sides of these triangles?
c) Prove that 'A P xx B P=C P^2'. Solution in Malayalam
Step by step video & image solution for a)Find out the angles of the triangles APC and BPC in the figure. '(##VPU_HSS_MAT_IX_C07_E04_016_Q01##)' b) What is the relation between the sides of these triangles? c) Prove that 'A P xx B P=C P^2'. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Find the distance between the two points on the number line, denoted by each pair of numbers given below:
i) 1,−5.
ii) 12,23
iii) −12,−13.
iy) −12,34
View SolutionThe weight of 6 players in a volley ball team are all different and the average weight is 60 kilograms
i) Prove that the team has at least one player weighing more than 60 kilograms.
ii) Prove that the team has at least one player weighing less than 60 kilograms.
View SolutionIn figure ABC and AMP are two right triangles, right angles at B and M respectively. Prove that(i) ΔABC~ΔAMP (ii) CAPA=BCMP
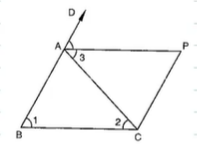
View SolutionIn Figure, ABC is an isosceles triangle in which AB=AC.CPparel≤lAB and AP is the bisector of exterior ∠CAD of ABC . Prove that ∠PAC=∠BCA and (ii) ABCP is a parallelogram.
View SolutionLet P be the point inside that ΔABC. Such that ∠APB=∠BPC=∠CPA. Prove that
PA+PB+PC=√a2+b2+c22+2√3Δ,wherea,b,cΔ are the sides and the area of ΔABC.
View SolutionLet A(5,−3),B(2,7)andC(−1,2) be the vertices of a △ABC. If P is a point inside the triangle ABC such that the triangle APC,APB and BPC have equal areas, then length of the line segment PB is:
View SolutionIn figure ABC and AMP are two right triangles, right angles at B and M respectively. Prove that(i) ΔABC~ΔAMP (ii) CAPA=BCMP

View SolutionIf a,b,c are in A.P. and p is a real number and
Δ=∣∣ ∣ ∣∣p+cp+2p+ap+bp+5p+bp+ap+8p+c∣∣ ∣ ∣∣
then Δ equals
View SolutionIn Figure, ABC is an isosceles triangle in which AB=AC.andCP∣∣AB and AP is the bisector of exterior ∠CAD of ABC . Prove that ∠PAC=∠BCA and (ii) ABCP is a parallelogram.
View SolutionABC is a triangle right angled at B;and P is the mid-point of AC . Prove that: PQ ⊥AB (ii) Q is the mid point of AB PB=PA=12AC
View SolutionLet A(5,-3), B(2,7) and C(-1,2) be the vertices of a triangle A,B,C. If P is a point inside the triangle ABC such that the triangle APC,APB and BPC have equal areas, then length of the line segment PB is:
View SolutionMycorrhizal association is responsible for br a- Increasing absorptive area, br b- Providing shelter to fungi, br c- Nitrogen fixation, br d- Enhanced supply of N, P, S
View SolutionIn a ΔABC, angle bisector of interior angle B and exterior angle C intersect at P . If ∠A=70∘ . Find ∠BPC.
View SolutionIn a ΔABC, angle bisector of exterior ∠B&∠C meets at point P, ∠BPC=65∘ then find ∠A .
View SolutionΔABC ,angle B external angle C meets at P ∠A=80 then ∠BPC = ?
View SolutionIf in a ΔABC angle bisectors of exterior angle ∠Band∠C intersect at P ∠BPC=40∘ find ∠A
View Solution
