PQRS is a rectangle. The perpendicular ST from S on PR divides ∠S in the ratio 2:3. Find ∠TPQ.
To solve the problem, we will follow these steps:
Step 1: Understand the Geometry
We have a rectangle PQRS, where S is the vertex from which a perpendicular ST is drawn to the diagonal PR. The angle at S is divided by ST into two parts in the ratio 2:3.
Step 2: Assign Variables to Angles
Let:
- ∠PST = 2x
- ∠RST = 3x
Step 3: Use the Angle Sum Property
Since the angles PST and RST together form the angle PSR, we can write:
∠PSR=∠PST+∠RST=2x+3x=5x
In a rectangle, the diagonal PR divides the angle PSR into two angles, and we know that ∠PSR is equal to 90 degrees (because the sum of angles in a rectangle at any vertex is 90 degrees).
Step 4: Set Up the Equation
From the above, we have:
5x=90
Step 5: Solve for x
Now, we can solve for x:
x=905=18
Step 6: Find the Angles PST and RST
Now we can find the angles:
- ∠PST = 2x = 2(18) = 36 degrees
- ∠RST = 3x = 3(18) = 54 degrees
Step 7: Find Angle TPQ
Since PQRS is a rectangle, we know that:
∠TPQ=∠RST=54 degrees
Thus, the final answer is:
∠TPQ=54 degrees
---
We have a rectangle PQRS, where S is the vertex from which a perpendicular ST is drawn to the diagonal PR. The angle at S is divided by ST into two parts in the ratio 2:3.
Let:
- ∠PST = 2x
- ∠RST = 3x
Since the angles PST and RST together form the angle PSR, we can write:
∠PSR=∠PST+∠RST=2x+3x=5x
In a rectangle, the diagonal PR divides the angle PSR into two angles, and we know that ∠PSR is equal to 90 degrees (because the sum of angles in a rectangle at any vertex is 90 degrees).
From the above, we have:
5x=90
Now, we can solve for x:
x=905=18
Now we can find the angles:
- ∠PST = 2x = 2(18) = 36 degrees
- ∠RST = 3x = 3(18) = 54 degrees
Since PQRS is a rectangle, we know that:
∠TPQ=∠RST=54 degrees
∠TPQ=54 degrees
Topper's Solved these Questions
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - APPLICATIONS, GAMES AND PUZZLES
3 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - THINK AND DISCUSS
2 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - THINK AND DISCUSS
2 Videos
- SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTBOOK - NCERT EXEMPLARCHAPTER - SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTEXERCISE - Exercise
52 Videos
- VISUALISING SOLID SHAPES BOOK - NCERT EXEMPLARCHAPTER - VISUALISING SOLID SHAPES EXERCISE - EXERCISE
91 Videos
Similar Questions
Knowledge Check
PQRS is a parallelogram in which PR is perpendicular to QS. If PR=8cm and QS=6 cm, then find PS
A5cmB4cmC7cmD6cmPQRS is a parallelogram in which PR is perpendicular to QS. If PR=8cm and QS=6 cm, then find PS
A5 cmB4 cmC7 cmD6 cmIn ∠PQR,PQ=6cm,PR=9cm and M is a point on QR such that it divides QR in the ratio 1:2PM⊥QR. Find QR.
A√18 cmB3√12 cmC3√15 cmD√20 cm
13. Two supplementary angles are in the ratio 3:2 Find the angles. 14 Two complementary angles are in the ratio 4:5. Find the angles.
View SolutionPQRS is a rectangle. The ratio of the sides PQ and QR is 3:1. If the length of the diagonal PR is 10cm, then what is the area (in cm2) of the rectangle?
View SolutionPQR is a triangle right angled at P. If PQ=10cmandPR=24cm, find QR
View SolutionIn the figure below, PQR is a right-angled triangle, right angled at P. A perpendicular line PS is drawn from P to QR. PR = 5 cm and PQ = 12 cm.
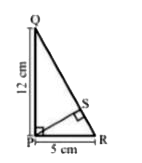
What is RS:SQ?
View SolutionPQRS is a rhombus in which ∠R = 60°. Then PR : QS =
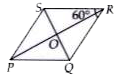
View Solution
