Ratio of area of AMNO to the area of parallelogram MNOP in the same figure 9.17 is
2:3
1:1
1:2
2:1
To find the ratio of the area of triangle AMO to the area of parallelogram MNOP, we can follow these steps:
Step 1: Understand the Areas
We need to find the areas of triangle AMO and parallelogram MNOP.
Step 2: Area of Triangle AMO
The formula for the area of a triangle is:
Area of triangle=12×Base×Height
In triangle AMO, we can take:
- Base = ON
- Height = OQ (the perpendicular drawn from point O to line MN)
Thus, the area of triangle AMO is:
Area of triangle AMO=12×ON×OQ
Step 3: Area of Parallelogram MNOP
The formula for the area of a parallelogram is:
Area of parallelogram=Base×Height
For parallelogram MNOP, we can take:
- Base = MP
- Height = OQ (the perpendicular height from point O to line MP)
Thus, the area of parallelogram MNOP is:
Area of parallelogram MNOP=MP×OQ
Step 4: Relate the Bases
From the properties of parallelograms, we know that opposite sides are equal. Therefore:
MP=NO
So we can substitute MP with NO in the area of the parallelogram:
Area of parallelogram MNOP=NO×OQ
Step 5: Calculate the Ratio
Now we can find the ratio of the area of triangle AMO to the area of parallelogram MNOP:
Ratio=Area of triangle AMOArea of parallelogram MNOP=12×ON×OQNO×OQ
Step 6: Simplify the Ratio
Since OQ is common in both the numerator and denominator, we can cancel it out:
Ratio=12×ONNO
Given that ON = NO (as they are equal sides of the parallelogram), we can substitute ON with NO:
Ratio=12×NONO=12
Final Step: Write the Ratio
Thus, the ratio of the area of triangle AMO to the area of parallelogram MNOP is:
Ratio=1:2
We need to find the areas of triangle AMO and parallelogram MNOP.
The formula for the area of a triangle is:
Area of triangle=12×Base×Height
In triangle AMO, we can take:
- Base = ON
- Height = OQ (the perpendicular drawn from point O to line MN)
Area of triangle AMO=12×ON×OQ
The formula for the area of a parallelogram is:
Area of parallelogram=Base×Height
For parallelogram MNOP, we can take:
- Base = MP
- Height = OQ (the perpendicular height from point O to line MP)
Area of parallelogram MNOP=MP×OQ
From the properties of parallelograms, we know that opposite sides are equal. Therefore:
MP=NO
So we can substitute MP with NO in the area of the parallelogram:
Area of parallelogram MNOP=NO×OQ
Now we can find the ratio of the area of triangle AMO to the area of parallelogram MNOP:
Ratio=Area of triangle AMOArea of parallelogram MNOP=12×ON×OQNO×OQ
Since OQ is common in both the numerator and denominator, we can cancel it out:
Ratio=12×ONNO
Given that ON = NO (as they are equal sides of the parallelogram), we can substitute ON with NO:
Ratio=12×NONO=12
Thus, the ratio of the area of triangle AMO to the area of parallelogram MNOP is:
Ratio=1:2
Topper's Solved these Questions
- PERIMETER AND AREABook:NCERT EXEMPLARChapter:PERIMETER AND AREAExercise:Exercise (Fill in the blanks)
Explore 19 Videos
- PERIMETER AND AREABook:NCERT EXEMPLARChapter:PERIMETER AND AREAExercise:Exercise (True or false)
Explore 7 Videos
- PERIMETER AND AREABook:NCERT EXEMPLARChapter:PERIMETER AND AREAExercise:Think and Discuss
Explore 7 Videos
- LINES AND ANGLES Book:NCERT EXEMPLARChapter:LINES AND ANGLES Exercise:Exercise (fill in the blanks)
Explore 3 Videos
- PRACTICAL GEOMETRY SYMMETRY AND VISUALISING SOLID SHAPESBook:NCERT EXEMPLARChapter:PRACTICAL GEOMETRY SYMMETRY AND VISUALISING SOLID SHAPESExercise:EXERCISE
Explore 108 Videos
Similar Questions
Knowledge Check
The adjacent sides of a parallelogram are 6 cm and 8 cm and the angle between them is 30∘ . What is the area of the parallelogram ?
A72 sq unitsB4 sq unitsC112 sq unitsD7 sq unitsIn the figure, the area of parallelogram ABCD is
 AAB×BMBBC×BNCDC×DLDAD×DL
AAB×BMBBC×BNCDC×DLDAD×DLThe base of a triangle is one-fifth of the base of a parallelogram having the same area as that of the triangle . The ratio of the corresponding heights of the triangle and the parallelogram will be
A5:1B1:10C1:5D10:1
In Fig. 9.29 ratio of the area of triangle ABC to the area of triangle ACD is the same as the ratio of base BC of triangle ABC to the base CD of triangle ACD.
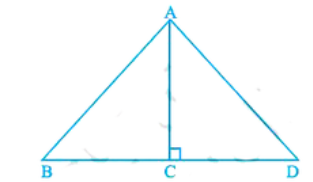
View SolutionArea and Perimeter of Parallelogram
View SolutionThe base of a triangle is half the base of a parallelogram having the same area as that of the triangle. The ratio of the corresponding heights of the triangle to the parallelogram will be :
View SolutionThe ratio of the area of a square to the area of a regular hexagon, having the same perimeter is:
View SolutionThe ratio of the areas of the triangle and a parallelogram in same parallels and on the same base is :
View Solution