In an office of 1200 employees, the ratio of urban to rural members of staff is 8 : 7. After joining some new employees, out of which 20 are rural, the ratio becomes 5 : 4. The number of new urban employees is:
1200 कर्मचारियों वाले एक कार्यालय में शहरी और ग्रामीण सदस्यों का अनुपात 8 : 7 है | कुछ नए कर्मचारियों, जिनमें से 20 ग्रामीण हैं, के शामिल होने के बाद यह अनुपात 5 : 4 हो जाता है | नए शहरी कर्मचारियों की संख्या ज्ञात करें |
a)100
b)85
c)76
d)108
To solve the problem, we will follow these steps:
Step 1: Determine the initial number of urban and rural employees.
The total number of employees is 1200, and the ratio of urban to rural employees is 8:7.
Let the parts of urban employees be 8x and rural employees be 7x.
The total parts = 8+7=15.
To find x:
15x=1200⟹x=120015=80
Now, calculate the number of urban and rural employees:
Urban employees=8x=8×80=640
Rural employees=7x=7×80=560
Step 2: Set up the equation after new employees join.
After some new employees join, including 20 rural employees, the new ratio of urban to rural employees becomes 5:4.
Let y be the number of new urban employees. The new number of urban employees will be 640+y and the new number of rural employees will be 560+20=580.
Step 3: Write the ratio equation.
The new ratio can be expressed as:
640+y580=54
Step 4: Cross-multiply to solve for y.
Cross-multiplying gives:
4(640+y)=5×580
Calculating the right side:
5×580=2900
Now, the equation becomes:
4(640+y)=2900
Step 5: Expand and simplify the equation.
Expanding the left side:
2560+4y=2900
Step 6: Isolate y.
Subtract 2560 from both sides:
4y=2900−2560
Calculating the right side:
4y=340
Step 7: Solve for y.
Dividing both sides by 4:
y=3404=85
Conclusion:
The number of new urban employees is 85.
---
The total number of employees is 1200, and the ratio of urban to rural employees is 8:7.
15x=1200⟹x=120015=80
Urban employees=8x=8×80=640
Rural employees=7x=7×80=560
After some new employees join, including 20 rural employees, the new ratio of urban to rural employees becomes 5:4.
The new ratio can be expressed as:
640+y580=54
Cross-multiplying gives:
4(640+y)=5×580
Calculating the right side:
5×580=2900
Now, the equation becomes:
4(640+y)=2900
Expanding the left side:
2560+4y=2900
Subtract 2560 from both sides:
4y=2900−2560
Calculating the right side:
4y=340
Dividing both sides by 4:
y=3404=85
The number of new urban employees is 85.
Topper's Solved these Questions
Similar Questions
A person sold an article at a loss of 15%. Had he sold it for Rs. 30.60 more, he would have gained 9%. To gain 10%, he should have sold it for:
एक व्यक्ति ने कोई वस्तु 15% हानि पर बेची | यदि उसने इसे 30.60 रुपये अधिक में बेचा होता, तो उसे 9% का लाभ होता | 10% का लाभ कमाने के लिए, उसे इस वस्तु को किस कीमत पर बेचना चाहिए था ?
View SolutionA borrows a sum of Rs 1000 from his friend B on 31 December 2015 on the condition that he will return the same after one year with simple interest at 12%. However, A gets into a position of returning the money on 1 May 2016. How much amount he has to return to B?
A ने अपने मित्र B से 1000 रुपये की राशि 31 दिसंबर 2015 को इस शर्त पर उधार ली कि वह इसे एक वर्ष के बाद 12% साधारण ब्याज के साथ लौटा देगा | हालाँकि, A 1 मई 2016 को ही यह राशि लौटाने की स्थिति में आ गया | वह B को कितनी राशि वापस करेगा ?
View SolutionNumber of employees in a factory decreases in the ratio of 8:7 and salary of employees increase in the ratio of 5:6. Find whether the total salary given to the employees is increased or decreased and in what ratio ?
View Solution42 நபர்கள் பணி செய்யும் ஓர் அலுவலகத்தில் 7 பணியாளர்கள் மகிழுந்து பயன்படுத்துகிறார்கள். 20 பணியாளர்கள் இரு சக்கர வண்டி பயன்படுத்துகிறார்கள் .மீதி 15 பணியாளர்கள் மிதிவண்டி பயன்படுத்துகிறார்கள் . ஒப்பீட்டு நிகழ்வெண் நிகழ்தகவைக் கண்டறிக.
View SolutionTwo-third of the number of employees of a campany are males and the rest are females, if 3/8 of the male employees and 2/5 of female employees are temporary and the total number of permanent employees is 740, then 7/15 of the total number of employees exceeds the number of temporary females by: एक कपनी के कुल का की संख्या में पुरूष कर्मचारियों की संख्या दो-तिहाई है और शेष महिलाएं हैं। यदि पुरूष कर्मचारियों का 3/8 और महिला कर्मचारियों का 2/5 अस्थायी कर्मचारी है तथा स्थायी कर्मचारियों की कल संख्या 740 है, तो कूल कर्मचारियों का 7/15 अस्थायी महिला कर्मचारियों से कितना अधिक है?
View SolutionIn an office, 5/8 of the total of employees are males and the rest are females. 2/5 of the number of males are non-technical workers while 3 of the number of females are technicles workers. What fraction of the total number of employees are technical workers? एक कार्यालय में कुल कमर्चारियों का 5/8 पुरूष है तथा शेष महिलाये हैं पुरूषों का 2/5 नोनटेकनिकल कर्मचारी है जबकि महिलायों का 2/3 तकनीकी कमचारी है तो कुल कमर्चारियों में टैकनिकल कर्मचारियों का कितना हिस्सा है।
View SolutionThe given table shows the number (in percent) of employees working in different depratments of an organization. The table also shows the ratio of males and females and the ratio of employees living in city Z and employees , living in city Y. The total number of employees in the organization 80,000.
दी गई तालिका में एक संगठन के विभिन्न विभागों में कार्य कर रहे कर्मचारियों की संख्या ( प्रतिशत में ) को दर्शाया गया है। यह तालिका पुरूषों तथा महिलाओं के अनुपाव को तथा शहर ,Z में रह रहे कर्मचारियों तथा शहर Y में रह रहे कर्मचारियों के अनुपात को भी दर्शाती है। संगठन रद में कुल कर्मचारियों की संख्या 80000 है।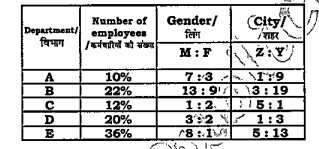 How many employees of department A and C together are living in city Z?
How many employees of department A and C together are living in city Z?
विभाग A तथा C के कितने कर्मचारी, शहर Z में साथ-मिलकर रह रहें है?
View SolutionThe given table shows the number (in percent) of employees working in different depratments of an organization. The table also shows the ratio of males and females and the ratio of employees living in city Z and employees , living in city Y. The total number of employees in the organization 80,000.
दी गई तालिका में एक संगठन के विभिन्न विभागों में कार्य कर रहे कर्मचारियों की संख्या ( प्रतिशत में ) को दर्शाया गया है। यह तालिका पुरूषों तथा महिलाओं के अनुपाव को तथा शहर ,Z में रह रहे कर्मचारियों तथा शहर Y में रह रहे कर्मचारियों के अनुपात को भी दर्शाती है। संगठन रद में कुल कर्मचारियों की संख्या 80000 है।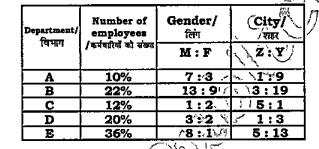 Male employees of department E is what percent of the employees living in city Z form department A?
Male employees of department E is what percent of the employees living in city Z form department A?
विभाग E के पुरूष कर्मचारी, विभाग A से शहर Z में रह रहे कर्मचारियों का कितने प्रतिशत है?
View SolutionThe given table shows the number (in percent) of employees working in different depratments of an organization. The table also shows the ratio of males and females and the ratio of employees living in city Z and employees , living in city Y. The total number of employees in the organization 80,000.
दी गई तालिका में एक संगठन के विभिन्न विभागों में कार्य कर रहे कर्मचारियों की संख्या ( प्रतिशत में ) को दर्शाया गया है। यह तालिका पुरूषों तथा महिलाओं के अनुपाव को तथा शहर ,Z में रह रहे कर्मचारियों तथा शहर Y में रह रहे कर्मचारियों के अनुपात को भी दर्शाती है। संगठन रद में कुल कर्मचारियों की संख्या 80000 है।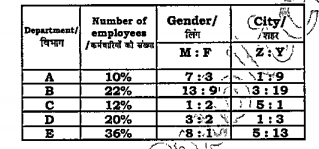 What is the ratio of male employee working in department B and D together to female employee working in department A and E together?
What is the ratio of male employee working in department B and D together to female employee working in department A and E together?
विभाग B तथा D को मिलाकर कार्य कर रहे पुरूष कर्मचारियों का विभाग A तथा E को मिलाकर कार्य कर रही महिला कर्मचारियों से क्या अनुपात है?
View SolutionThe given table shows the number (in percent) of employees working in different depratments of an organization. The table also shows the ratio of males and females and the ratio of employees living in city Z and employees , living in city Y. The total number of employees in the organization 80,000.
दी गई तालिका में एक संगठन के विभिन्न विभागों में कार्य कर रहे कर्मचारियों की संख्या ( प्रतिशत में ) को दर्शाया गया है। यह तालिका पुरूषों तथा महिलाओं के अनुपाव को तथा शहर ,Z में रह रहे कर्मचारियों तथा शहर Y में रह रहे कर्मचारियों के अनुपात को भी दर्शाती है। संगठन रद में कुल कर्मचारियों की संख्या 80000 है।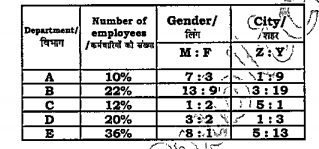 On an average how many residents of city Y are working in each department?
On an average how many residents of city Y are working in each department?
शहर Y के कितने निवासी औसतन एक विभाग में कार्य कर रहे है?
View SolutionThe given table shows the number (in percent) of employees working in different depratments of an organization. The table also shows the ratio of males and females and the ratio of employees living in city Z and employees , living in city Y. The total number of employees in the organization 80,000.
दी गई तालिका में एक संगठन के विभिन्न विभागों में कार्य कर रहे कर्मचारियों की संख्या ( प्रतिशत में ) को दर्शाया गया है। यह तालिका पुरूषों तथा महिलाओं के अनुपाव को तथा शहर ,Z में रह रहे कर्मचारियों तथा शहर Y में रह रहे कर्मचारियों के अनुपात को भी दर्शाती है। संगठन रद में कुल कर्मचारियों की संख्या 80000 है।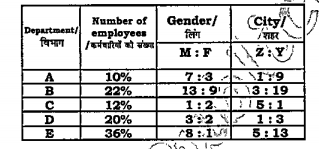 what are the total number of employee in department A and E together?
what are the total number of employee in department A and E together?
विभाग A तथा E में मिलाकर कुल कर्मचारियों की संख्या क्या है?
View SolutionIn a class of 80 students, the ratio of the urban to the rural is 5 : 3. In a test, the average score of the rural students is 40% more than that of the urban students. If the average score of all the students is 69, then what is the average score of the rural students?
80 छात्रों की एक कक्षा में, शहरी और ग्रामीण का अनुपात 5 : 3 है | किसी परीक्षा में, ग्रामीण छात्रों के औसत अंक शहरी छात्रों के औसत अंक से 40% अधिक थे | यदि सभी छात्रों के औसत अंक 69 हैं, तो ग्रामीण छात्रों के औसत अंक ज्ञात करें |
View SolutionTwo numbers are in the ratio 5:7. If the first number is 20, then the second number will be:
दो संख्याएँ 5 : 7 के अनुपात में हैं | यदि पहली संख्या 20 है, तो दूसरी संख्या कितनी है ?
View SolutionThe ratio of the number of rural and urban workers (includes men and women only) in an office is 3 : 2. If 20% of rural and 25% of urban are women, the percentage of men is:
किसी कार्यालय में ग्रामीण और शहरी कर्मचारियों ( केवल पुरुष और महिला शामिल ) की संख्या का अनुपात 3 : 2 है | यदि 20% ग्रामीण तथा 25% शहरी कर्मचारी महिलाएं हैं, तो पुरुषों का प्रतिशत ज्ञात करें |
View SolutionThe Bar graph shown below presents the number of employees in an office during eight consecutive years.
नीचे दिया गया दंड आरेख किसी कार्यालय में आठ लगातार वर्षों के दौरान कर्मचारियों की संख्या को दर्शाता है |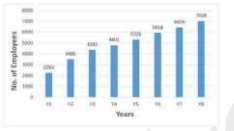
What is the growth percentage in the number of employees from Y5 to Y6?
Y5 से Y6 तक कर्मचारियों की संख्या में वृद्धि का प्रतिशत कितना है ?
View Solution
