A circle touches the side PQ of a △APQ at the point R and sides AP and AQ produced at the points B and C, respectively. If the perimeter of △APQ = 30 cm, then the length of AB is :
एक वृत्त त्रिभुज APQ की भुजा PQ को बिंदु R पर स्पर्श करता है तथा भुजाओं AP और AQ को क्रमश: बिंदु B और C तक बढ़ाया जाता है | यदि त्रिभुज APQ का परिमाप = 30 सेमी है, तो AB की लंबाई ज्ञात करें |
20 cm
10 cm
12 cm
15 cm
To solve the problem step by step, we will analyze the triangle APQ and the circle that touches its sides.
Step 1: Understand the Geometry
We have a triangle APQ with a circle inscribed in it, which touches side PQ at point R. The sides AP and AQ are extended to points B and C, respectively.
Step 2: Use the Property of Tangents
From the properties of tangents drawn from an external point to a circle, we know that:
- PB = PR (tangent from point P)
- QC = QR (tangent from point Q)
- AP = AC (tangent from point A)
Step 3: Set Up the Perimeter Equation
The perimeter of triangle APQ is given as 30 cm. Therefore, we can express the perimeter as:
Perimeter=AP+AQ+PQ=30 cm
Step 4: Express PQ in Terms of Tangents
Since R is the point where the circle touches PQ, we can express PQ as:
PQ=PR+RQ
From the tangent properties, we know:
- PR = PB
- RQ = QC
Thus, we can rewrite the perimeter equation as:
AP+AQ+(PB+QC)=30
Step 5: Substitute Equal Lengths
Since PB = PR and QC = RQ, we can substitute these into the equation:
AP+AQ+PB+QC=30
Now, since AP = AC, we can denote:
- Let AP=AC=x
- Therefore, PB=PR=x and QC=RQ=x
Now, substituting these into the perimeter equation:
x+x+x+x=30
This simplifies to:
4x=30
Step 6: Solve for x
Now, divide both sides by 4:
x=304=7.5 cm
Step 7: Find AB
Since AB = AP + PB, we can express AB as:
AB=AP+PB=x+x=2x
Substituting the value of x:
AB=2×7.5=15 cm
Conclusion
Thus, the length of AB is 15 cm.
We have a triangle APQ with a circle inscribed in it, which touches side PQ at point R. The sides AP and AQ are extended to points B and C, respectively.
From the properties of tangents drawn from an external point to a circle, we know that:
- PB = PR (tangent from point P)
- QC = QR (tangent from point Q)
- AP = AC (tangent from point A)
The perimeter of triangle APQ is given as 30 cm. Therefore, we can express the perimeter as:
Perimeter=AP+AQ+PQ=30 cm
Since R is the point where the circle touches PQ, we can express PQ as:
PQ=PR+RQ
From the tangent properties, we know:
- PR = PB
- RQ = QC
AP+AQ+(PB+QC)=30
Since PB = PR and QC = RQ, we can substitute these into the equation:
AP+AQ+PB+QC=30
Now, since AP = AC, we can denote:
- Let AP=AC=x
- Therefore, PB=PR=x and QC=RQ=x
x+x+x+x=30
This simplifies to:
4x=30
Now, divide both sides by 4:
x=304=7.5 cm
Since AB = AP + PB, we can express AB as:
AB=AP+PB=x+x=2x
Substituting the value of x:
AB=2×7.5=15 cm
Thus, the length of AB is 15 cm.
Topper's Solved these Questions
Similar Questions
A circle is touching the side BC of a △ABC at point P and touching AB and AC produced at Q and R respectively. Prove that AQ=12(perimeter of △ABC).
View SolutionA circle is inscribed in △ABC, touching AB, BC and AC at points P, Q and R respectively. If AB-BC = 4cm, AB-AC = 2cm and the perimeter of △ABC=32cm, then PB+AR is equal to :
/ एक वृत्त त्रिभुज ABC के भीतर स्थित है जो AB, BC और AC को क्रमशः बिंदु P, Q और R पर स्पर्श करता है | यदि AB-BC =4 सेमी, AB-AC =2 सेमी और त्रिभुज ABC का परिमाप = 32 सेमी है, तो PB+AR का मान ज्ञात करें
View Solution
Knowledge Check
A circle touches the side BC of a ΔABC at P and also touches AB and AC produced at Q and R, respectively. If the perimeter of ΔABC = 26.4 cm, then the length of AQ is:
A8.8 cmB13.2 cmC15.4 cmD17.6 cmA circle is inscribed in a triangle ABC. It touches the sides AB, BC and AC at the points R, P and Q respectively. If AQ = 4.5 cm, PC = 5.5 cm and BR = 6 cm, then the perimeter of the triangle ABC is:
एक वृत्त किसी त्रिभुज ABC में अन्तर्निहित है | यह भुजाओं AB, BC और AC को क्रमशः बिंदु R, P और Q पर स्पर्श करता है | यदि AQ = 4.5 सेमी, PC = 5.5 सेमी और BR = 6 सेमी है, तो त्रिभुज ABC का परिमाप ज्ञात करें |A30.5cm.B28cm.C32cm.D26.5cm.
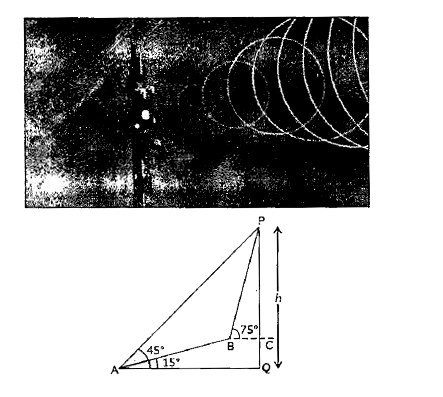
In the right angled triangle APQ, the length AP is :
View SolutionA circle touches the side BC of △ABC at D and AB and AC are produced to E and F, respectively. If AB = 10 cm, AC=8.6 cm and BC=6.4 cm, then BE = ?
एक वृत्त त्रिभुज ABC की भुजा BC को D पर स्पर्श करता है और AB तथा AC को क्रमशः E और F तक बढ़ाया जाता है | यदि AB = 10 सेमी, AC= 8.6 सेमी और BC=6.4 सेमी है, तो BE =?
View SolutionA circle is inscribed in quadrilateral touching sides AB , BC , CD and AD at the points P , Q , R and S respectively. If BP = 4cm, SD = 6cm and BC = 7cm, then the length of DC is:
एक वृत्त किसी चतुर्भुज के भीतर स्थित है जो भुजाओं AB, BC, CD और AD को क्रमशः P, Q, R और S पर स्पर्श करता है | यदि BP = 4 सेमी, SD = 6 सेमी और BC = 7 सेमी है, तो DC की लंबाई ज्ञात करें |
View SolutionP and Q are the points on sides AB and AC respectively of a ΔABC , such that PQ||BC .If AP∣PB=2:3 and AQ=4 cm , then the length of AC is ….. Cm .
View SolutionA circle is inscribed in a triangle ABC. It touches the sides AB, BC and AC at the points R, P and Q respectively. If AQ = 4.5 cm, PC = 5.5 cm and BR = 6 cm, then the perimeter of the triangle ABC is:
एक वृत्त किसी त्रिभुज ABC में अन्तर्निहित है | यह भुजाओं AB, BC और AC को क्रमशः बिंदु R, P और Q पर स्पर्श करता है | यदि AQ = 4.5 सेमी, PC = 5.5 सेमी और BR = 6 सेमी है, तो त्रिभुज ABC का परिमाप ज्ञात करें |
View SolutionA circle is inscribed in a triangle ABC. It touches sides AB, BC and AC at the points P,Q and R respectively. If BP = 6.5 cm, CQ = 4.5 cm and AR = 5.5 cm, then the perimeter (in cm) of the triangle ABC is :
एक वृत्त किसी त्रिभुज ABC के भीतर स्थित है | यह भुजाओं AB, BC तथा AC को क्रमशः बिंदु P, Q और R पर स्पर्श करता है | यदि BP = 6.5 सेमी, CQ = 4.5 सेमी और AR = 5.5 सेमी है, तो त्रिभुज ABC का परिमाप ( सेमी में ) क्या होगा ?
View Solution
