Chords AB and CD of a circle intersect externally at P. If AB = 6 cm, CD = 3 cm and PB = 4 cm, then the length (in cm) of PD is :
एक वृत्त की जीवायें AB और CD एक दूसरे को बाहर से P पर काटती हैं | यदि AB = 6 सेमी,CD =3 सेमी और PB =4 सेमी है, तो PD की लंबाई (सेमी में ) ज्ञात करें |
5
6
2
7
To find the length of PD, we can use the property of intersecting chords in a circle. The property states that if two chords AB and CD intersect at an external point P, then the following relationship holds:
PB×PA=PD×PC
Where:
- PB is the length from point P to point B,
- PA is the length from point P to point A,
- PD is the length from point P to point D,
- PC is the length from point P to point C.
Step 1: Identify the lengths given in the problem.
- AB=6cm
- CD=3cm
- PB=4cm
From the length of chord AB, we can find PA:
PA=AB−PB=6cm−4cm=2cm
Step 2: Calculate PA and PC.
Since CD=3cm, we can express PC in terms of PD:
PC=CD+PD=3cm+PD
Step 3: Substitute the values into the intersecting chords formula.
Now we can substitute PB, PA, and PC into the equation:
PB×PA=PD×PC
4×10=PD×(3+PD)
Step 4: Simplify the equation.
Calculating the left side:
4×10=40
So, we have:
40=PD×(3+PD)
Step 5: Rearrange the equation.
This leads us to:
PD2+3PD−40=0
Step 6: Factor the quadratic equation.
Now we can factor the quadratic equation:
(PD+8)(PD−5)=0
Step 7: Solve for PD.
Setting each factor to zero gives us:
1. PD+8=0 → PD=−8 (not valid since length cannot be negative)
2. PD−5=0 → PD=5
Thus, the length of PD is:
PD=5cm
Final Answer:
The length of PD is 5 cm.
---
- PB is the length from point P to point B,
- PA is the length from point P to point A,
- PD is the length from point P to point D,
- PC is the length from point P to point C.
- AB=6cm
- CD=3cm
- PB=4cm
PA=AB−PB=6cm−4cm=2cm
Since CD=3cm, we can express PC in terms of PD:
PC=CD+PD=3cm+PD
Now we can substitute PB, PA, and PC into the equation:
PB×PA=PD×PC
4×10=PD×(3+PD)
Calculating the left side:
4×10=40
So, we have:
40=PD×(3+PD)
This leads us to:
PD2+3PD−40=0
Now we can factor the quadratic equation:
(PD+8)(PD−5)=0
Setting each factor to zero gives us:
1. PD+8=0 → PD=−8 (not valid since length cannot be negative)
2. PD−5=0 → PD=5
PD=5cm
The length of PD is 5 cm.
Topper's Solved these Questions
Similar Questions
In the given figure,chords AB and CD of a circle intersect externally at P. AB=4 cm,CD=11 cm and PD=15 cm,then the length of PB is:
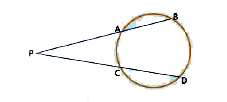
View SolutionThe chords AB and CD of a circle intersect at E. If AE = 12cm, BE = 20.25 cm and CE = 3DE, then the length (in cm) of CE is:
एक वृत्त की दो जीवाएँ AB तथा CD एक-दूसरे को E पर काटती हैं | यदि AE = 12 सेमी, BE =20.25 सेमी तथा CE = 3DE है, तो CE की लंबाई ( सेमी में ) कितनी होगी ?
View Solution
Knowledge Check
Chords AB and CD of a circle intersect externally at P. If AB=6cm,CD=3cmandPD=5cm, then the length of PB is
A5 cmB7.35 cmC6 cmD4 cmChords AB and CD of a circle intersect at a point P inside the circle. If AB = 10 cm, AP = 4 cm and PC = 5 cm, then CD is equal to:
A4.8 cmB6.8 cmC9.8 cmD7.8 cmThe two chords AB and CD of a circle intersect at point P, such that BP=4 cm, PD=5 cm, and CP=8 cm. find the length of chord AB.
A8B10C12D14
In the figure, chords AB and CD of a circle intersect externally at P. If AB = 4cm, CD =11cm and PD=15cm, then the length of PB is:
दी गयी आकृति में, जीवा AB और CD एक वृत्त को बाहरी रूप से P पर काटते हैं। यदि AB = 4cm, CD =11 cm और PD = 15cm है, तो PB की लंबाई ज्ञात करे |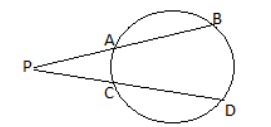
View SolutionTwo chords AB and CD of a circle intersect at a point P inside the circle. If AB = 7cm , PC = 2 cm and AP = 4 cm, then CD is equal to:
एक वृत्त की दो जीवाएं AB और CD वृत्त के भीतर बिंदु P पर एक दूसरे को काटती हैं | यदि AB = 7 सेमी, PC = 2 सेमी और AP = 4 सेमी है, तो CD किसके बराबर होगा ?
View SolutionChords AB and CD of a circle intersect at a point P inside the circle. If AB = 10 cm. AP = 4 cm and PC = 5 cm, then CD is equal to:
एक वृत्त की जीवायें AB और CD वृत्त के भीतर स्थित बिंदु P पर एक दूसरे को काटती हैं | यदि AB =10 सेमी,AP = 4 सेमी और PC = 5 सेमी है, तो CD का मान क्या होगा ?
View SolutionIn a circle, AB and DC are two chords. When AB and DC are produced, they meet at P. If PC = 5.6 cm, PB = 6.3 cm and AB = 7.7 cm, the the length of CD is :
/ एक वृत्त में, AB तथा DC दो जीवाएं है | जब AB और DC को बढ़ाया जाता है, तो वे P पर मिलती हैं | यदि PC = 5.6 सेमी , PB = 6.3 सेमी और AB = 7.7 सेमी है, तो CD की लंबाई ज्ञात करें |
View Solutionin a circle, chords AB and CD intersect each other at E. If CD = 18 cm, DE = 6 cm and AE = 18 cm, then BE = ?
एक वृत्त में, जीवा AB और CD एक दूसरे को E पर काटते हैं| यदि CD = 18 सेमी, DE = 6 सेमी और AE = 18 सेमी है, तो BE =?
View Solution