in a circle, chords AB and CD intersect each other at E. If CD = 18 cm, DE = 6 cm and AE = 18 cm, then BE = ?
एक वृत्त में, जीवा AB और CD एक दूसरे को E पर काटते हैं| यदि CD = 18 सेमी, DE = 6 सेमी और AE = 18 सेमी है, तो BE =?
6 cm
8 cm
3 cm
4 cm
To solve the problem, we will use the property of intersecting chords in a circle. According to this property, if two chords intersect each other, then the products of the lengths of the segments of each chord are equal.
1. Identify the lengths given in the problem:
- CD = 18 cm
- DE = 6 cm
- AE = 18 cm
- Let BE = x (this is what we need to find).
2. Calculate CE:
Since CD = 18 cm and DE = 6 cm, we can find CE:
CE=CD−DE=18cm−6cm=12cm
3. Set up the equation using the intersecting chords property:
According to the property, we have:
AE×BE=CE×DE
Substituting the known values:
18×x=12×6
4. Calculate the right side of the equation:
12×6=72
So the equation becomes:
18x=72
5. Solve for x:
To find x, divide both sides by 18:
x=7218=4
6. Conclusion:
Therefore, BE = 4 cm.
Final Answer:
BE = 4 cm.
- CD = 18 cm
- DE = 6 cm
- AE = 18 cm
- Let BE = x (this is what we need to find).
Since CD = 18 cm and DE = 6 cm, we can find CE:
CE=CD−DE=18cm−6cm=12cm
According to the property, we have:
AE×BE=CE×DE
Substituting the known values:
18×x=12×6
12×6=72
So the equation becomes:
18x=72
To find x, divide both sides by 18:
x=7218=4
Therefore, BE = 4 cm.
BE = 4 cm.
Topper's Solved these Questions
Similar Questions
in a circle, chords AB and CD intersect each other at E. If CD = 18 cm, DE = 6 cm and AE = 18 cm, then BE = ?
एक वृत्त में, जीवा AB और CD एक दूसरे को E पर काटते हैं| यदि CD = 18 सेमी, DE = 6 सेमी और AE = 18 सेमी है, तो BE =?
View SolutionChords AB and CD of a circle intersect externally at P. If AB = 6 cm, CD = 3 cm and PB = 4 cm, then the length (in cm) of PD is :
एक वृत्त की जीवायें AB और CD एक दूसरे को बाहर से P पर काटती हैं | यदि AB = 6 सेमी,CD =3 सेमी और PB =4 सेमी है, तो PD की लंबाई (सेमी में ) ज्ञात करें |
View Solution
Knowledge Check
Chords AB and CD of a circle intersect externally at P. If AB=6cm,CD=3cmandPD=5cm, then the length of PB is
A5 cmB7.35 cmC6 cmD4 cmChords AB and CD of a circle Intersect at E. If AE = 9 cm, BE = 12 cm and CE = 3DE, then the length of DE (in cm) is
A94B4C6D7
The chords AB and CD of a circle intersect at E. If AE = 12cm, BE = 20.25 cm and CE = 3DE, then the length (in cm) of CE is:
एक वृत्त की दो जीवाएँ AB तथा CD एक-दूसरे को E पर काटती हैं | यदि AE = 12 सेमी, BE =20.25 सेमी तथा CE = 3DE है, तो CE की लंबाई ( सेमी में ) कितनी होगी ?
View SolutionTwo chords AB and CD of a circle intersect at a point P inside the circle. If AB = 7cm , PC = 2 cm and AP = 4 cm, then CD is equal to:
एक वृत्त की दो जीवाएं AB और CD वृत्त के भीतर बिंदु P पर एक दूसरे को काटती हैं | यदि AB = 7 सेमी, PC = 2 सेमी और AP = 4 सेमी है, तो CD किसके बराबर होगा ?
View SolutionChords AB and CD of a circle intersect at a point P inside the circle. If AB = 10 cm. AP = 4 cm and PC = 5 cm, then CD is equal to:
एक वृत्त की जीवायें AB और CD वृत्त के भीतर स्थित बिंदु P पर एक दूसरे को काटती हैं | यदि AB =10 सेमी,AP = 4 सेमी और PC = 5 सेमी है, तो CD का मान क्या होगा ?
View SolutionIn the given figure, if AB = 10 cm, CD = 7 cm, SD = 4 cm and AS = 5 cm, then BC = ?

View SolutionIn the figure, chords AB and CD of a circle intersect externally at P. If AB = 4cm, CD =11cm and PD=15cm, then the length of PB is:
दी गयी आकृति में, जीवा AB और CD एक वृत्त को बाहरी रूप से P पर काटते हैं। यदि AB = 4cm, CD =11 cm और PD = 15cm है, तो PB की लंबाई ज्ञात करे |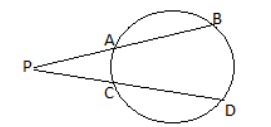
View SolutionChords AB and CD of a circle , when produced, meet at point P outside the circle. If AB = 6 cm, CD = 3 cm and PD = 5 cm, then PB is equal to :
एक वृत्त की जीवाएं AB और CD को जब बढ़ाया जाता है तो वे वृत्त के बाहर बिंदु P पर मिलती हैं | यदि AB = 6 सेमी, CD = 3 सेमी और PD = 5 सेमी है, तो PB का मान क्या होगा ?
View Solution
