Question

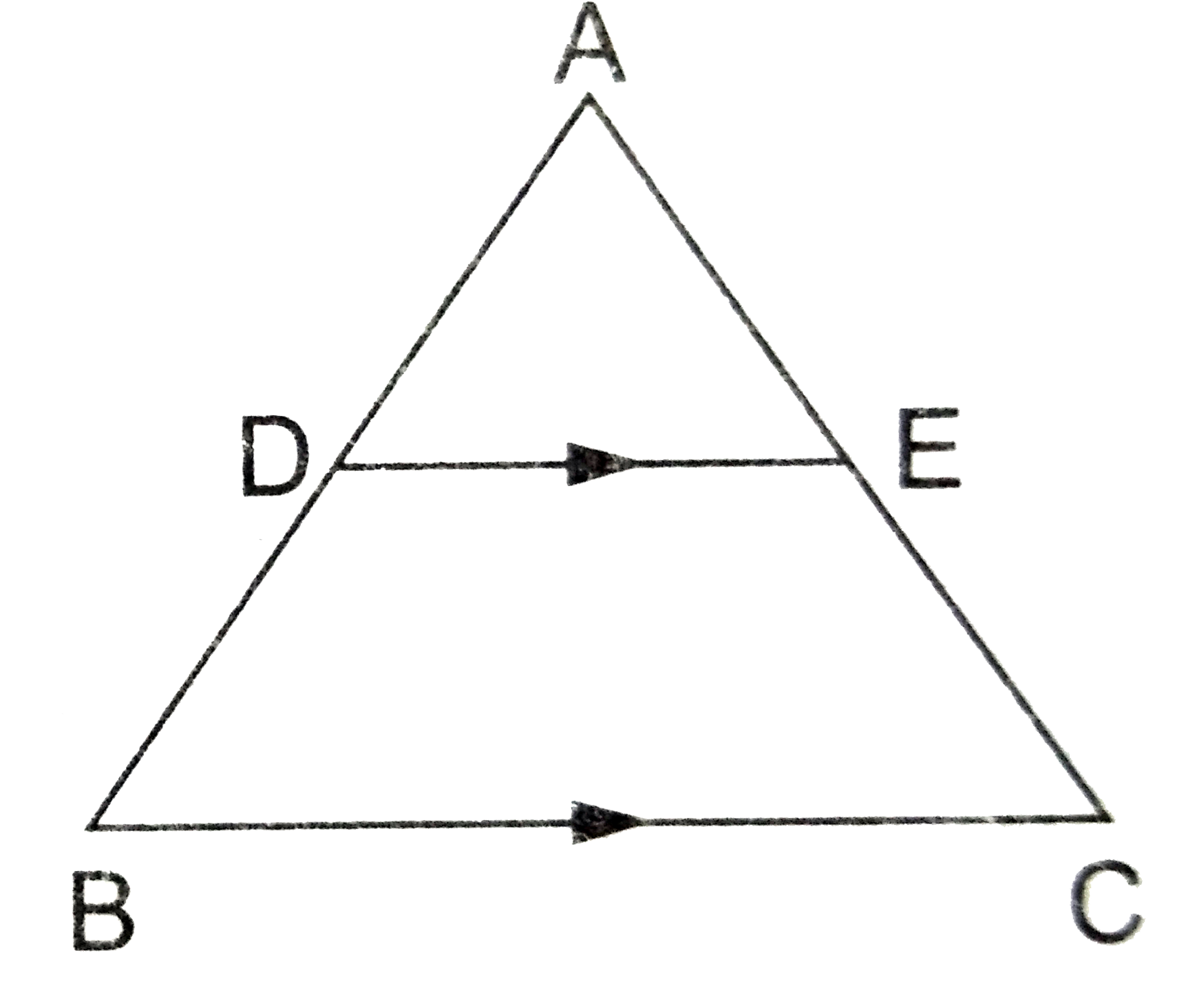
In a ${\displaystyle △ABC}$, DE is parallel to BC, AD = 3 cm, AE = 4cm and AC = 10 cm, then the value of BD in centimetres, is:

${\displaystyle △ABC}$ में, DE, BC & समानांतर है, AD = 3 cm, AE= 4cm और AC = 10 cm, फिर BD (सेंटीमीटर में) मान है:

Options

A. 3.5
B. 7.5
C. 4.5
D. 5.5

Explanation

To solve the problem, we will use the properties of similar triangles since DE is parallel to BC. Here are the steps:

1. Identify the Given Information:
- AD = 3 cm
- AE = 4 cm
- AC = 10 cm
- DE is parallel to BC.

2. Calculate EC:
Since AC = AE + EC, we can find EC:
\( EC = AC - AE = 10 \text{ cm} - 4 \text{ cm} = 6 \text{ cm} \)

3. Use the Basic Proportionality Theorem:
According to the theorem, if a line is drawn parallel to one side of a triangle, it divides the other two sides proportionally. Therefore, we have:
\( \frac{AD}{DB} = \frac{AE}{EC} \)

4. Substitute the Known Values:
Let DB = x. Then we can substitute the known values into the proportion:
\( \frac{3}{x} = \frac{4}{6} \)

5. Cross-Multiply to Solve for x:
Cross-multiplying gives:
\( 3 \cdot 6 = 4 \cdot x \)
\( 18 = 4x \)

6. Solve for x:
Divide both sides by 4:
\( x = \frac{18}{4} = 4.5 \text{ cm} \)

7. Conclusion:
Therefore, the value of BD is:
\( BD = 4.5 \text{ cm} \)

Final Answer:
BD = 4.5 cm