In a circle , O is the centre of the circle. Chords AB and CD intersect at P. If ∠AOD=32∘ and ∠COB=26∘, then the measure of ∠APD lies between:
केंद्र O के साथ एक वृत्त में, जीवा AB और CD,P पर प्रतिच्छेद करते हैं, यदि ∠AOD=32∘ और ∠COB=26∘ तो ∠APD का माप किसके बीच में होगा
26∘ and 30∘
30∘ and 34∘
22∘ and 26∘
18∘ and 22∘
To solve the problem, we need to find the measure of angle APD given that angle AOD = 32° and angle COB = 26°. We'll use the properties of angles in a circle, specifically the relationship between central angles and inscribed angles.
1. Identify the Angles:
- We are given:
- Angle AOD = 32°
- Angle COB = 26°
2. Use the Central Angle Property:
- The angle AOD is a central angle that subtends the arc AD. The inscribed angle APD that subtends the same arc AD will be half of the central angle AOD.
- Therefore, angle APD = 1/2 * angle AOD
- So, angle APD = 1/2 * 32° = 16°.
3. Calculate the Measure of Angle BOC:
- Similarly, angle COB is a central angle that subtends the arc CB. The inscribed angle BPC that subtends the same arc CB will be half of the central angle COB.
- Therefore, angle BPC = 1/2 * angle COB
- So, angle BPC = 1/2 * 26° = 13°.
4. Determine the Measure of Angle APD:
- Now, we know that angle APD and angle BPC are angles that are formed by the intersection of the chords AB and CD at point P.
- The angles APD and BPC are related as follows:
- Angle APD + Angle BPC = 180° (since they are supplementary angles).
- Therefore, angle APD = 180° - angle BPC = 180° - 13° = 167°.
5. Final Measure of Angle APD:
- Since angle APD is formed by the intersection of the two chords, we can conclude that angle APD lies between 16° and 167°.
Conclusion:
The measure of angle APD lies between 16° and 167°.
- We are given:
- Angle AOD = 32°
- Angle COB = 26°
- The angle AOD is a central angle that subtends the arc AD. The inscribed angle APD that subtends the same arc AD will be half of the central angle AOD.
- Therefore, angle APD = 1/2 * angle AOD
- So, angle APD = 1/2 * 32° = 16°.
- Similarly, angle COB is a central angle that subtends the arc CB. The inscribed angle BPC that subtends the same arc CB will be half of the central angle COB.
- Therefore, angle BPC = 1/2 * angle COB
- So, angle BPC = 1/2 * 26° = 13°.
- Now, we know that angle APD and angle BPC are angles that are formed by the intersection of the chords AB and CD at point P.
- The angles APD and BPC are related as follows:
- Angle APD + Angle BPC = 180° (since they are supplementary angles).
- Therefore, angle APD = 180° - angle BPC = 180° - 13° = 167°.
- Since angle APD is formed by the intersection of the two chords, we can conclude that angle APD lies between 16° and 167°.
The measure of angle APD lies between 16° and 167°.
Topper's Solved these Questions
Similar Questions
In the following figure, O is the centre of the circle. AB and CD are equal chords. If ∠AOB=100∘ , Find the ∠CED.

View SolutionTwo chords AB and CD of a circle with centre O intersect each other at P If ∠APC=95∘ and ∠AOD=110∘, then what ∠BOC is:
केंद्र O वाले एक वृत्त की दो जीवाएँ AB तथा CD हैं जो एक-दूसरे को P पर प्रतिच्छेद करती हैं | यदि ∠APC=95∘ तथा ∠AOD=110∘है ,तो ∠BOC का मान क्या होगा ?
View Solution
Knowledge Check
If 76 is divided into four parts proportional to 7, 5, 3, 4, then the smallest part is:
AA) 12BB) 15CB) 15DD) 19The incomes of two persons A and B are in the ratio 3 : 4. If each saves Rs.100 per month, the ratio of their expenditures is Rs. 1 : 2. Find their incomes.
AA) Rs. 100 and Rs.150BB) Rs. 150 and Rs.200CC) Rs.200 and Rs.250DD) Rs.250 and Rs.300Alloy A contains 40% gold and 60% silver. Alloy B contains 35% gold and 40% silver and 25% copper. Alloys A and B are mixed in the ratio of 1:4 .What is the ratio of gold and silver in the newly formed alloy is?
AA) 20% and 30%BB) 36% and 44%CC) 25% and 35%DD) 49% and 36%
In a circle, chords AD and BC meet at a point E outside the circle. If ∠BAE=76∘ and ∠ADC=102∘, then ∠AEC is equal to:
एक वृत्त में, जीवाएं AD और BC वृत्त के बाहर स्थित बिंदु E पर मिलती हैं | यदि ∠BAE=76∘ और ∠ADC=102∘है, तो ∠AEC किसके बराबर है?
View SolutionIn a circle with centre O, AB is the diameter, P and Q are two points on the circle on the same side of the diameter AB, AQ and BP intersect at C. If ∠POQ=54∘, then the measure of ∠PCA is:
केंद्र O के साथ एक वृत्त में, AB व्यास है, P और Q, व्यास AB के एक तरफ वृत्त पर दो बिंदु है AQ और BP, C पर परिछेदित करती है। यदि ∠POQ=54∘ है, तो ∠PCA का माप है
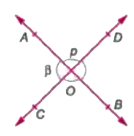
View SolutionIn the given figure, straight lines AB and CD intersect at O.If ∠β = 3∠p,then ∠p is equal to
View SolutionIn a circle with centre O, chords AB and CD intersect inside the circumference at E. Find ∠AEC. If ∠AOC=40∘and∠BOD=50∘.
View Solution
In the above figure, O is the centre of the circle and AB=CD. If ∠APB=110∘, then find the angle made by the chord CD at the centre.
View Solution
