Question

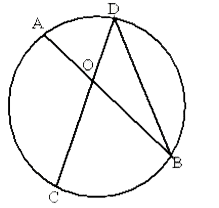
In a circle with centre O, AB is the diameter, P and Q are two points on the circle on the same side of the diameter AB, AQ and BP intersect at C. If ${\displaystyle \angle POQ={54}^{\circ }}$, then the measure of ${\displaystyle \angle PCA}$ is:
केंद्र O के साथ एक वृत्त में, AB व्यास है, P और Q, व्यास AB के एक तरफ वृत्त पर दो बिंदु है AQ और BP, C पर परिछेदित करती है। यदि
${\displaystyle \angle POQ={54}^{\circ }}$ है, तो ${\displaystyle \angle PCA}$ का माप है

Options

A. `54^@`
B. `63^@`
C. `72^@`
D. `56^@`

Explanation

To find the measure of angle PCA in the given circle with center O, where AB is the diameter and points P and Q lie on the circle, we can follow these steps:

1. Draw the Circle: Start by drawing a circle with center O. Mark the diameter AB.

2. Locate Points P and Q: Place points P and Q on the circumference of the circle on the same side of the diameter AB.

3. Identify Intersection Point C: Draw lines AQ and BP such that they intersect at point C.

4. Given Angle: We know that angle POQ = 54°.

5. Use the Central Angle Theorem: According to the Central Angle Theorem, the angle subtended at the center (angle POQ) is twice the angle subtended at any point on the circumference by the same arc. Therefore, angle PQA (which subtends the same arc PQ) will be:
\( \text{Angle PQA} = \frac{1}{2} \times \text{Angle POQ} = \frac{1}{2} \times 54° = 27° \)

6. Angle in a Semicircle: Since AB is the diameter, angle APB is a right angle (90°) because any angle subtended by a diameter in a circle is a right angle.

7. Calculate Angle PCA: Now we can find angle PCA using the fact that the sum of angles in triangle APC is 180°:
\( \text{Angle PCA} + \text{Angle PQA} + \text{Angle APB} = 180° \)
Substituting the known values:
\( \text{Angle PCA} + 27° + 90° = 180° \)
\( \text{Angle PCA} + 117° = 180° \)
\( \text{Angle PCA} = 180° - 117° = 63° \)

Final Answer:
The measure of angle PCA is 63°.