From a point P on a level ground, the angle of elevation of the top of a tower is 30∘ . If the tower is 270 m high, the distance
of point P from the foot of the tower is:
भूमि पर स्थित किसी बिंदु P से एक मीनार के शीर्ष का उन्नयन कोण 30∘ है | यदि यह मीनार 270 मीटर ऊँची है, तो मीनार के तल से बिंदु P की दूरी ज्ञात करें |
467.65m
476.65m
376.65m
367.65m
To solve the problem, we will use trigonometric ratios in a right triangle formed by the tower, the point on the ground, and the line of sight from the point to the top of the tower.
1. Understand the Setup:
- Let point A be the top of the tower, point B be the foot of the tower, and point P be the point on the ground from which the angle of elevation is measured.
- The height of the tower (AB) is given as 270 m.
- The angle of elevation from point P to point A is 30 degrees.
2. Identify the Triangle:
- We have a right triangle PAB where:
- AB = height of the tower = 270 m (perpendicular)
- PB = distance from point P to the foot of the tower (base)
- Angle ∠APB = 30 degrees
3. Use the Tangent Function:
- In triangle PAB, the tangent of the angle of elevation (30 degrees) is given by the formula:
tan(θ)=oppositeadjacent
- Here, the opposite side is AB (height of the tower) and the adjacent side is PB (distance from P to the foot of the tower).
- Therefore, we can write:
tan(30∘)=ABPB=270PB
4. Find the Value of Tan(30 degrees):
- The value of tan(30∘) is 1√3.
5. Set Up the Equation:
- Substitute the value of tan(30∘) into the equation:
1√3=270PB
6. Cross Multiply to Solve for PB:
- Cross multiplying gives:
PB=270⋅√3
7. Calculate the Distance:
- Therefore, the distance of point P from the foot of the tower is:
PB=270√3 meters
Final Answer:
The distance of point P from the foot of the tower is 270√3 meters.
---
- Let point A be the top of the tower, point B be the foot of the tower, and point P be the point on the ground from which the angle of elevation is measured.
- The height of the tower (AB) is given as 270 m.
- The angle of elevation from point P to point A is 30 degrees.
- We have a right triangle PAB where:
- AB = height of the tower = 270 m (perpendicular)
- PB = distance from point P to the foot of the tower (base)
- Angle ∠APB = 30 degrees
- In triangle PAB, the tangent of the angle of elevation (30 degrees) is given by the formula:
tan(θ)=oppositeadjacent
- Here, the opposite side is AB (height of the tower) and the adjacent side is PB (distance from P to the foot of the tower).
- Therefore, we can write:
tan(30∘)=ABPB=270PB
- The value of tan(30∘) is 1√3.
- Substitute the value of tan(30∘) into the equation:
1√3=270PB
- Cross multiplying gives:
PB=270⋅√3
- Therefore, the distance of point P from the foot of the tower is:
PB=270√3 meters
The distance of point P from the foot of the tower is 270√3 meters.
Topper's Solved these Questions
Similar Questions
From a point P on a level ground, the angle of elevation of the top of a tower is 30o . If the tower is 100 m high, the distance of point P from the foot of the tower is (a) 149 m (b) 156 m (c) 173 m (d) 200 m
View SolutionIn figure, the angle of elevation of the top of a tower AC from a point B on the ground is 60∘. If the height of the tower is 20m , find the distance of the point from the foot of the tower.
View Solution
Knowledge Check
From a point P on a level ground the angle of elevation to the top of the tower is 30∘ .If the tower is 100 metre high , the distance of point P from the foot of the tower is (Take √3=1.73)
A149 metreB156 metreC173 metreD188 metreFrom a point 20 m away from the foot of a tower, the angle of elevation of the top of the tower is 30∘ . The height of the tower is
A10√3mB20√3 mC10√3 mD20√3mAt a point 20 m away from the foot of a tower, the angle of elevation of the top of the tower is 30∘ The height of the tower is
A20√3mB20√3mC√320mDNone of these
The angle of elevation of the top of a tower from a point on the ground, which is 25 m away from the foot of between the ship and tower.
View SolutionIn the figure-2, the angle of elevation of the top of tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m. find the distance of the point from the foot of the tower.
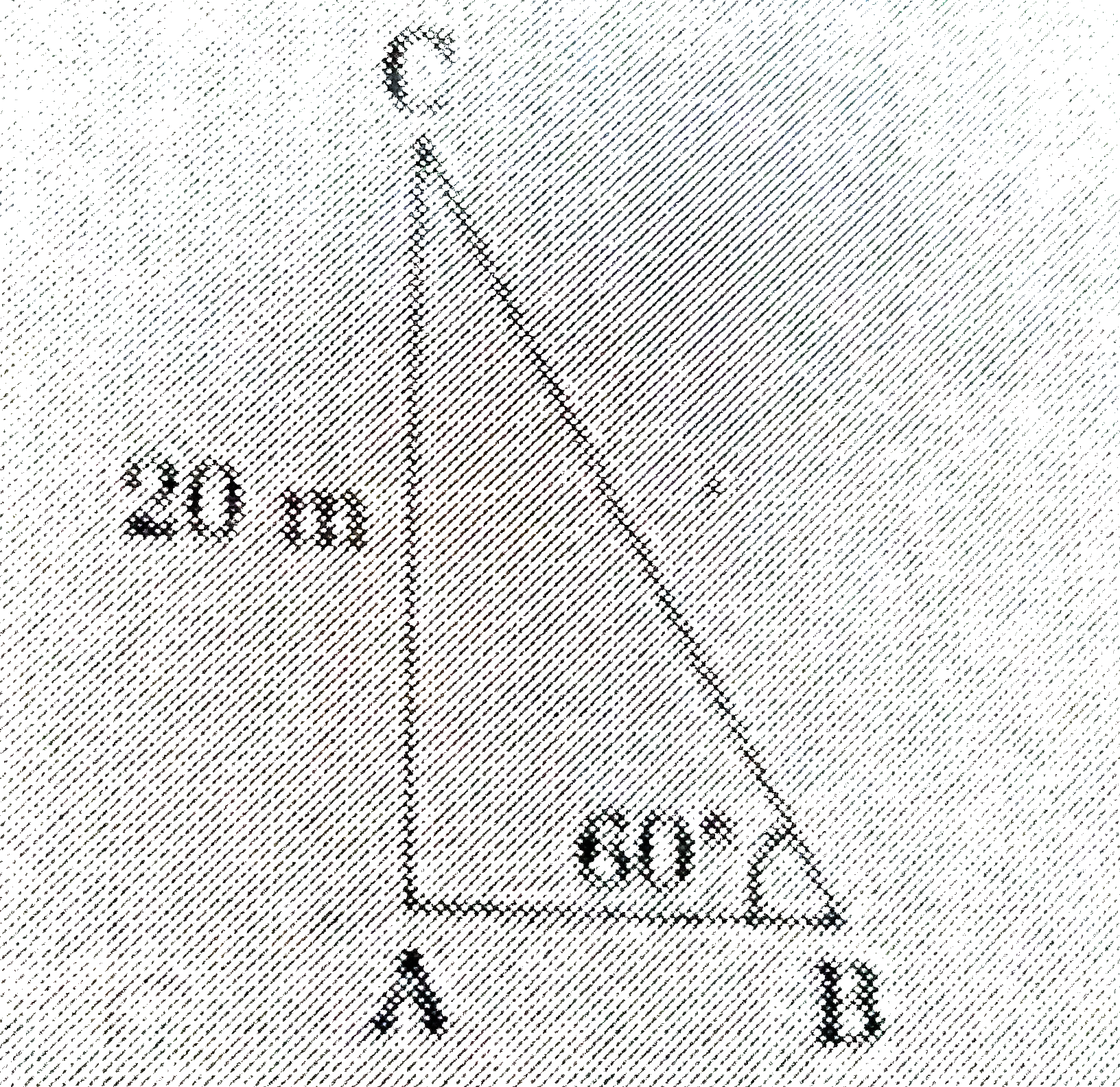
View SolutionThe angle of elevation of the top of a tower at a point on the ground, 50 m away from the foot of the tower, is 60°. Find the height of the tower.
View SolutionOn the level ground, the angle of elevation of a tower is 30∘. On moving 20 m nearer, the angle of elevation is 60∘. The height of the tower is
View SolutionAt a point 20 m away from the foot of a tower, the angle of elevation of the top of the tower is 30∘ The height of the tower is
View Solution
