Ship A is moving with velocity 30m/s East and ship B with velocity 40m/s due North, Initial separation between the ships is 10 km as shown in figure. After what time the ships are closest to each other?
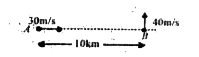
80sec
120sec
160sc
None of these
The correct Answer is:B
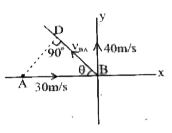
¯vBA=¯vB−¯va=40ˆj−30ˆi
tanθ=4030,|vBA|=50m/s Required time =BDvBA=ABcosθvBA=104×3550=120sec
Similar Questions
Ship A is moving with a velocity 40m/s due north and ship B is moving with a velocity 30m/s due west as shown in the figure. After what time the ships will be closest to each other, if the initial separation between them is 15km ?
View SolutionShip A is moving towards south with speed 3m/s and ship B is travelling due west with speed 4m/s Find the velocity of ship B with respect to ship A
View Solution
Knowledge Check
Ship A is travelling with a velocity of 5kmh−1 due east. A second ship is heading 30∘ east of north. What should be the speed of second ship if it is to remain always due north with respect to the first ship ?
A10kmh−1B9kmh−1C8kmh−1D7kmh−1A ship is moving due east with a velocity of 12m/sec, a truck is moving across on the ship with velocity 4m/sec.A monkey is climbing the vertical pole mounted on the truck with a velocity of 3m/sec.Find the velocity of the monkey as observed by the man on the shore (m/sec)
A10B15C13D20A ship A is moving westwards with a speed of 10kmh−1 and a ship B 100 km south of A, is moving northwards with a speed of 10kmh−1 The time after which the distance between them becomes shortest, is
A0 hB5 hC5√2hD10√2h
Ship A is travelling with a velocity of 5kmh−1 due east. A second ship is heading 30∘ east of north. What should be the speed of second ship if it is to remain always due north with respect to the first ship ?
View SolutionA ship is moving due east with a velocity of 12 m/ sec, a truck is moving across on the ship with velocity 4 m/sec. A monkey is climbing the vertical pole mounted on the truck with a velocity of 3m/sec. Find the velocity of the monkey as observed by the man on the shore
View SolutionTwo ships A and B are 4 km apart. A is due west of B. If A moves with a uniform velocity of 8 km hr−1 due east and B moves with a uniform velocity of 6 km hr−1 due south, calculate (i) the magnitude of velocity of A relative to B, (ii) the closest distance apart of A and B.
View SolutionShip A is located 4.0 km north and 2.5 km east of ship B. Ship A has a velocity of 22 km/h toward the south, and ship B has a velocity of 40 km/h in a direction 37∘ north of east. (a) What is the velocity of A relative to B in unit-vector notation with toward the east? (b) Write an expression (in terms of ˆiandˆj) for the position of A relative to B as a function of t, where t = 0 when the ships are in the positions described above. ( c) At what time is the separation between the ships least? (d) What is that least separation?
View SolutionA ship moves along the equator to the east with velocity v0=30km/hour. The southeastern wind blows at an angle φ=60∘ to the equator with velocity v=15km/hour. Find the wind velocity v′ relative to the ship and the angle of φ′ between the equator and the wind direction in the reference frame fixed to the ship.
View Solution
