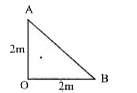
Three identical spheres, each of mass m are placed at the corners of a right angled triangle with mutually perpendicular sides equal to 2m. Taking their point of intersection as the origin, the position vector of centre of mass is
13(ˆi−ˆj)
23(ˆi−ˆj)
23(ˆi+ˆj)
13(ˆi+ˆj)
The correct Answer is:C
xCM=∑mixi∑mi=m×0+m×0+m×2m+m+m=23
yCM=∑miyi∑mi=m×2+m×0+m×0m+m+m=23∴ Position vector of centre mass is 23(ˆi+ˆj)
Similar Questions
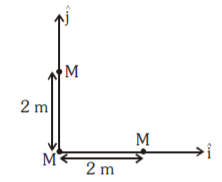
Three identical spheres , each of mass M , are placed at the corners of a right angled triangle with mutually perpendicular sides equal to 2 m . Taking their point of intersection as the origin , find the position vector of centre of mass
View SolutionThree identical spheres each of mass M are placed at corners of right angle triangle with mutually perpendicular sides equal to 2m. Taking point of intersection of two mutually perpendicular sides as origin find position vector center of mass
View Solution
Knowledge Check
Three identical spheres each of mass M are placed at corners of right angle triangle with mutually perpendicular sides equal to 2m. Taking point of intersection of two mutually perpendicular sides as origin find position vector center of mass
 A(##PW_NEET_45_DAY_PHY_C06_E03_004_Q01##)B23(ˆi+ˆj)C43(ˆi+ˆj)D2(ˆi+ˆj)
A(##PW_NEET_45_DAY_PHY_C06_E03_004_Q01##)B23(ˆi+ˆj)C43(ˆi+ˆj)D2(ˆi+ˆj)Three identical spheres each of mass M are placed at corners of right angle triangle with mutually perpendicular sides equal to 2m. Taking point of intersection of two mutually perpendicular sides as origin find position vector center of mass
 A2(ˆi+ˆj)B(ˆi+ˆj)C2ˆi+ˆj3D4ˆi+ˆj3
A2(ˆi+ˆj)B(ˆi+ˆj)C2ˆi+ˆj3D4ˆi+ˆj3Three identical spheres of mass M each are placed at the corners of an equilateral triangle of side 2 m. Taking one of the corners as the origin, the position vector of the centre of mass is
A√3(ˆi−ˆj)Bˆi√3+ˆjCˆi+ˆj/3Dˆi+ˆj/√3
Three identical spheres of mass M each are placed at the corners of an equilateral triangle of side 2 m. Taking one of the corners as the origin, the position vector of the centre of mass is
View SolutionFour identical spheres each of mass m are placed at the corner of square of side 2 m. Taking the point of intersection of the diagonals as the orgin the coordinates of the centre of mass are ?
View SolutionThree particles each 1kg mass are placed at the corners of a right angled triangle AOB, O being the origin of the co-ordinate system OA and OB along +ve x-direction and +ve y-direction. The position vector of the centre of mass is (OA=OB=1m) (in meters)
View SolutionThree equal masses each of 50 g, are placed at the corners of a right angled isoceles triangle whose two equal sides are 5 cm each. The position of the centre of mass of the system is
View SolutionThree identical spheres of mass m each are placed at the corners of an equilateral triangle of side 2m taking one of the corner as the origin the position vector of the centre of mass is
View Solution
