Derive the folowing relation by the method of dimensions
T=Tension, =mass per unit length.Solution in Punjabi
Step by step video & image solution for Derive the folowing relation by the method of dimensionsv=sqrt(T/mu) T=Tension, mu=mass per unit length. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Can mechanical waves travel through vacuum?
View SolutionDefine unit
View SolutionWhat is the work done in holding a 15 kg suitcase whiel waiting for a bus for 15 mimnutes?
View SolutionThe frequency (f) of a stretched string depends upen the tension F (dimensions of form ) of the string and the mass per unit length mu of string .Derive the formula for frequency
View SolutionAssertion : Two strins shown in figure have the same tension. Speed of transverse waves in string -1 will be more. Reason : v prop (1)/sqrt(mu) ,Here mu is mass per unit length of string.
View SolutionThe figure represents two snaps of a travelling wave on a string of mass per unit length μ=0.25kg/m. The first snap is taken at t=0 and the second is taken at t=0.05s.
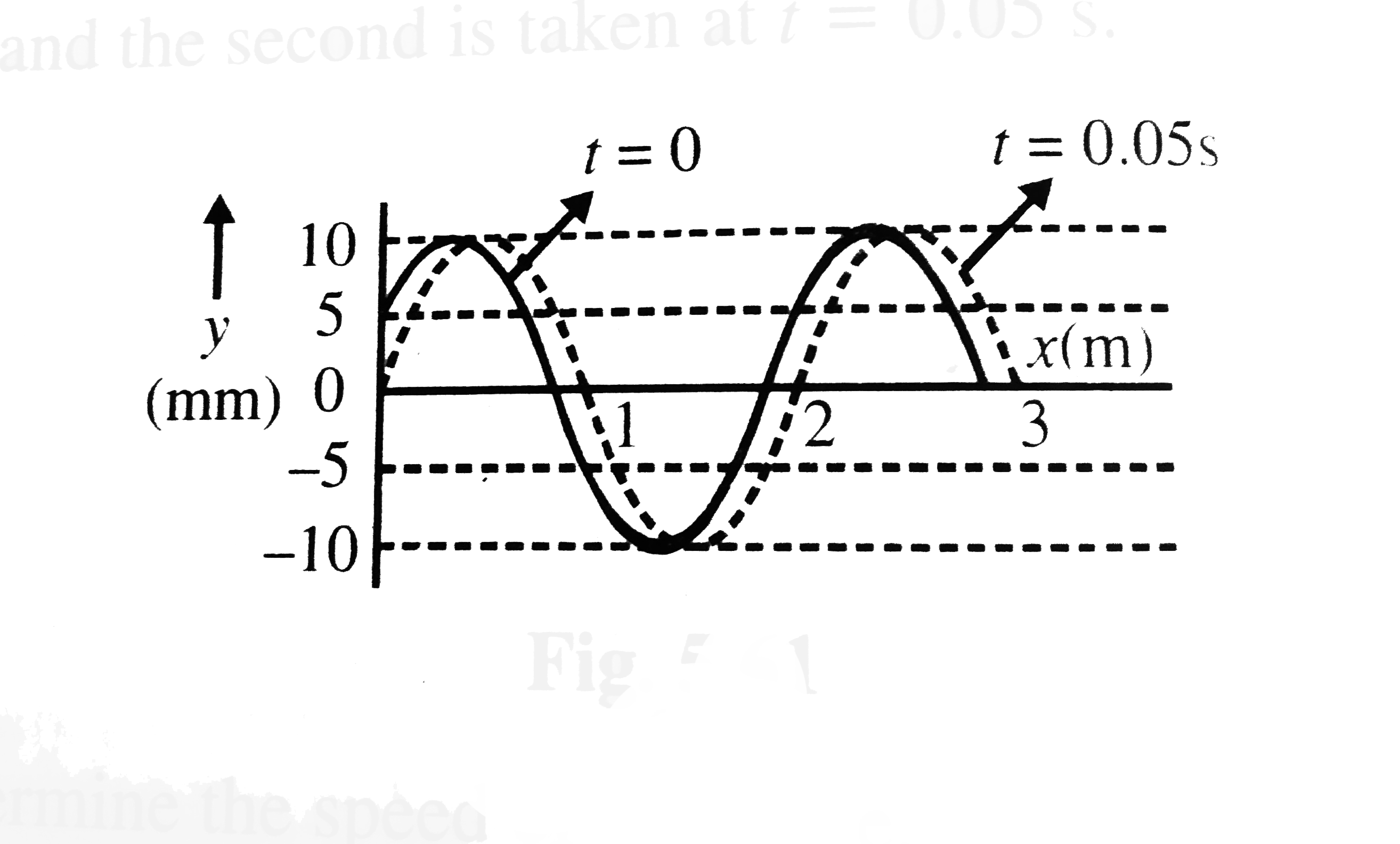
Determine the tension in the string.
View SolutionThe frequency of vibration (v) of a string may depend upon length (I) of the string, tension (T) in the string and mass per unit length (m) of the string. Using the method of dimensions, derive the formula for v.
View SolutionChack the correctness of the relations. (i) escape velocity, upsilon = sqrt((2GM)/(R )) (ii) v =(1)/(2l) sqrt((T)/(m)) , where l is length, T is tension and m is mass per unit length of the string.
View SolutionThe frequency f of vibration of a string between two fixed ends is proportional to LaTbμc, where L is the length of string, T is tension in the string and mu is the linear mass density (or mass per unit length) of string. Find the value of a,b and c.
View SolutionThe speed of transverse wave v in a stretched string depend on length tension T in the string and liner mass density (mass per unit length). μ. Find the relation using method of dimensions.
View SolutionA composite wire is made by joining two uniform wires. If l1=l2=l and μ1=μ29=μ. Tension in the strings is T , μ is mass per unit length. Then lowest frequency such that the junction is an antinode.

View SolutionDerive an expression for the velocity of pulse in a in stretched in string under a tension T and μ is the mass per unit length of the sting.
View SolutionThe unit of 1/l√(T/μ)) is the same as that of (l-length, T-tension and μ-mass/unit length)
View SolutionThe frequency (f) of a stretched string depends upen the tension F (dimensions of form ) of the string and the mass per unit length mu of string .Derive the formula for frequency
View SolutionAssertion : Two strins shown in figure have the same tension. Speed of transverse waves in string−1 will be more.
 Reason : v∝1√μ,Heremu is mass per unit length of string.
Reason : v∝1√μ,Heremu is mass per unit length of string.
View SolutionA composite wire is made by joining two uniform wires. If l1=l2=l and μ1=μ29=μ. Tension in the strings is T , μ is mass per unit length. Then lowest frequency such that the junction is an antinode.

View Solution
