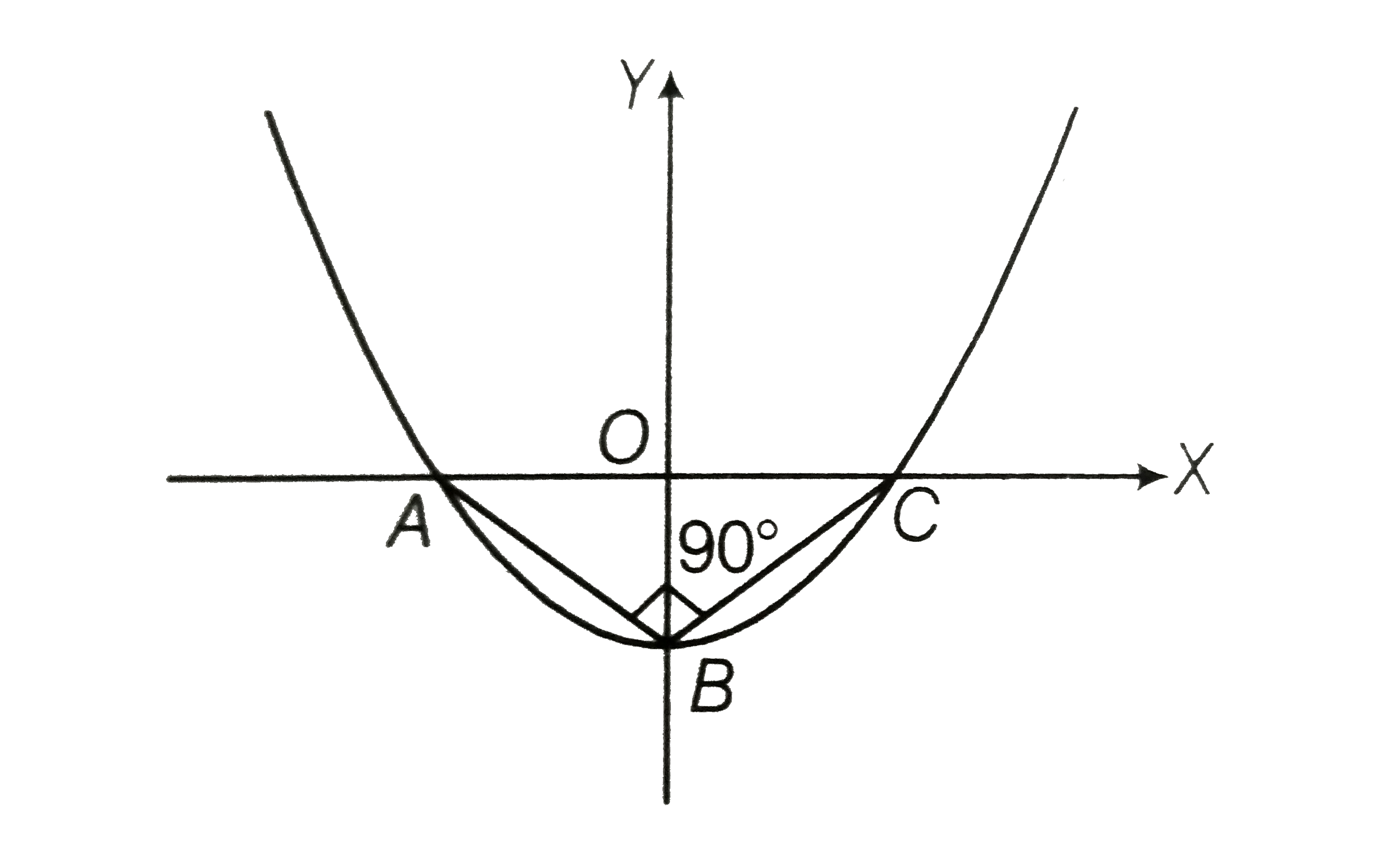
In the given figue vertices of ΔABC lie on y=f(x)=ax2+bx+c. The ΔAB is right angled isosceles triangle whose hypotenuse AC=4√2 units.
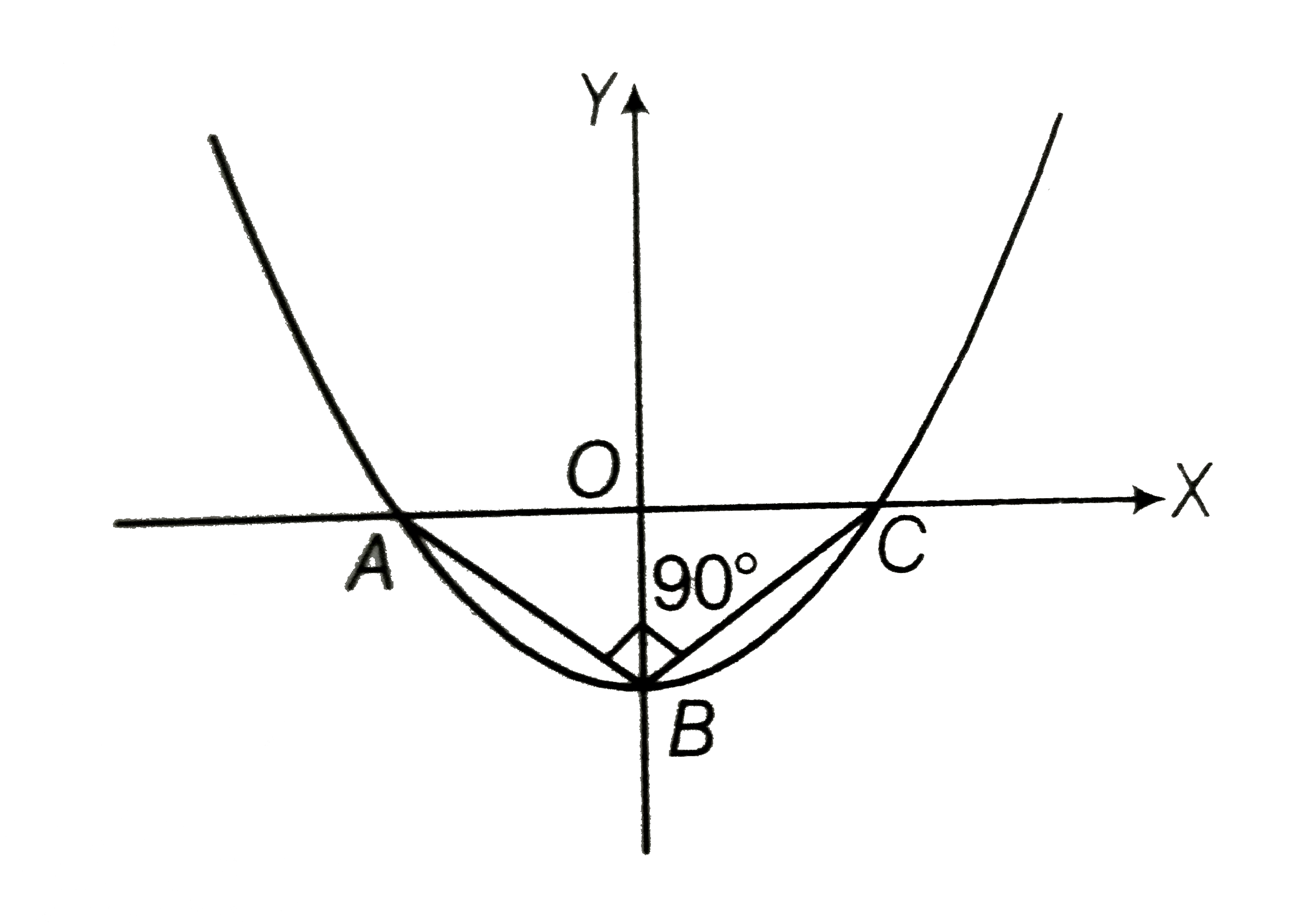
y=f(x) is given by Solution in Gujrati
y=−x2−8
y=x22√2−2√2
y=x2−4
y=x22−√2
The correct Answer is:B
Given that AC=4√2 units
∴AB=BC=AC√2=4 units andd OB=√(BC)2−(OC)2
=√(4)2−(2√2)2[∵OC=AC2]
=2√2 units
∴ Vertices are A=(−2√2,0),
B=−(0,−2√2)
and C=(2√2,0)
Since y=f(x)=ax2+bx+c passes through A,B and C then
0=8a−2√2b+c−2√2=c
and 0=8a+2√2b+c
We get b=0,a=12√20 and c=−2√2
∴y=f(x)=x22√2−2√2
Similar Questions
In the given figue vertices of ΔABC lie on y=f(x)=ax2+bx+c. The ΔAB is right angled isosceles triangle whose hypotenuse AC=4√2 units.
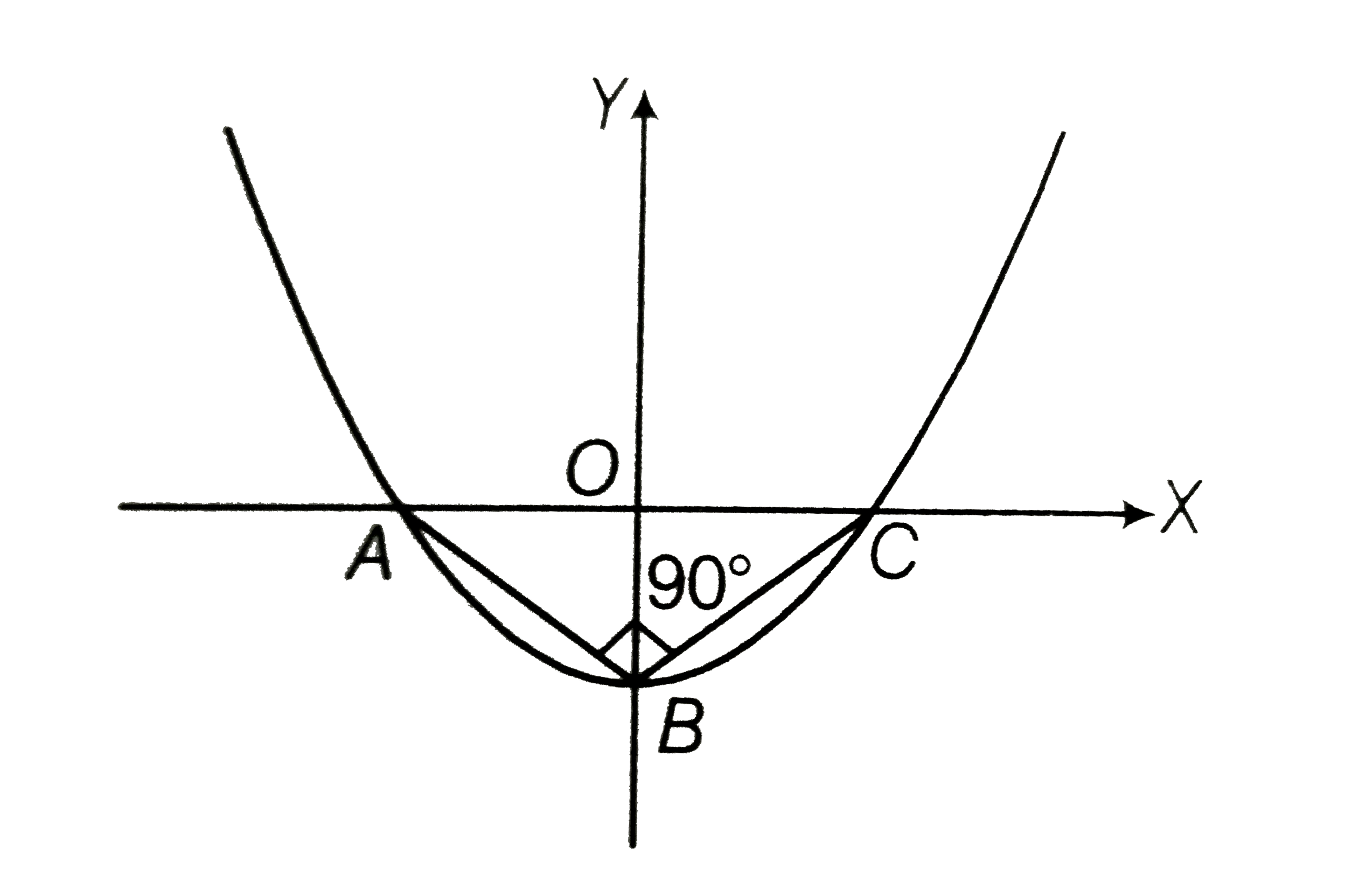
Number of integral value of λ for which λ2 lies between the roots of f(x)=0, is
View Solutionदिये गये चित्र में ΔABC के शीर्ष y=f(x)=ax2+bx+c पर स्थित है ΔABC समकोण समद्विबाहु त्रिभुज है जिसका कर्ण AC=4√2 इकाई है, तब

y=f(x) दिया गया है
View Solutionदिये गये चित्र में ΔABC के शीर्ष y=f(x)=ax2+bx+c पर स्थित है ΔABC समकोण समद्विबाहु त्रिभुज है जिसका कर्ण AC=4√2 इकाई है, तब
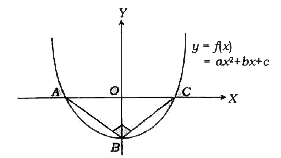
समीकरण y=f(x) का न्यूनतम मान है
View Solutionदिये गये चित्र में ΔABC के शीर्ष y=f(x)=ax2+bx+c पर स्थित है ΔABC समकोण समद्विबाहु त्रिभुज है जिसका कर्ण AC=4√2 इकाई है, तब
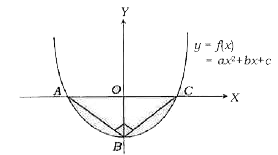
k के पूर्णांक मानों की संख्या जिसके लिए k2,f(x)=0 के मूलों के बीच स्थित है
View SolutionIn the given figue vertices of ΔABC lie on y=f(x)=ax2+bx+c. The ΔABC is right angled isosceles triangle whose hypotenuse AC=4√2 units.
Minimum valueof y=f(x) is
View SolutionIn the given figue vertices of ΔABC lie on y=f(x)=ax2+bx+c. The ΔAB is right angled isosceles triangle whose hypotenuse AC=4√2 units.

Number of integral value of λ for which λ2 lies between the roots of f(x)=0, is
View SolutionIn the given figure, vertices of Delta ABC lie on y = f(x) = ax^2 + bx + c. The DeltaABC is right angled isosceles triangle whose hypotenuse AC = 4sqrt2 units. Number of integral values of k for which one root of f(x) = 0 is more than k and other less than k
View SolutionIn the given figue vertices of ΔABC lie on y=f(x)=ax2+bx+c. The ΔAB is right angled isosceles triangle whose hypotenuse AC=4√2 units.

Minimum valueof y=f(x) is
View Solution
