Two immiscible of densities 2.5g/cm3 and 0.8g/cm3 are taken in the ratio of their masses as 2:3 respectively find the average density of the liquid conbination.Solution in Gujrati
Let masses be 2m g & 3m, g, then V=V1+V2=(2m2.5+3m0.8)cm3
total mass =2m+3m+5mg
therefore the average desity ρ=5mV=5m2m2.5+3m0.8=522.5+30.8=109.1gm/cm3=1.09gm/cm3
Topper's Solved these Questions
- BOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 1 (Elasticity)16videos
ELASTICITY, SURFACE TENSION AND FLUID MECHANICS
- BOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 1 (Surface Tension)21videos
ELASTICITY, SURFACE TENSION AND FLUID MECHANICS
- BOOK - ALLEN CHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V B19videos
CENTRE OF MASS
- BOOK - ALLEN CHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)22videos
ERROR AND MEASUREMENT
Similar Questions
Which one of the following substances possesses the highest elasticity-
03:13View SolutionThe length of needle foating on the surface of water is 1.5 cm the force in addition to its weight required to lift the needle from water surface will be (surface tension of water =7.5N/cm)
03:22View SolutionTwo particles of masses m1 and m2 in projectile motion have velocities →v1 and →v2 , respectively , at time t=0. They collide at time t0. Their velocities become →v'1 and →v'2 at time 2t0 while still moving in air. The value of ∣∣∣(m1→v'1+m2→v'2)−(m1→v1+m2→v2)∣∣∣
View SolutionA cube has a side of length 1.2×10−2m. Calculate its volume.
03:53View SolutionTwo discs one of density 7.2 g//cm^(3) and the other of density 8.9g//cm^(3) are of same mass and thickness. Their moments of inertia are in the ratio
05:24View SolutionA metallic sphere floats in immiscible mixture of water (density 10^(3) kgm^(-3)) and a liquid (density 8xx10^(3) kgm^(-3)) such that its (2//3) part is in water and (1//3) part in the liquid. The density of the metal is
02:02View SolutionAn object floats in three immiscible liquids A, B and C of densities 3g cm−3,2g cm−3, respectively as shown in the figure. When the object is placed in the liquids, the levels of liquid A, B and C rise by 3 cm, 5 cm and 8 cm, respectively. The areas of cross-sections of the container and the object are 10 cm2and5cm2, respectively. Calculate the density of the object.
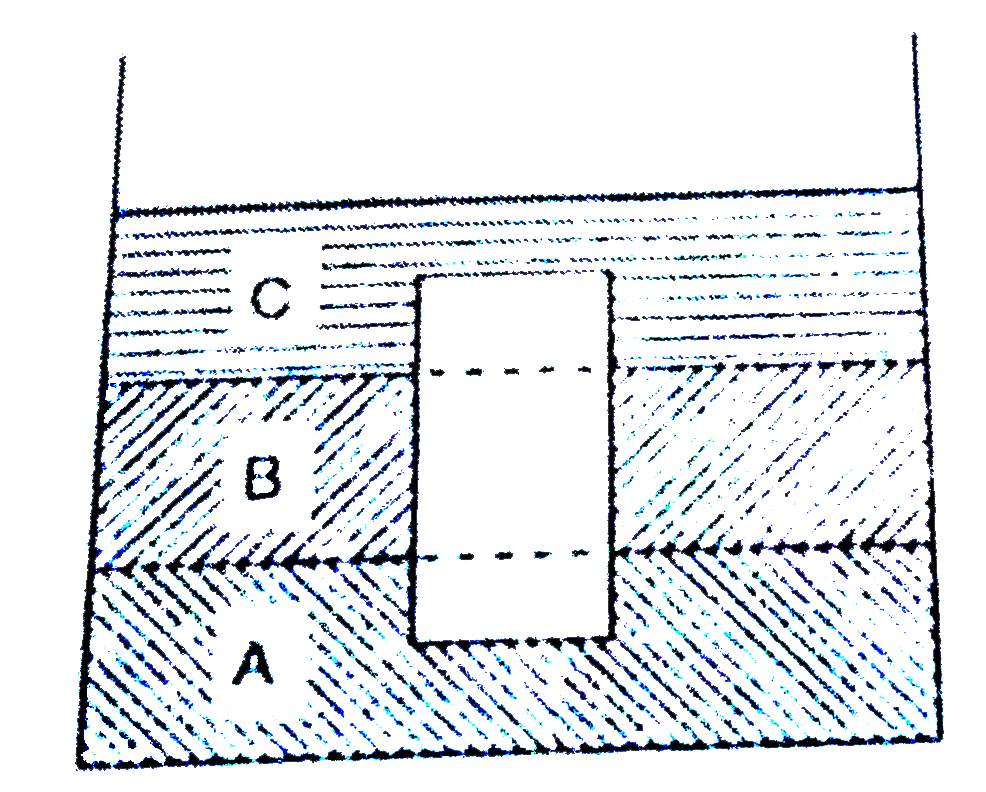 03:02View Solution
03:02View SolutionTwo identical containers A and B are filled with two different liquids of equal masses. The level of the liquid in container A is found to be one-fourth of the level of the liquid in container B. What is the ratio of thedensity of two liquids? If the density of he liquid in the container A is 2 " g " cm^(-3) , then find the density of the mixture of the two liquids.
03:17View SolutionDensity of water is 1.00 g//cm^(3) and density of ethanol is 0.9 g//cm^(3) . If these two liquids were mixed to prepare a dilute solution then
04:58View SolutionHow much of a liquid of density 1.3g⋅cm−3 can be stored in a container of 5 kg of kerosene oil? Density of kerosene = 0.8g⋅cm−3. Give your answer in kg.
View SolutionA glass ball of density 2.6g*cm^(-3) is coated with a thick layer of wax of density 0.8g*cm^(-3) . If the combination floats in water remaining completely submerged, find the ratio of the volume of wax to that of the glass ball.
05:34View SolutionTwo discs one of density 7.2 g cm^(-3) and other of density 8.9 g cm^(-3) are of same mass and thickness. Their M.I. are in the ratio of :
View SolutionA block of density rho_(1) floats in two immiscible liquids of density 4 rho and 2 rho , such that (1/4)^(th) portion is in liquid of density 4 rho and remaining liquid of density 2 rho ,then rho_(1) =_________ g/(cm)^3 (Given rho=0.8g/(cm)^3 )
03:00View Solution10g of a liquid of density 5g cm^(-3) is mixed with 12g of another miscible liquid of density 4g cm^(-3) . Find the density of mixture.
03:09View Solutionदो परस्पर अघुलनशील द्रव का द्रव्यमान जिनका घनत्व 2.5g/cm3 और 0.8g/cm3 है 2:3 है का औसत घनत्व ज्ञात कीजिए।
06:47View SolutionTwo discs one of density 7.2 g/cm3 and the other of density 8.9 g/cm3 are of same mass and thickness. Their moments of inertia are in the ratio
05:21View Solution

