[Gujrati] When jet liquid strikes a fixed or moving surface it exerts

In the figure shown the straing versus stress graph for two values of young's modulus ?
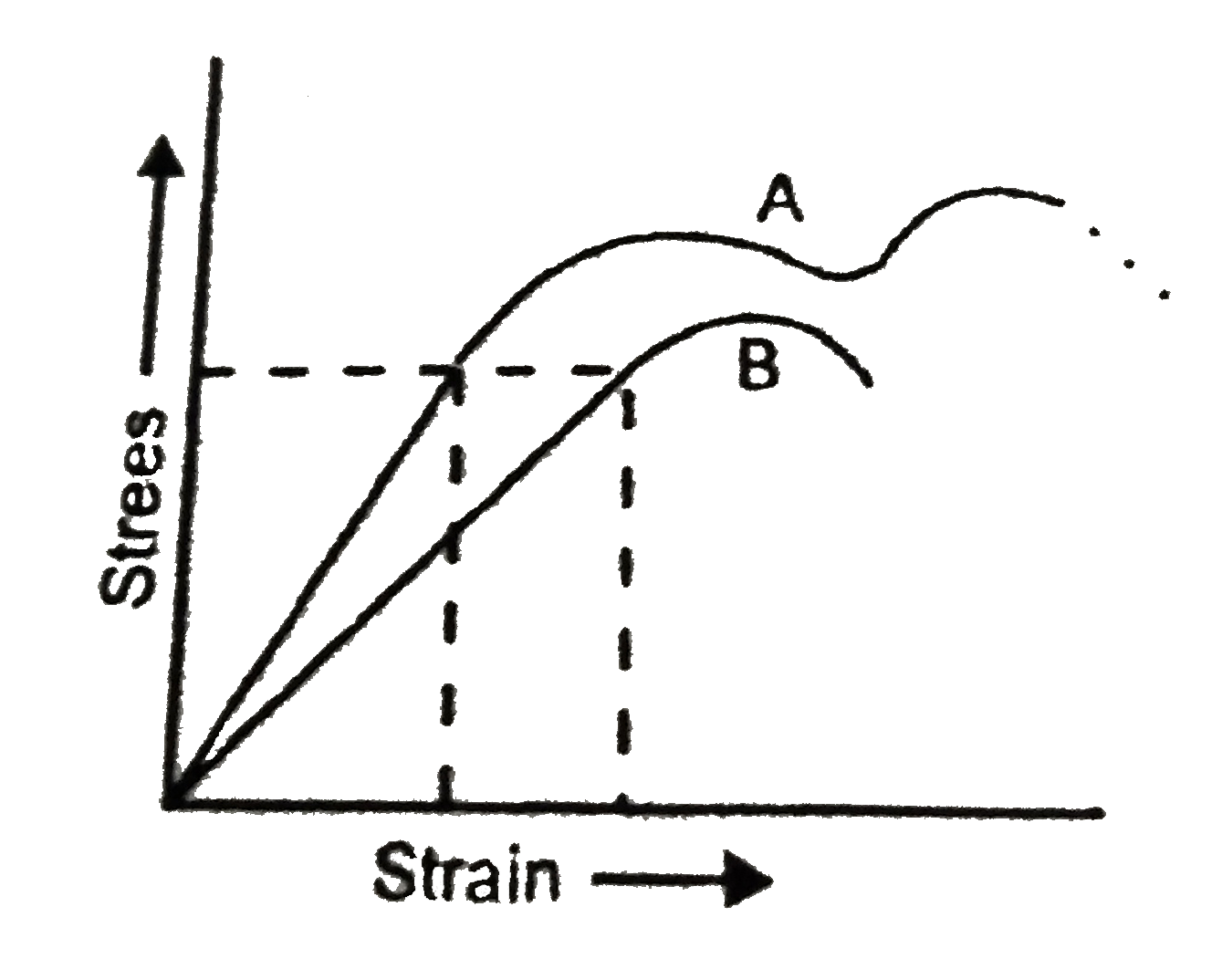
ocr_image
$(i)$ which material is more ductile ? Explain.
$(i i)$ Which material is more brittle ? Explain.
$(i i i)$ Which material is stronger ? Explain.

View Solution

ocr_image
PQRS is a rectangular frame of copper wire shown in figure the side RS of the frame is movable. If a soap film is formed on it then what is the diameter of the wire to maintain equilibirum (given surface tension of soap solution $= 0.045 N / m$ and density of copper $= 8.96 \times 10^{3} k g / m^{3})$

View Solution

Statement-1: A solid sphere and a hollow sphere of same material are floating in a liquid radius of both the spheres are same percentage of volume immersed of both the spheres will be same.
Statement-2: Upthust acts on volume of liquid displaced. It has nothing to do whether the body is solid or hollow.

View Solution

Two particles of masses $m_{1}$ and $m_{2}$ in projectile motion have velocities ${\to v}_{1}$ and ${\to v}_{2}$ , respectively , at time $t = 0$ . They collide at time $t_{0}$ . Their velocities become ${\to v'}_{1}$ and ${\to v'}_{2}$ at time $2 t_{0}$ while still moving in air. The value of $∣ ∣ ∣ (m_{1} {\to v'}_{1} + m_{2} {\to v'}_{2}) - (m_{1} {\to v}_{1} + m_{2} {\to v}_{2}) ∣ ∣ ∣$

View Solution

A cube has a side of length $1.2 \times 10^{- 2} m$ . Calculate its volume.

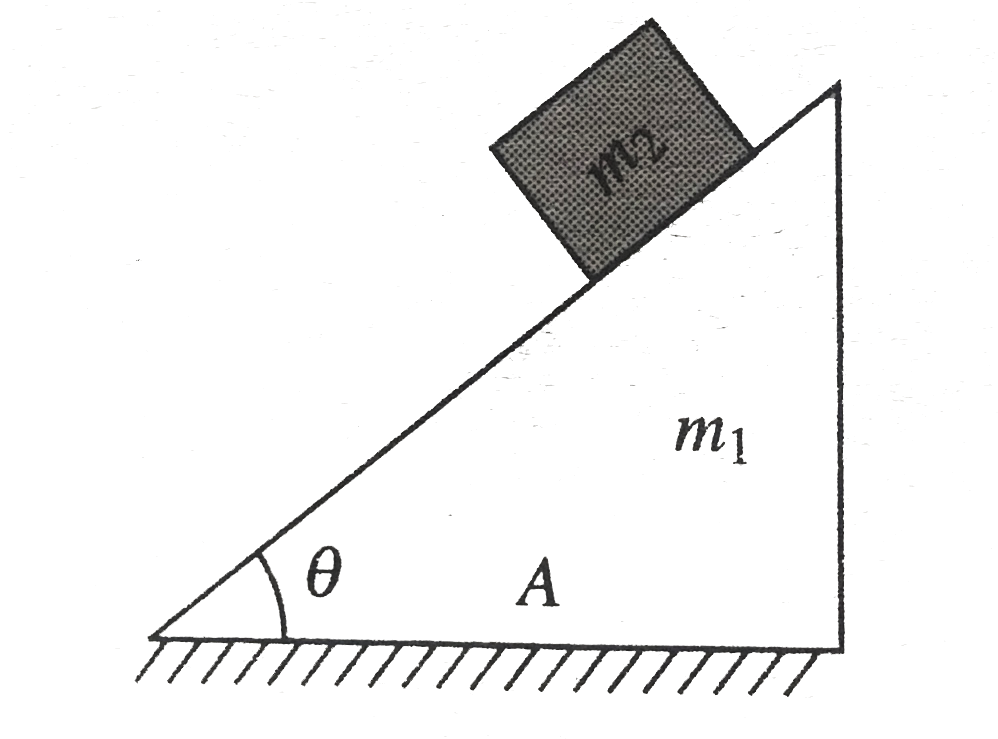
View Solution

In fig., the mass $m_{2}$ starts with velocity $v_{0}$ and moves with constant velocity on the surface. During motion, the normal reaction between the horizontal surface and fixed triangle block $m_{1}$ is $N$ . Then during motion.
ocr_image

View Solution

When a jet of liquid strikes a fixed or moving surface, it exerts thrust on it due to rate of change of momentum. F=(rhoAV_(0))V_(0)-(rhoAV_(0))V_(0)costheta=rhoAV_(0)^(2)[1-costheta] If surface is free and starts moving due to thrust of the liquid, then at any instant, the above equation gets modified based on relative change of momentum with respect to surface. Let, at any instant, the velocity of surface be u . Then the above equation becomes F=rhoA(V_(0)-u)^(2)[1-costheta] Based on the above concept, as shown in the following figure, if the cart is frictionless and free to move in the horizontal direction, then answer the following. Given that cross sectional area of jet =2xx10^(-4)m^(2) velocity of jet V_(0)=10m//s density of liquid =1000kg//m^(3) ,mass of cart M=10 kg . Initially ( l=0 ) the force on the cart is equal to

View Solution

When a jet of liquid strikes a fixed or moving surface, it exerts thrust on it due to rate of change of momentum.
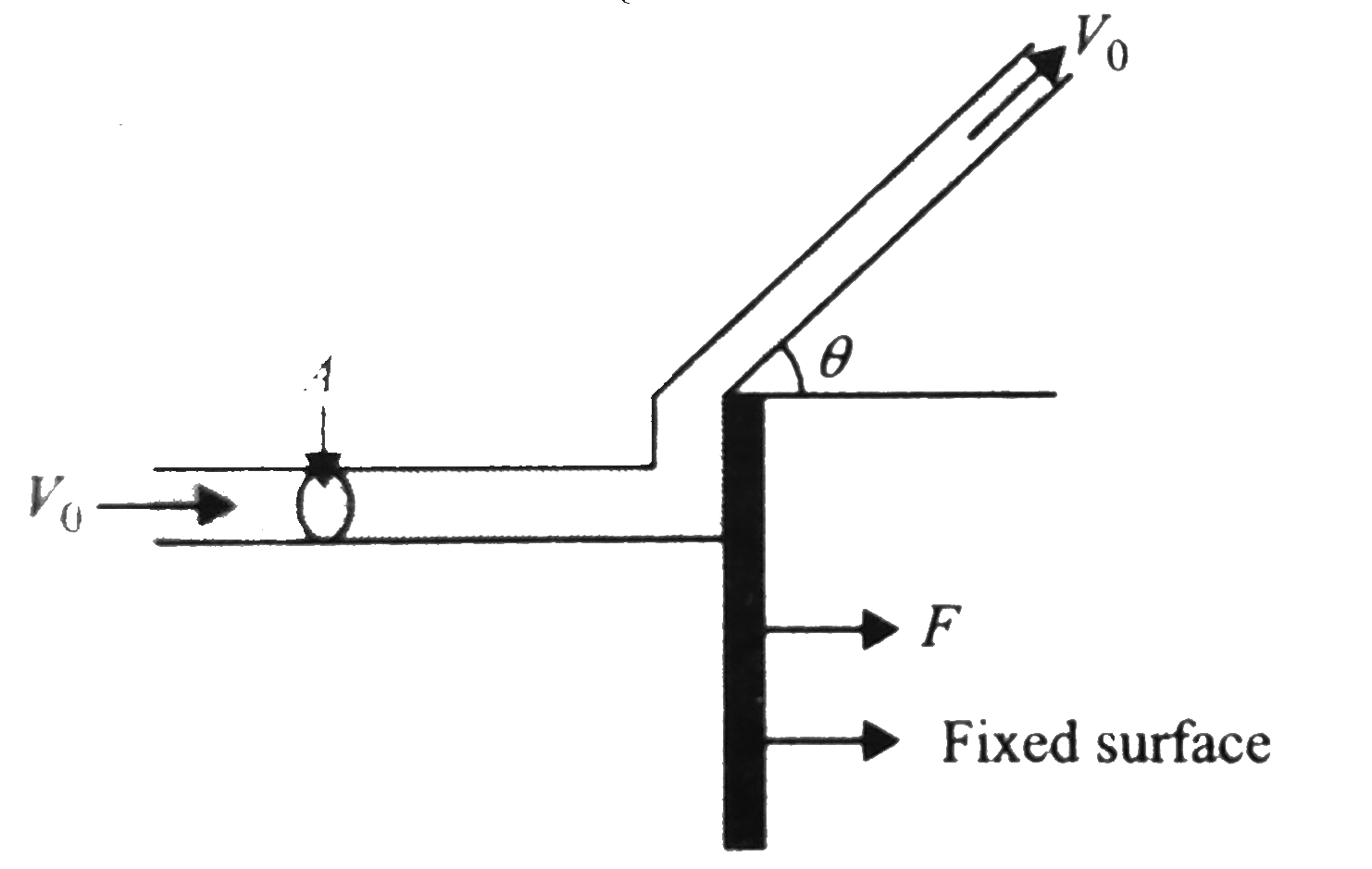
ocr_image
$F = (ρ A V_{0}) V_{0} - (ρ A V_{0}) V_{0} cos θ = ρ A V_{0}^{2} [1 - cos θ]$ If surface is free and starts moving due to thrust of the liquid, then at any instant, the above equation gets modified based on relative change of momentum with respect to surface. Let, at any instant, the velocity of surface be $u$ . Then the above equation becomes $< b r > F = ρ A {(V_{0} - u)}_{0}^{2} [1 - cos θ]$ Based on the above concept, as shown in the following figure, if the cart is frictionless and free to move in the horizontal direction, then answer the following.
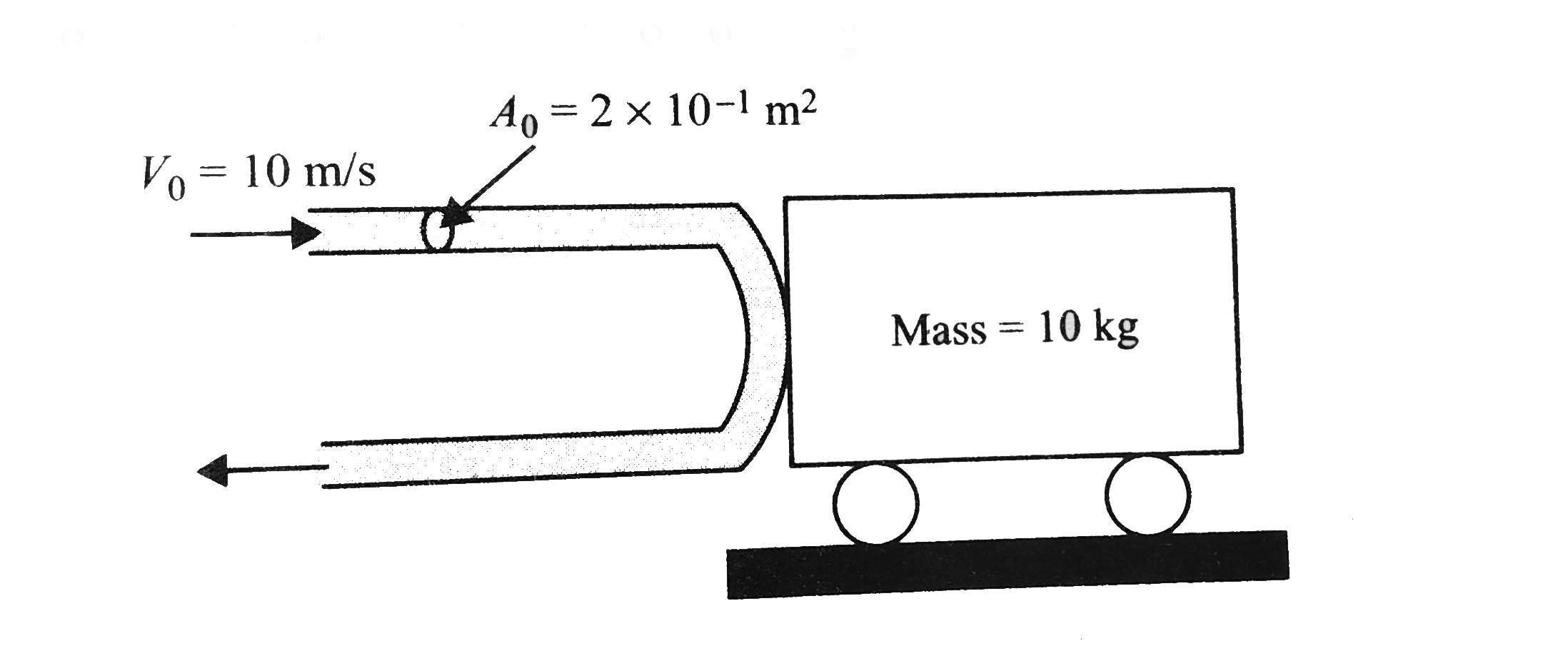
ocr_image
Given that cross sectional area of jet $= 2 \times 10^{- 4} m^{2}$ velocity of jet $V_{0} = 10 m / s$ density of liquid $= 1000 k g / m^{3}$ ,mass of cart $M = 10 k g$ .
Velocity of cart at $t = 10 s$ is equal to

View Solution

When a jet of liquid strikes a fixed or moving surface, it exerts thrust on it due to rate of change of momentum. F=(rhoAV_(0))V_(0)-(rhoAV_(0))V_(0)costheta=rhoAV_(0)^(2)[1-costheta] If surface is free and starts moving due to thrust of the liquid, then at any instant, the above equation gets modified based on relative change of momentum with respect to surface. Let, at any instant, the velocity of surface be u . Then the above equation becomes F=rhoA(V_(0)-u)^(2)[1-costheta] Based on the above concept, as shown in the following figure, if the cart is frictionless and free to move in the horizontal direction, then answer the following. Given that cross sectional area of jet =2xx10^(-4)m^(2) velocity of jet V_(0)=10m//s density of liquid =1000kg//m^(3) ,mass of cart M=10 kg . The time at which velocity of cart becomes 2 m/s is equal to

View Solution

When a jet of liquid strikes a fixed or moving surface, it exerts thrust on it due to rate of change of momentum. F=(rhoAV_(0))V_(0)-(rhoAV_(0))V_(0)costheta=rhoAV_(0)^(2)[1-costheta] If surface is free and starts moving due to thrust of the liquid, then at any instant, the above equation gets modified based on relative change of momentum with respect to surface. Let, at any instant, the velocity of surface be u . Then the above equation becomes F=rhoA(V_(0)-u)^(2)[1-costheta] Based on the above concept, as shown in the following figure, if the cart is frictionless and free to move in the horizontal direction, then answer the following. Given that cross sectional area of jet =2xx10^(-4)m^(2) velocity of jet V_(0)=10m//s density of liquid =1000kg//m^(3) ,mass of cart M=10 kg . The power supplied to the cart when its velocity becomes 5 m//s is equal to

View Solution

The fraction of a floating object of volume $V_{0}$ and density $d_{0}$ above the surface of a liquid of density d will be

View Solution

A sphere is moving on a smooth surface with linear speed $v_{0}$ and angular velocity $ω_{0}$ . It finds a rough inclined surface and it starts climbing up:
ocr_image

View Solution

A particle moves with initial velocity v_(0) and retardation alphav , where v is velocity at any instant t. Then the particle

View Solution

A sphere moving with a speed v_(0) on a smooth surface suddenly enters on a rough horizontal surface as shown. The angular momentum of the sphere about any point on the surface is conserved.

View Solution

$V_{0}$ आयतन व $d_{0}$ घनत्व का पिंड, d घनत्व वाले द्रव में तैर रहा है। पिंड के आयतन का वह भाग क्या होगा जो द्रव की सतह के ऊपर है।

View Solution

In fig., the mass m_(2) starts with velocity v_(0) and moves with constant velocity on the surface. During motion, the normal reaction between the horizontal surface and fixed triangle block m_(1) is N . Then during motion.

View Solution

The correct Answer is:D

Topper's Solved these Questions

Similar Questions

ALLEN -ELASTICITY, SURFACE TENSION AND FLUID MECHANICS-Exercise 3 (Comprehension based questions)