A spherical solild of volume V is made of a material of density ρ1. It is falling through a liquid of density ρ2(ρ2<ρ1). Assume that the liquid applies a viscous froce on the ball that is proportional ti the its speed v, i.e., Fviscous=−kv2(k>0). The terminal speed of the ball is Solution in Gujrati
⎷(Vg(ρ1−ρ2)k)
Vgρ1k
√Vgρ1k
(Vg(ρ1−ρ2)k)
The correct Answer is:A
Step by step video & image solution for A spherical solild of volume V is made of a material of density rho_(1). It is falling through a liquid of density rho_(2)(rho_(2) lt rho_(1)). Assume that the liquid applies a viscous froce on the ball that is proportional ti the its speed v, i.e., F_(viscous)=-kv^(2)(kgt0). The terminal speed of the ball is by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (MCQ)
13 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Multiple correct answers)
2 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 4 B (Bran storming Subjective Exercise)
11 Videos
- CENTRE OF MASSBOOK - ALLEN CHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V B
19 Videos
- ERROR AND MEASUREMENTBOOK - ALLEN CHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
Similar Questions
Water from a tap emerges vertically downwards with an initial spped of 1.0ms−1. The cross-sectional area of the tap is 10−4m2. Assume that the pressure is constant throughout the stream of water, and that the flow is steady. The cross-sectional area of the stream 0.15 m below the tap is
View SolutionA solid sphere of radius R and density ρ is attached to one end of a mass-less spring of force constant k. The other end of the spring is connected to another solid sphere of radius R and density 3ρ. The complete arrangement is placed in a liquid of density 2ρ and is allowed to reach equilibrium. The correct statements(s) is (are)
View SolutionA container of large uniform cross-sectional area A resting on a horizontal surface, holes two immiscible, non-viscon and incompressible liquids of densities d and 2d each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0. A homogeneous solid cylinder of length L(L<H/2) and cross-sectional area A/5 is immeresed such that it floats with its axis vertical at the liquid-liquid interface with length L/4 in the denser liquid,
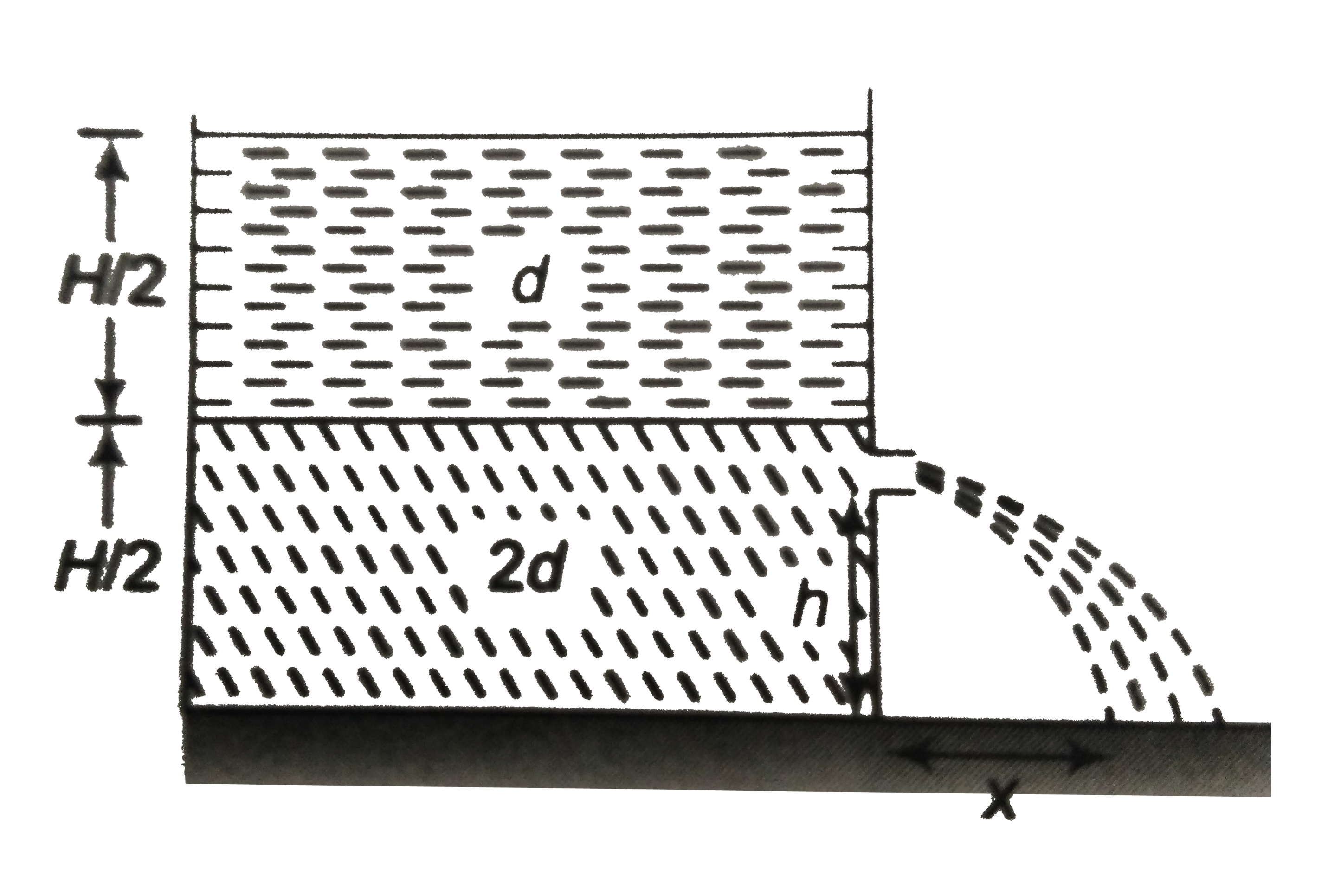
The cylinder is then removed and the original arrangement is restroed. a tiny hole of area s(s<<A) is punched on the vertical side of the container at a height h(h<H/2). As a result of this, liquid starts flowing out of the hole with a range x on the horizontal surface.
The total pressure with cylinder, at the bottom of the container is
View SolutionTwo particles of masses m1 and m2 in projectile motion have velocities →v1 and →v2 , respectively , at time t=0. They collide at time t0. Their velocities become →v'1 and →v'2 at time 2t0 while still moving in air. The value of ∣∣∣(m1→v'1+m2→v'2)−(m1→v1+m2→v2)∣∣∣
View SolutionA cube has a side of length 1.2×10−2m. Calculate its volume.
View SolutionA spherical solid ball of volume V is made of a material of density ρ1. It is falling through a liquid of density ρ2(ρ2<ρ1). Assume that the liquid applies a viscous force on the ball that is proportional to the square of its speed v, i.e., Fviscous=−kv2(k>0). The terminal speed of the ball is
View SolutionA ball of volume V and density rho_(1) is moved downwards by a distance 'd' in liquid of density rho_(2) . Find total change in potential energy of the system.
View SolutionA solid uniform ball having volume V and density rho floats at the interface of two unmixible liquids as shown in Fig. 7(CF).7. The densities of the upper and lower liquids are rho_(1) and rho_(2) respectively, such that rho_(1) lt rho lt rho_(2) . What fractio9n of the volume of the ball will be in the lower liquid.
View SolutionA spherical solild of volume V is made of a material of density rho_(1) . It is falling through a liquid of density rho_(2)(rho_(2) lt rho_(1)) . Assume that the liquid applies a viscous froce on the ball that is proportional ti the its speed v, i.e., F_(viscous)=-kv^(2)(kgt0) . The terminal speed of the ball is
View SolutionA body of density rho floats with a volume V_(1) of its total volume V immersed in one liquid of density rho_(1) and with the remainder of volume V_(2) immersed in another liquid of density rho_(2)," ""where"" "rho_(1)gt rho_(2). Find the relative volumes immersed in two liquids.
View SolutionA solid ball of density ρ1 and radius r falls vertically through a liquid of density ρ2. Assume that the viscous force acting on the ball is F=krv, where k is a constant and v its velocity. What is the terminal velocity of the ball ?
View Solutionएक गोलाकार ठोस गेंद जिसका आयतन V है, घनत्व ρ1 के पदार्थ से बनी है | यह घनत्व ρ2(ρ2<ρ1) वाले द्रव में गिर रही है | यदि द्रव द्वारा गेंद पर लगने वाला श्यान बल, चाल v के वर्ग के अनुक्रमानुपाती है अर्थात Fश्यान=−kv2 ( जहाँ k>0) , तो गेंद की अन्त्य चाल होगी :
View SolutionV आयतन की एक ठोस गोलीय गेंद ρ1 घनत्व के पदार्थ से बना है । यह ρ2(ρ2<ρ1) घनत्व के द्रव में गिर रही है । यदि द्रव गेंद पर श्यान बल को लगाता हो जो की गेंद के वेग के वर्ग के अनुक्रमानुपाती हो अर्थात श्यानता बल F=−kv2(k>0) तो गेंद की सीमांत चाल होगी -
View SolutionA spherical solid ball of volume V is made of a material of density p1 It is falling through a liquid of density p_(1) (p_(2) lt p_(1)) . Assume the that the liquid applies a viscous force on the ball that is proportional to the square of its speed v, i.e., F_("viscous") = - kv^(2)(k gt 0) . The terminal speed of the ball is
View SolutionA spherical solild of volume V is made of a material of density rho_(1) . It is falling through a liquid of density rho_(2)(rho_(2) lt rho_(1)) . Assume that the liquid applies a viscous froce on the ball that is proportional ti the its speed v, i.e., F_(viscous)=-kv^(2)(kgt0) . The terminal speed of the ball is
View SolutionA solid uniform ball of volume V floats on the interface of two immiscible liquids. The specific gravity of the upper liquid is rho_(1) and that of lower one is rho_(2) and the specific gravity of ball is rho(rho_(1) lt rho lt rho_(2)) . The fraction of the ball in the upper liquid Is
View Solution