_S01_025_Q01.png)
In Young's double slit experiment the slits, S1&S2 are illuminated by a parallel beam of light of wavelength 4000 Å from the medium of refractive index n1=1.2 A thin film of thickness 1.2 μm and refractive index n=1.5 is placed infrom of S1 perpedicular to path of light. the refractive index of medium between plane of slits & screen is n2=1.4 if the light coming from the film and S1&S2 have equal intensities I then intensity at geometrical centre of the screen O is Solution in Gujrati
0
2I
4I
None of these
The correct Answer is:B
Path difference at O: (μrel−1)t=(nn2−1)t
∴phase difference at O: t(nn2−1)πλ2 where n1λ1−n2λ2
⇒ phase difference =π2⇒Resultant intensity =2I
Topper's Solved these Questions
- WAVE OPTICSBOOK - ALLEN CHAPTER - WAVE OPTICSEXERCISE - Example 8
1 Videos
- WAVE OPTICSBOOK - ALLEN CHAPTER - WAVE OPTICSEXERCISE - Example 9
1 Videos
- WAVE OPTICSBOOK - ALLEN CHAPTER - WAVE OPTICSEXERCISE - Example 6
1 Videos
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR BOOK - ALLEN CHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (J-A)
7 Videos
Similar Questions
_S01_026_Q01.png)
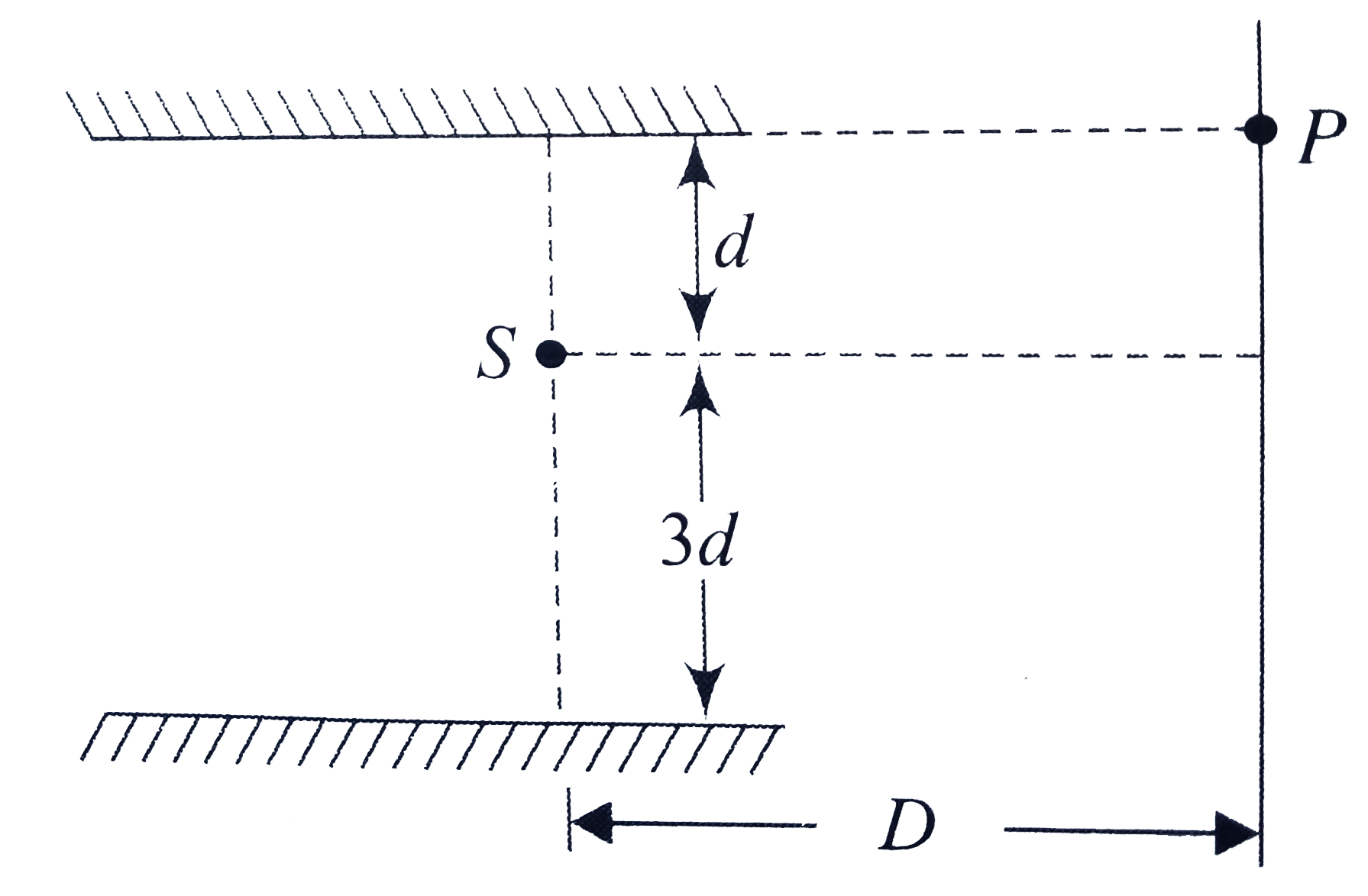
In a YDSE experiment two slits S1 and S2 have separation of d=2mm the distance of the screen is D=85 m source S starts moving from a very large distance towards S2 perpendicular to S1S2 as shown in figure the wavelength of monochromatic light is 500 n. The number of maximas observed on the screen at point P as the moves towards S2 is
View SolutionConsider the optical system shown in figure. The point source of ligth S is having wavelength equal to λ. The light is reaching screen only after reflection. For point P to be socond maxima, the value of λ would be (D>>d and d>>λ)
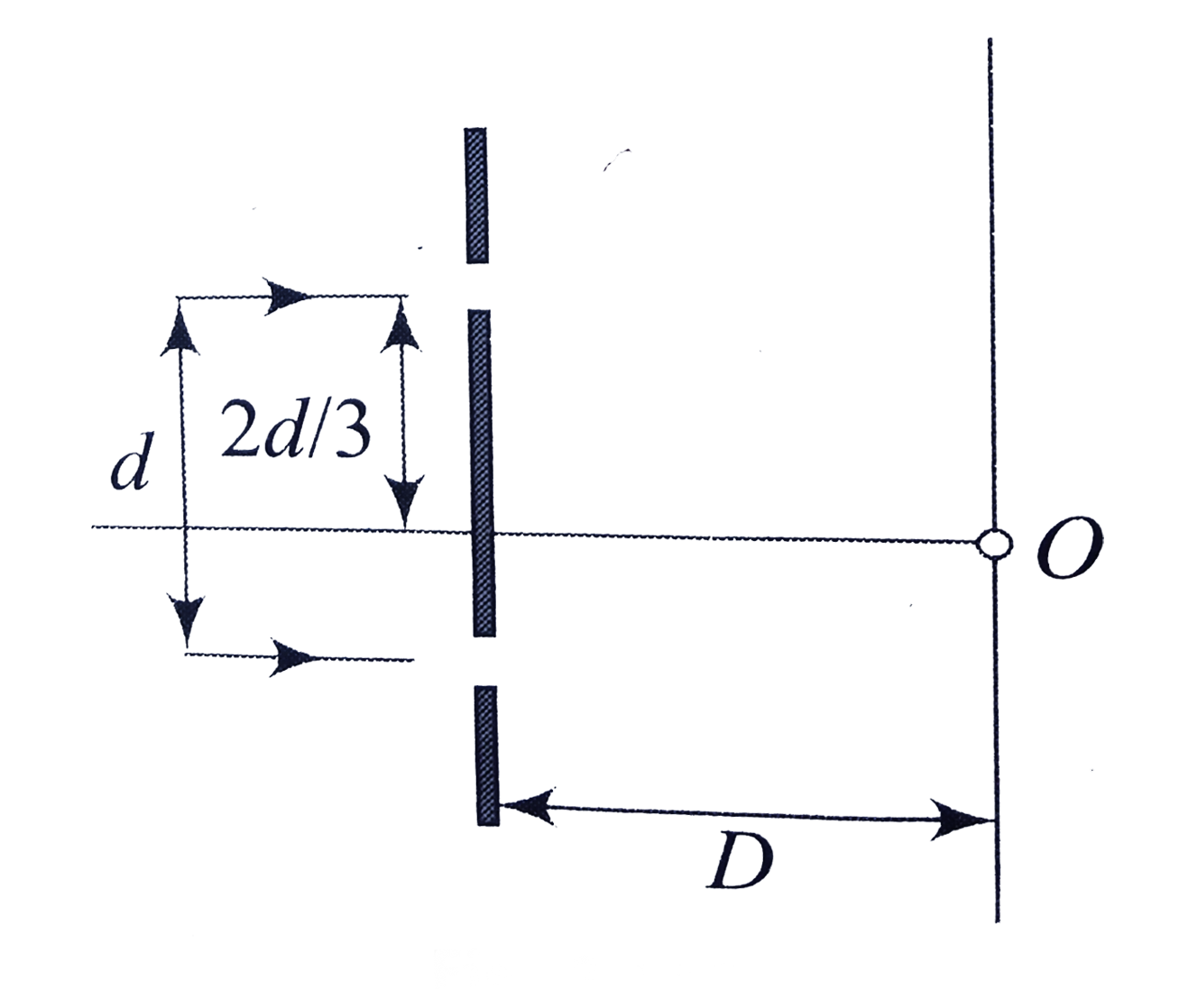
View SolutionIn figure, if a parallel beam of white light is incident on the plane of the slit, then the distance of the nearest white spot on the screen form O is [assume d<<D,λ<<D]
View SolutionMatch List I with List II and select the correct answer using the codes given below the lists :

View SolutionIn figure S is a monochromatic source of light emitting light of wavelength of wavelength lambda (in air). Light on slits S_(1) from S and then reaches in the slit S_(2) and S_(3) through a medium of refractive index mu_(1) . Light from slit S_(2) and S_(3) reaches the screen through a medium of refractive index mu_(3) . A thin transparent film of refractive index mu_(2) and thickness t is used placed in front of S_(2) . Point P is symmetrical w.r.t. S_(2) and S_(3) . Using the values d = 1 mm, D = 1 m, mu_(1) = 4//3 , mu_(2) = 3//2, mu_(3) = 9//5 , and t = (4)/(9) xx 10^(-5) m , a. find distance of central maxima from P, b. If the film in front of S_(2) is removed, then by what distance and in which direction will be central maxima shift ? .
View SolutionIn YDSE shown in figure a parallel beam of light is incident on the slits from a medium of refractive index n_(1) . The wavelength of light in this medium is lambda_(1) . A transparent of thickness t and refractive index n_(3) is put in front of one slit. The medium between the screen and the plane of the slits is n_(2) . The phase difference between the light waves reaching point O (symmetrical, relative to the slits) is
View SolutionA YDSE is performed in a medium of refractive index 4/3, A light of 600 nm wavelength is falling on the slits having 0.45 nm separation . The lower slit S2 is covered b a thin glass plate of thickness 10.4 mm and refractive index 1.5. The interference pattern is observed on a screen placed 1.5 m from the slits as shown in figure. (All the wavelengths in this problem are for the given medium of refractive index 4/3, ignore absorption.)
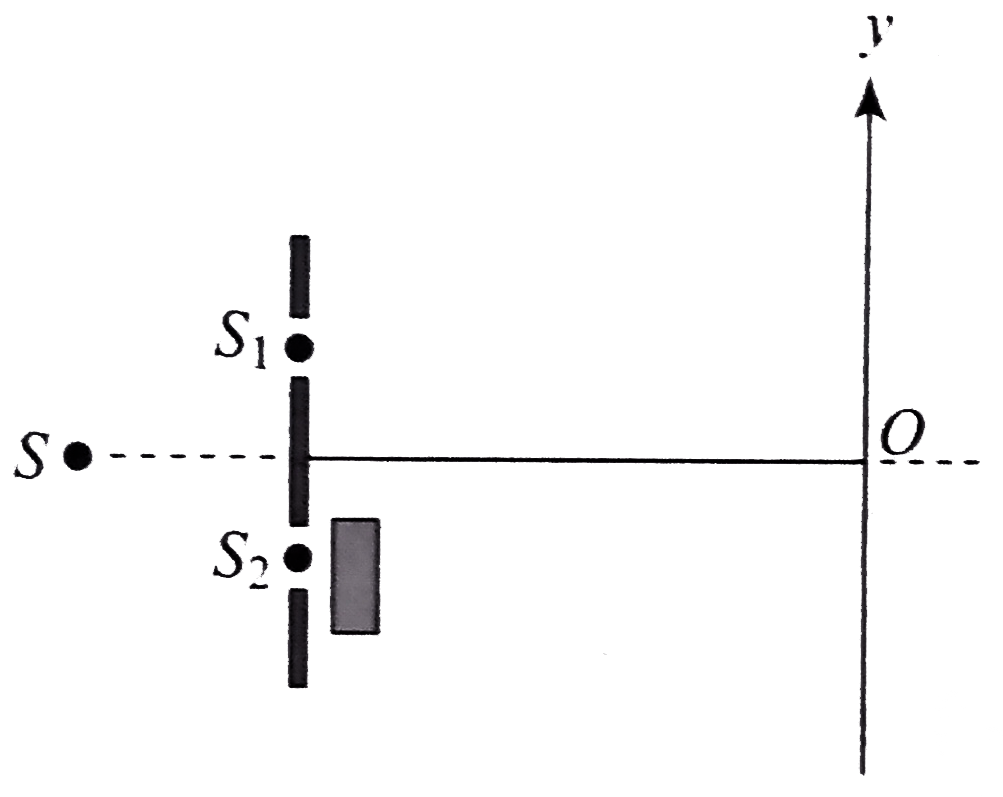
The location of the central maximum (bright fringe with zero path difference) on the y-axis will be
View SolutionA YDSE is performed in a medium of refractive index 4 // 3 , A light of 600 nm wavelength is falling on the slits having 0.45 nm separation . The lower slit S_(2) is covered b a thin glass plate of thickness 10.4 mm and refractive index 1.5. The interference pattern is observed on a screen placed 1.5 m from the slits as shown in figure. (All the wavelengths in this problem are for the given medium of refractive index 4 // 3 , ignore absorption.) Find the light intensity at point O relative t maximum fringe intensity.
View Solution_S01_025_Q01.png)
In Young's double slit experiment the slits, S1&S2 are illuminated by a parallel beam of light of wavelength 4000 Å from the medium of refractive index n1=1.2 A thin film of thickness 1.2 μm and refractive index n=1.5 is placed infrom of S1 perpedicular to path of light. the refractive index of medium between plane of slits & screen is n2=1.4 if the light coming from the film and S1&S2 have equal intensities I then intensity at geometrical centre of the screen O is
View SolutionIn a medium of refractive index n1, a monochromatic light of wavelength λ1 is travelling. When it enters in a denser medium of refractive index n2, the wavelength of the light in the second medium is
View Solutionएक double slit उपकरण की एक स्लिट पर n1 अपवर्तनांक तथा दूसरी स्लिट पर n2 अपवर्तनांक की बराबर मोटाई की फिल्मे चिपकाई गई है । प्रयोग में तरंगदैर्घ्य λ का प्रकाश स्लिटो पर लम्बवत पड़ता है । फिल्मो की न्यूनतम मोटाई बताएं जिसके लिए पैटर्न के केंद्र पर अदीप्त फ्रिंज बने ।
View SolutionLight of wavelength λ1 enters a medium with refractive index n2 from a medium with refractive index n1. What is the wavelength of light in second medium ?
View SolutionIn Young's double slit experiment the two slits are illuminated with light of wavelength λ .The thickness of a film of refractive index 1.4 to be introduced in the path of one of the interfering beams so that the central image shifts by one fringe width is
View SolutionIn Young's double slit experiment the point source is placed slightly off the central axis as shown in figure. The plane of slits (S1,S2) and screen are separated by a distance of 2m. The separation between the slits is 10 mm. The position of the slit above the axis is 1mm. if the wavelength λ=4000Å in the above arrangement

If central maximum is to occur at point 'P' then a sheet of thin film of refractive index μ=1.5 can be introduced in the path of rays from one the slits to the point 'P'. (t-thickness of film)
View SolutionThe young's double slit experiment is done in a medium of refractive index 4/3 . A light of 600 nm wavelength is falling on the slits having 0.45 mm separation. The lower slit S_(2) is covered by a thin glass sheet of thickness 10.4 mu m and refractive index 1.5. The interference pattern is observed on a screen placed. 1.5 m from the slit as shown in figure. Find the light intensity at point O relative to the maximum fringe intensity
View SolutionIn the figure shown in YDSE, a parallel beam of light is incident on the slit from a medium of refractive index n_1 . The wavelength of light in this medium is lambda_(1) . A transparent glass of thickness t and of refractive index n_(3) is put in front of one of the slits. The medium between the screen and the plane of the slits is n_(2) . The phase difference between the light waves reaching point .O. (symmetrically relative to the slit is
View Solution
