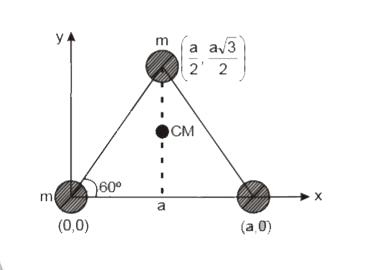
Three rods of the same mass are placed as shown in the figure. Calculate the coordinates of the centre of mass of the system.
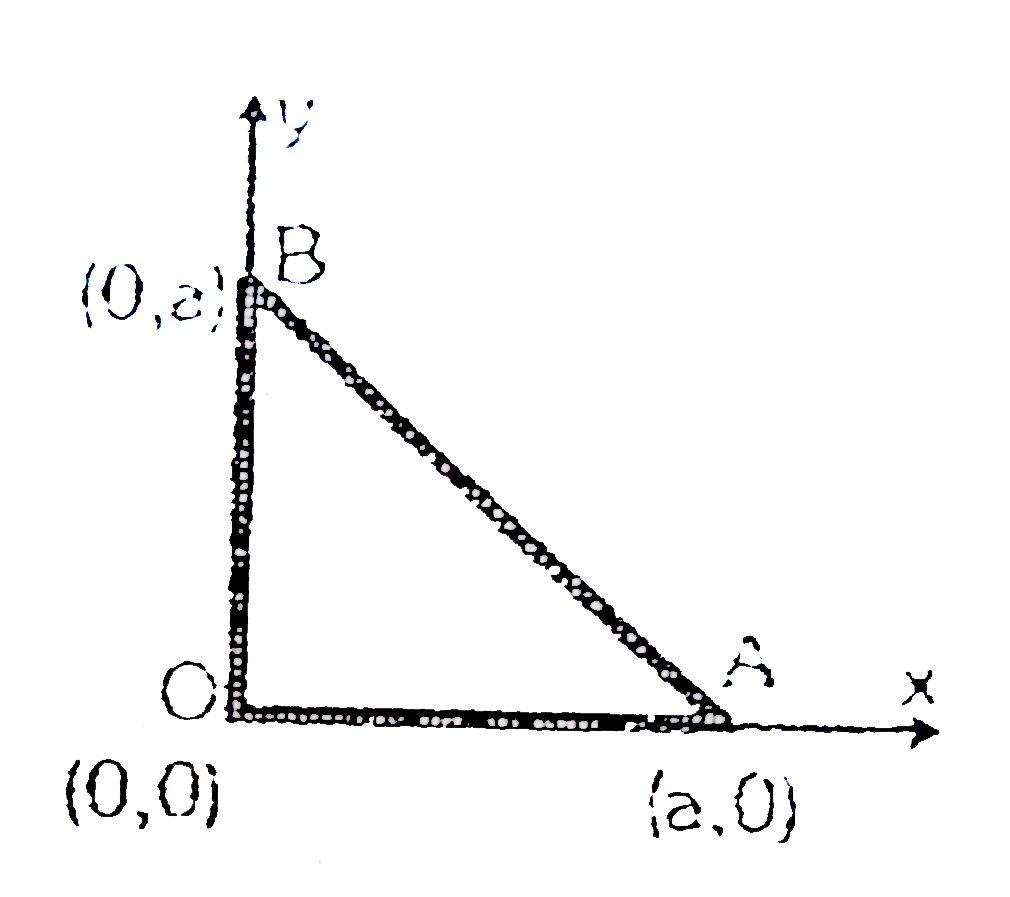 Solution in Gujrati
Solution in Gujrati
The correct Answer is:(a3,a3)
xcm=(M×a2)+(M×0)+(M×a2)M+M+M=a3
ycm=(M×0)+(M×a2)+(M×a2)M+M+M=a3
Topper's Solved these Questions
- CENTRE OF MASSBOOK - ALLEN CHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-IV B
14 Videos
- CENTRE OF MASSBOOK - ALLEN CHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V A
20 Videos
- CENTRE OF MASSBOOK - ALLEN CHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-III
41 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLEN CHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON
11 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)
3 Videos
Similar Questions
A bullet of mass M is fired with a velocity 50m/s at an angle with the horizontal. At the highest point of its trajectory, it collides head-on with a bob of mass 3M suspended by a massless string of length 10/3 metres and gets embeded in the bob. After the collision, the string moves through an angle of 120∘. Find
(i) the angle θ,
(ii) the vertical and horizontal coordinates of the initial position of the bob with respect to the point of firing of the bullet. Take g=10m/s2
View SolutionTwo identical particles move towards each other with velocity 2v and v respectively. The velocity of centre of mass is
View SolutionA particle of mass m, kinetic energy K and momentum p collision head on elastically with another particle of mass 2m at rest. After collision. :
Column IColumn II(A)Momentum of first particle(p)3/4p(B)Momentum of second particle(q)−K/9(C)Kinetic energy of first particle(r)−p/3(D)Kinetic energy of second particle(s)8K9None
View SolutionAssertion: If the initial and final positions coincide, the displacement is a null vector.
Reason: A physical quantity can not be called a vector, if its magnitude is zero.
View SolutionTwo soap bubbles A and B are kept in a closed chamber where the air is maintained at pressure 8N/m2.The radii of bubbles A and B are 2cm and 4cm, respectively. Surface tension of the soap-water used to make bubbles is 0.04N/m. Find tha ratio nB/nA, where nA and nB are the number of moles of air in bubbles A and B, respectively. [Neglect the effect of gravity.]
View SolutionA uniform thin rod is bent in the form of closed loop ABCDEFA as shown in the figure. The y- coordinate of the centre of mass of the system is
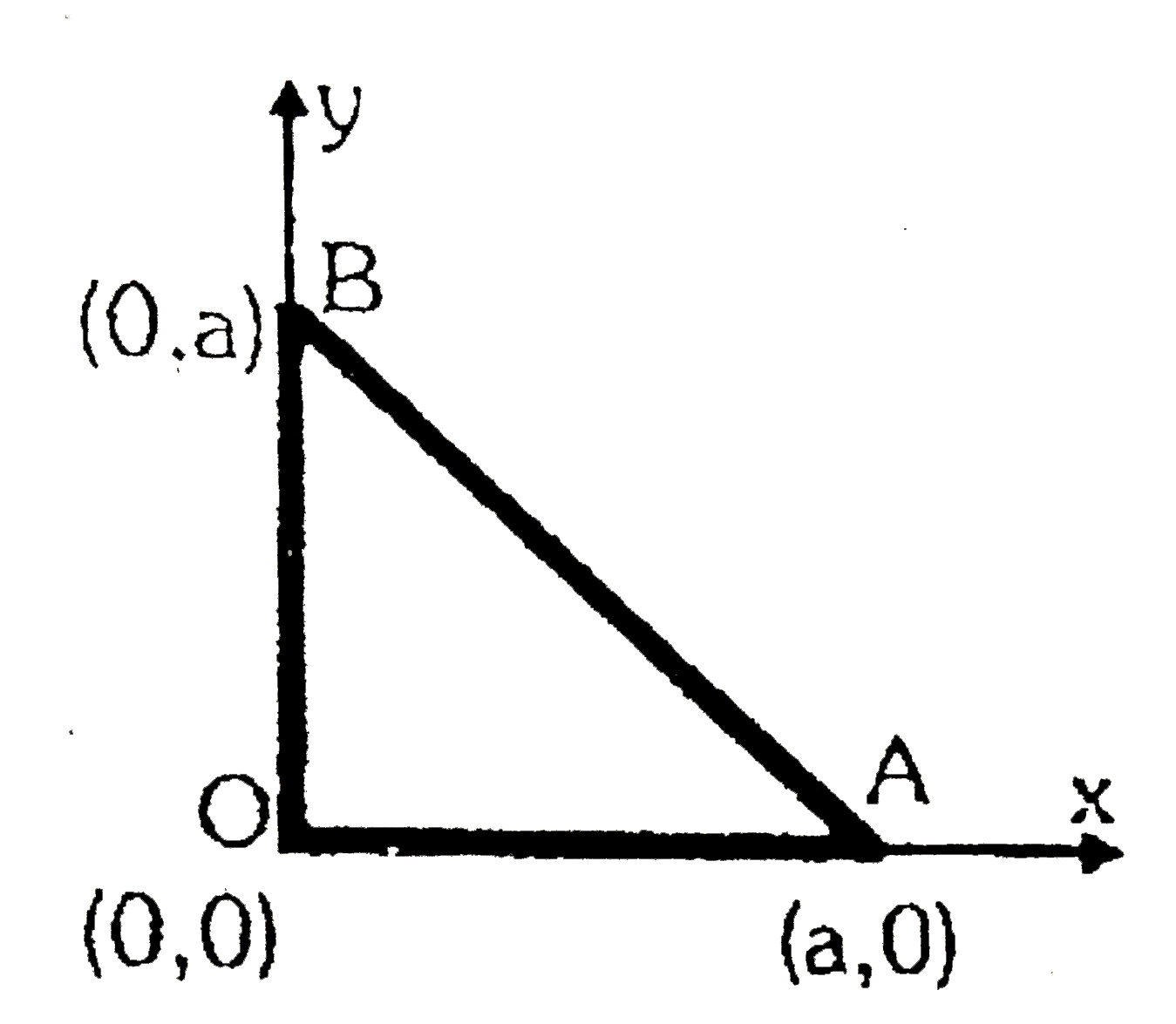
View SolutionThree rods of the same mass are placed as shown in the figure. Calculate the coordinates of the centre of mass of the system.
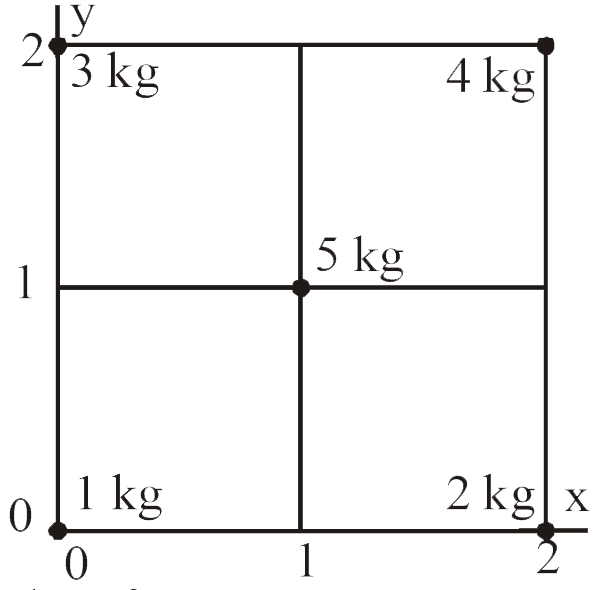
View SolutionFive masses are placed in a plane as shown in figure. The coordinates of the centre of mass are nearest to
View SolutionYou are supplied with three identical rods of same length and mass. If the length of each rod is 2pi . Two of them are converted into rings and then placed over the third rod as shown in figure. If point A is considered as origin of the coordinate system the coordinate of the center of mass will be (you may assume AB as x-axis of the coordinate system).
View SolutionA rod of length 2l is bent as shown in figure. Coordinates of centre of mass are
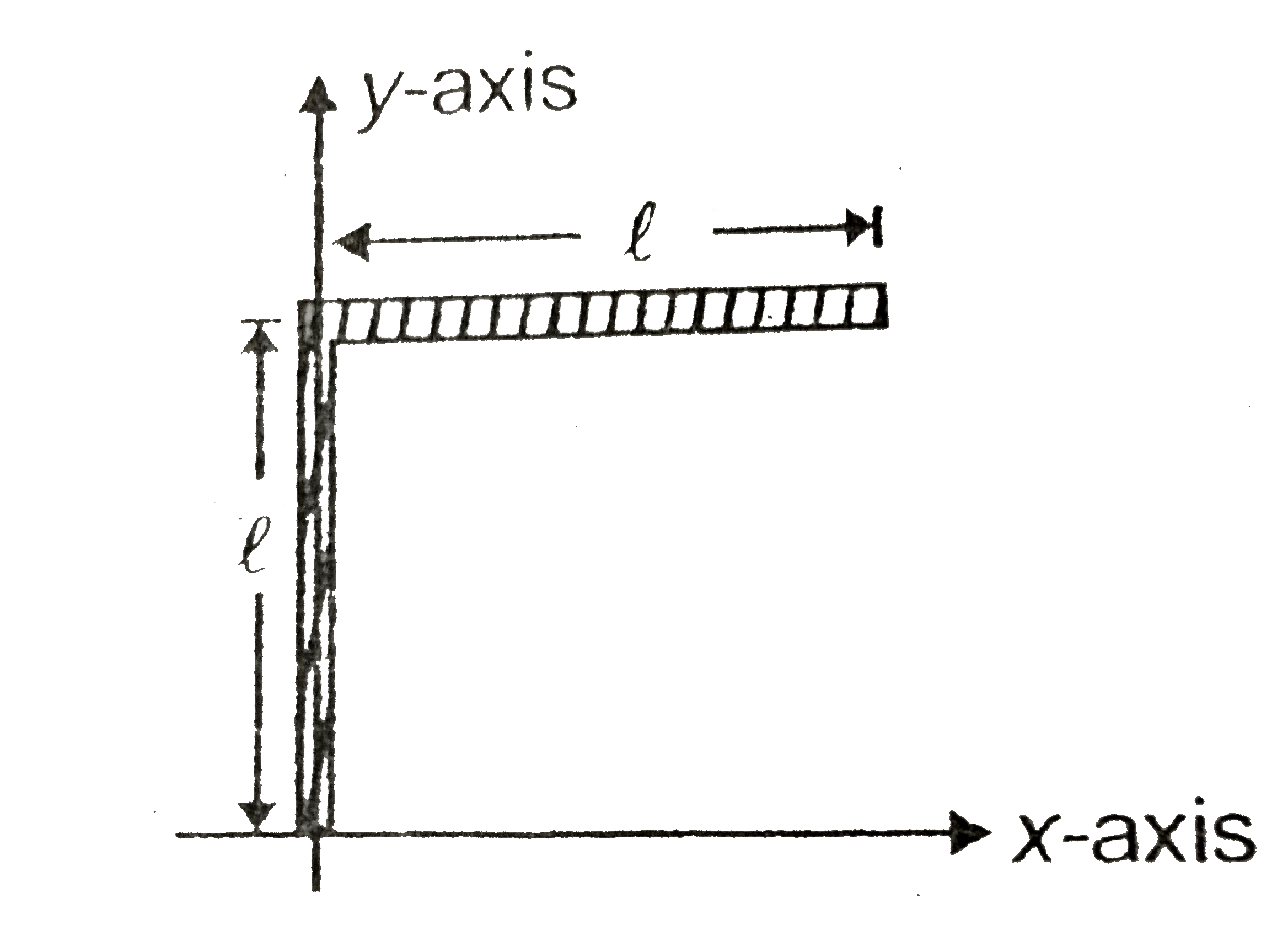
View SolutionThe coordinate of the centre of mass of a system as shown in figure : -
View SolutionThe coordinate of the centre of mass of a system as shown in figure : -
View SolutionSix rods of the same mass m and length ? are arranged as shown in figure. Calculate the coordinate of the centre of mass of the system:- У >X
View SolutionThree rods of the same mass are placed as shown in the figure. What will be the co-ordinates of the cneter of mass of the system ?

View SolutionIf three rods of same mass are placed as shown in the figure, the co-ordinates of the centre of mass of the system are
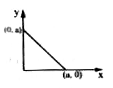
View SolutionThree rods of the same mass are placed as shown in the figure. Calculate the coordinates of the centre of mass of the system.

View Solution
