In figure, determine the character of the collision. The masses of the blocks, and the velocities before and after are given. The collision is
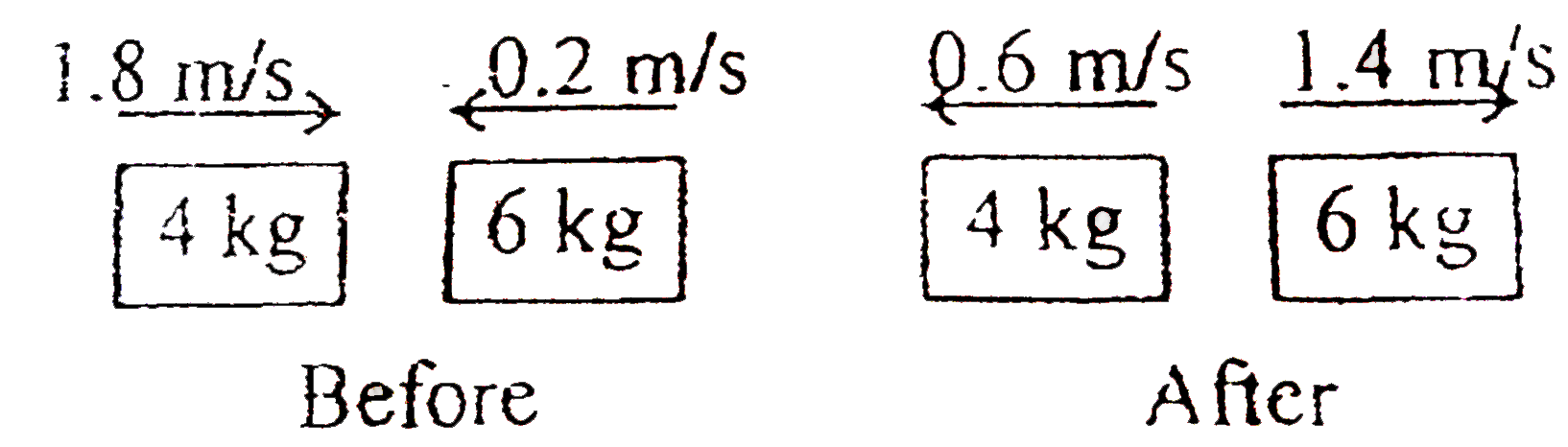 Solution in Gujrati
Solution in Gujrati
perfectly elastic
Partially inelastic
Completely inelastic
This collision is not possible
The correct Answer is:A
Ans.(1)
Linear momentum is conserverd and
e=Speed of separationSpeed of approach=1.4+0.61.8+0.2=1
So, collision of perfectly elastic.
Topper's Solved these Questions
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
- TEST PAPER 1BOOK - ALLEN CHAPTER - TEST PAPER 1EXERCISE - PHYSICS
11 Videos
Similar Questions
Choose the correct statement:
View SolutionChoose the correct statement:
View SolutionA rod with rectangular cross section oscillates about a horizontal axis passing through one of its ends and it behaves like a seconds pendulum, its length will be
View SolutionWhy are electric field lines perpendicular at a point on an equipotential surface of a conductor ?
View SolutionFind the final velocities of the masses after in collision the given situations.

View SolutionIn a one-dimensional collision between two particles, their relative velocity is vecv_(1) before the collision and vecv_(2) after the collision
View SolutionA block of mass 5 kg moves from left to right with a velocity of 2ms^(-1) and collides with another block of mass 3 kg moving along the same line in the opposite direction with velocity 4 ms^(-1) . (i) If the collision is perfectly elastic, determine velocities of both the blocks after their collision. (ii) If coefficient of restitution is 0.6, determine velocities of both the blocks after their collision.
View SolutionThe ratio of relative velocity of separation after collision to the relative velocity of approach before collision between two colliding bodies is ____________.
View SolutionDerive velocities after the collision in terms of velocities before collision in elastic collision in one dimension case.
View SolutionObtain the expression for the velocities of the two bodies after collision in the case of one dimensional elastic collision ond discuss the specical cases.
View Solution2m द्रव्यमान वाला एक गुटका जो अचर वेग 3→v से चल रहा है, एक अन्य गुटके से जिसका द्रव्यमान m है और जो विराम में है, से टकराता है | टक्कर के फलस्वरूप दोनों गुटके आपस में जुड़ जाते हैं | टक्कर के बाद जुड़े हुए गुटकों का वेग होगा
View SolutionIn one-dimensional elastic collision, the relative velocity of approach before collision is equal to
View SolutionShow that in a perfectly elastic collision , the relative velocity of seperation after collision is equal to relative velocity of approach before collision .
View SolutionShow that in case of one dimensional elastic collision of two bodies, the relative velocity' of sepa¬ ration after the collision is equal to the relative velocity of approach before the collision
View SolutionShow that in a perfectly elastic collision in one dimension, relative velocity after collision is equal to relative velocity before collision.
View SolutionThe ratio of relative velocity of separation after collision to the relative velocity of approach before collision between two colliding bodies is ____________.
View Solution
