Assertion :− velocity time graph of two paticles undergoing head−on collison is shown in the figure. If collision is inelastic then value of y must be less than x.
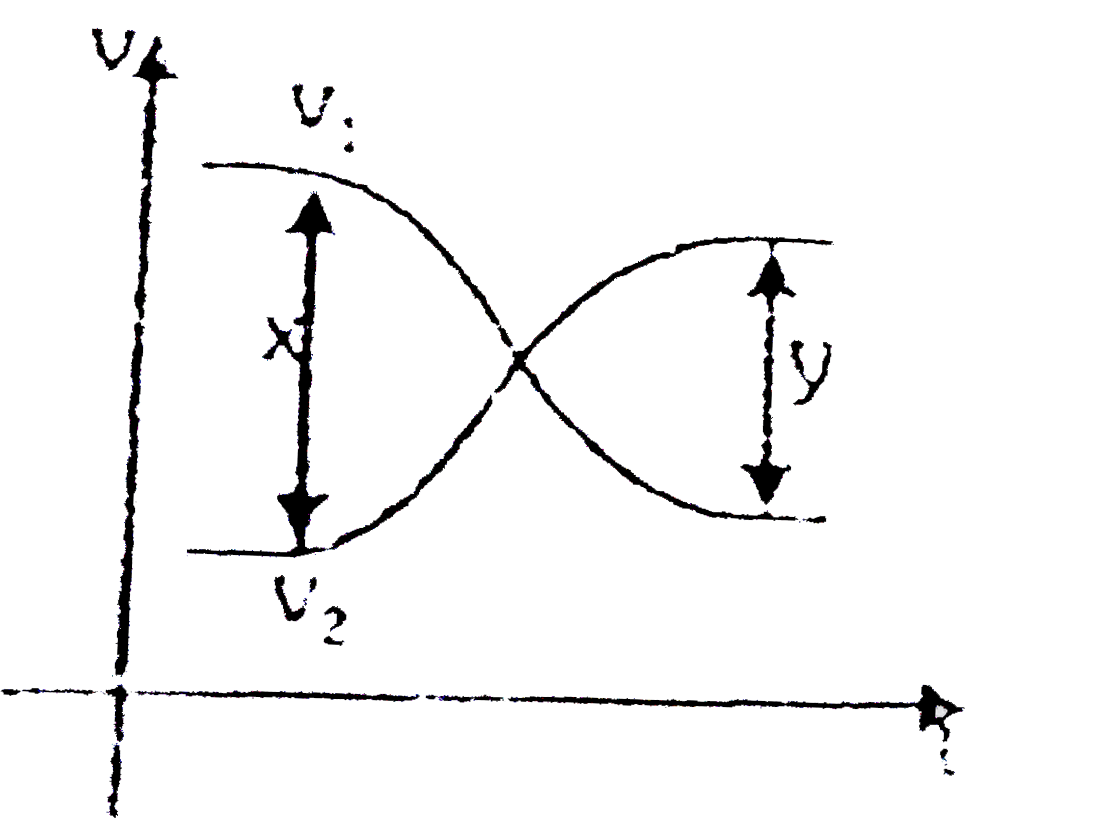
Reason :− Coefficient of restitution(e)=|velocity of separation||velocity of approach|Solution in Gujrati
If both Assertion & Reason are True & the Reason is a correct explanation of the Assertion.
If both Assertion & Reason are True but Reason is not a correct explanation of the Assertiion.
If Assertion is True but the Reason is False.
If both Assertion & Reason are False
The correct Answer is:A
Step by step video & image solution for Assertion :- velocity time graph of two paticles undergoing head-on collison is shown in the figure. If collision is inelastic then value of y must be less than x. Reason :- Coefficient of restitution(e)=(|"velocity of separation"|)/(|"velocity of approach"|) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS4videos
TEST PAPER
- BOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS4videos
TEST PAPER
- BOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example1videos
SIMPLE HARMONIC MOTION
- BOOK - ALLEN CHAPTER - TEST PAPER 1EXERCISE - PHYSICS11videos
TEST PAPER 1
Similar Questions
Choose the correct statement:
02:32View SolutionChoose the correct statement:
02:18View SolutionA rod with rectangular cross section oscillates about a horizontal axis passing through one of its ends and it behaves like a seconds pendulum, its length will be
01:48View SolutionWhy are electric field lines perpendicular at a point on an equipotential surface of a conductor ?
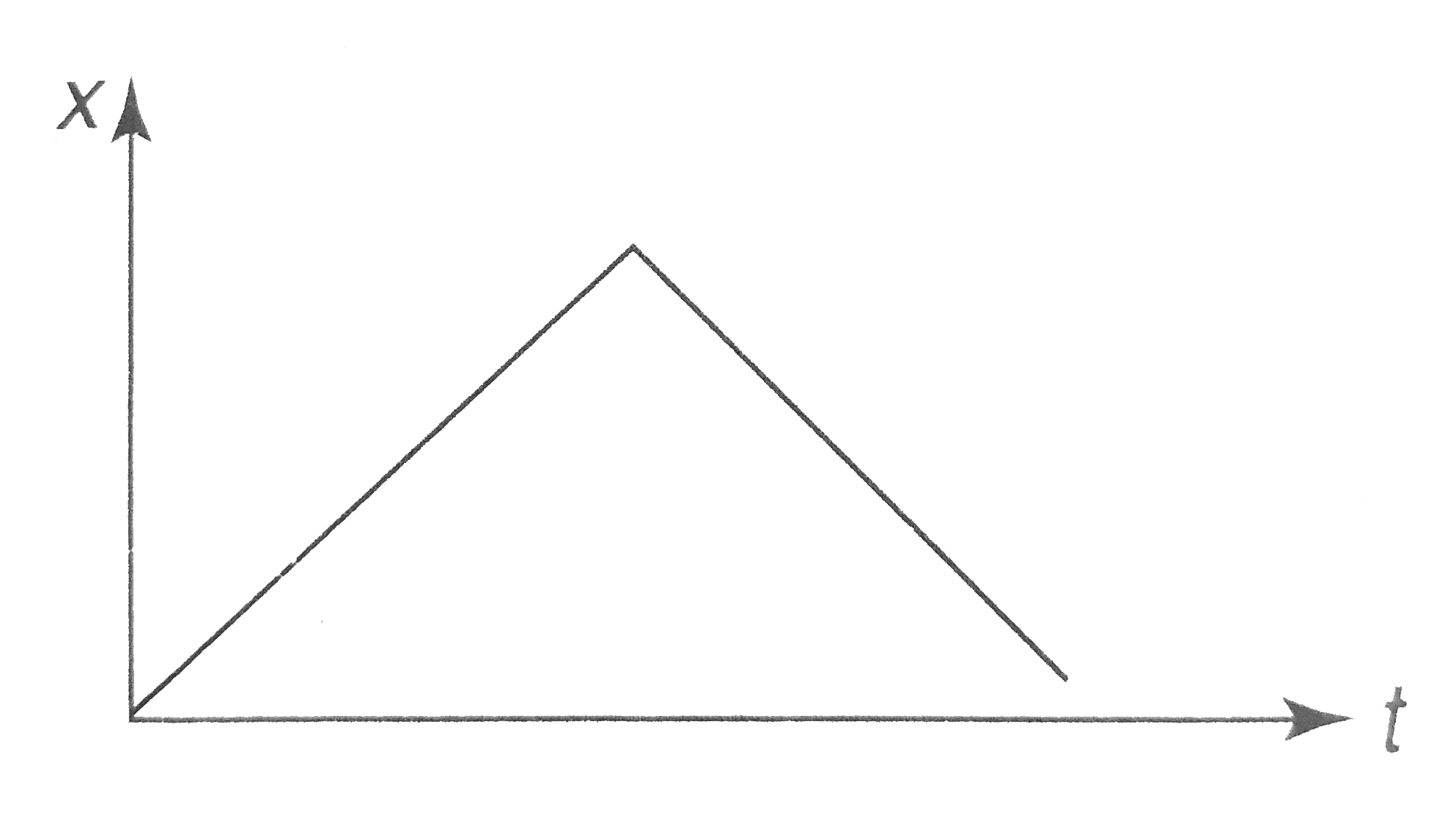
04:01View SolutionAssertion: x−t graph, for a particle undergoing rectilinear motion, can be as shown in the figure.
Reason: Infinitesimal changes in velocity are physically possible only in infinitesimal time.
 03:27View Solution
03:27View SolutionIn two separate collisions, the coefficient of restitutions e_(1) and e_(2) are in the ratio 3 : 1 . In the first collision the relative velocity of approach is twice the relative velocity of separation. Then, the ratio between relativevelocity of approach and relative velocity of separation in the second collision is
03:26View SolutionThese questions consists of two statements each printed as Assertion and Reason. While answering these question you are required to choose any one of the following five responses. Reason: For inelastic collision, 0leelt1 . Hence, the magnitude of relative velocity of separation after collision is less than relative velocity of approach before collision.
02:13View SolutionSTATEMENT-1 : In an elastic collision between two bodies, the relative velocity of separation equals relative velocity of approach. and STATEMENT-2 : In a elastic collision , the coefficient of restitution is 1 .
03:59View SolutionA sphere of mass 'm' moving with velocity 'v' collides head-on on another sphere of same mass which is at rest. The ratio of final velocity of second sphere to the initial velocity of the first sphere is (e is coefficient of restitution and collision is inelastic)
01:36View SolutionIn two separate collisions, the coefficient of restitutions d) A is false but B is true e and e are in the ratio 3: 1. In the first collision the relative velocity of approach is twice the relative velocity of separation, then the ratio between relative velocity of approach and the relative velocity of separation in the second collision is,
View SolutionVelocity of the ball A after collision with the ball B as shown in the figure is (Assume perfectly inelastic and head - on collision)
02:06View SolutionIf the velocity of separation is equal to the velocity of approach , then the collision is
04:24View SolutionFor complete inelastic collision, restitution coefficient e = …...
03:23View SolutionIn two separate collisions, the coefficients of retitutions, 'e1' are in the ratio 3:1. In the first collision, the relative velocity of approach is twice the relative velocity of separation. Then the ratio between the relative velocity of approach velocity of separation in the second collision is
08:08View SolutionThe coefficient of restitution e for a perfectly elastic collision is
01:06View SolutionA sphere of mass 'm' moving with velocity 'v' collides head-on on another sphere of same mass which is at rest. The ratio of final velocity of second sphere to the initial velocity of the first sphere is (e is coefficient of restitution and collision is inelastic)
01:44View Solution

