The distance x covered in time t by a body having initial velocity v0 and havig a constant acceleration a is given by x=v0t+(12)at2. This result follows from:-Solution in Gujrati
Newton's first law
Newton's second law
Newtons' third law
None of these three
The correct Answer is:B
F=Ma
a=∂2x∂t2
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - ALLEN CHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLEN CHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-I
52 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLEN CHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (JA)
4 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
25 Videos
Similar Questions
Assertion: The driver in a vechicle moving with a constant speed on a straight road is in a non-inertial frame of referance.
Reason: A reference frame in which Newton's laws of motion are applicable is non-inertial.
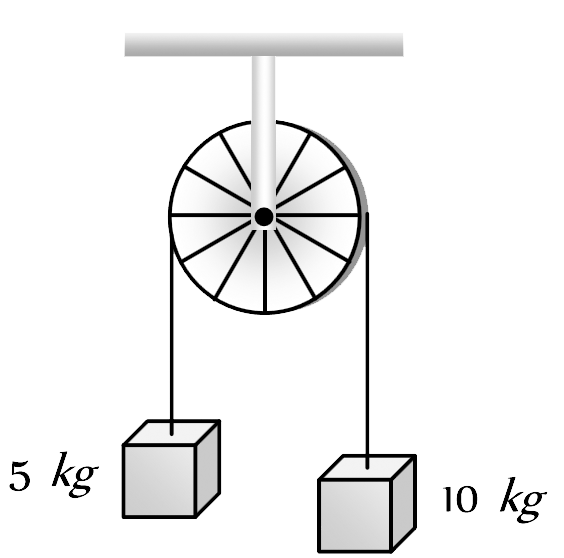
View SolutionUSS 150) Two masses of 5 kg and 10 kg are connected to a pulley as shown. What will be the acceleration of the system (g= acceleration due to gravity)
View SolutionA piece of wire is bent in the shape of a parabola y=kx2 (y-axis vertical) with a bead of mass m on it. The bead can slide on the wire without friction. It stays at the lowest point of the parabola when the wire is at rest. The wire is now accelerated parallel to the x-axis with a constant acceleration a. The distance of the new equilibrium position of the bead, where the bead can stay at rest with respect to the wire, from the y-axis is:
View SolutionThe frequency of a radar is 780 MHz. After getting reflected from an approaching aeroplane, the apparent frequency is more than the actual frequency by 2.6 kHz. The aeroplane has a speed of
View SolutionThe velocity of a particle is given by v=v_(0) sin omegat , where v_(0) is constant and omega=2pi//T . Find the average velocity in time interval t=0 to t=T//2.
View SolutionThe position of a particle at time t, is given by the equation, x(t) = (v_(0))/(alpha)(1-e^(-alpha t)) , where v_(0) is a constant and alpha gt 0 . The dimensions of v_(0) & alpha are respectively.
View SolutionThe distance x covered in time t by a body having initial velocity v0 and havig a constant acceleration a is given by x=v0t+(12)at2. This result follows from:-
View SolutionThe distance x covered in time t by a body having initial velocity v0 and havig a constant acceleration a is given by x=v0t+(12)at2. This result follows from:-
View SolutionThe position of a particle at time t is given by the equation x(t) = V_(0)/A (1-e^(At)) V_(0) = constant and A > 0 Dimensions of V_(0) and A respectively are
View Solutionकोई पिंड प्रारम्भिक वेग v से गतिमान है। उसका त्वरण a समय t के अनुक्रमानुपाती है । समय t पश्चात पिंड का वेग क्या होगा ?
View Solutionसमय t पर, त्वरण f से x-अक्ष पर गति करते कण का समीकरण f=f0(1−1T) है। जहाँ f0 तथ T नियतांक हैं। समय t = 0 पर कण विरामावस्था में है। समयान्तर, t = 0 तथा वह क्षण, जब f = 0 है, तब मध्य कण का वेग vx है
View SolutionThe position x of a particle at time t is given by : x=(v_(0))/(a)(1-e^(-at)) where v_(0) is a constant and a>0. The dimensional formula of v_(0) and a is :
View SolutionThe position x of a particle at time t is given by x=V0a(1−e−at), where V0 is constant and a>0. The dimensions of V0 and a are
View SolutionThe position of a particle at time t is given by the equation X(t)=(V_(0))/(A)(1-e^(At)) V_(0) = constant and A gt 0
View SolutionThe acceleration of a particle is increasing linearly with time t as bt.The particle starts from origin with an initial velocity V_(0) .The distance travelled by the particle in time t will be
View SolutionThe position x of a particle at time t is given by x=V0a(1−e−at), where V0 is constant and a>0. The dimensions of V0 and a are
View Solution
