Solids and liquids both expands on heating. The density of substance decreases on expanding according to the relation ρ2=ρ11+γ(T2−T1), where , ρ1→density atT1,ρ2→density atT2,γ→ coefficient of volume expansion of substances. When a solid is submerged in a liquid , liquid exerts an upward force on solid which is equal to the weight of liquid displaced by submerged part of solid. Solid will float or sink depends on relative densities of solid and liquid . A cubical block of solid floats in a liquid with half ot its volume submerged in liquid as shown in figure (at temperature T) αS→ Coefficient of linear expansion of solid
γL→Coefficient of volume expansion of liquid
ρS→Density of solid at temperatureT
ρL→ Density of liquid at temperatureT
_E01_198_Q01.png)
If temperature of system increases, then fraction of solid submerged in liquid Solution in Gujrati
increase
decrease
remains the same
inadequate information
The correct Answer is:D
If γliq>γsolid Also ρLVg=Mg
As T↑,ρL↓,so V displaced↑
Fraction of solid submerged should increased.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - ALLEN CHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE - 04 (A)
48 Videos
- GEOMETRICAL OPTICSBOOK - ALLEN CHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE - 04 (B)
15 Videos
- GEOMETRICAL OPTICSBOOK - ALLEN CHAPTER - GEOMETRICAL OPTICSEXERCISE - ASSERTION-REASON
40 Videos
- CURRENT ELECTRICITYBOOK - ALLEN CHAPTER - CURRENT ELECTRICITYEXERCISE - EX.II
66 Videos
- GRAVITATIONBOOK - ALLEN CHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
Similar Questions
Two rods each of length L2 and coefficient of linear expansion α2 each are connected freely to a third rod of length L1 and coefficient of expansion α1 to form an isoscles triangle. The arrangement is supported on a knife-edge at the midpoint of L1 which is horizontal. what relation must exist between L1 and L2 so that the apex of the isoscles triangle is to remain at a constant height from the knife edge as the temperature changes ?
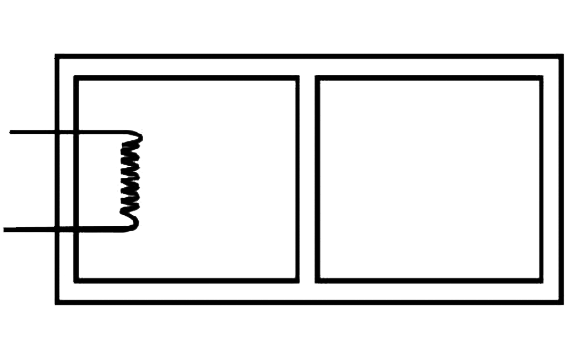
View SolutionThe rectangular box shown in Fig has partition which can slide without friction along the length of the box. Initially each of the two chambers of the box has one mole of a mono-atomic ideal gas (λ=5/3) at a pressure P0, volume V0 and temperature T0. The chamber on the left is slowly heated by an electric heater. The walls of the box and the lead wires of the heater is negligible. The gas in the left chamber expands pushing the partition until the final pressure in both chambers becomes 243P0/32. Determine (i) the final temperature of the gas in each chamber and (ii) the work done by the gas in the right chamber.
View SolutionStatement-1: A real gas behaves as an ideal gas at high temperature and low pressure .
Statement-2: Liquid state of an ideal gas is impossible.
View SolutionIn the Bohr's model of hy drogen atom, the electron moves around the nucleus in a circular orbit of radius 5×10−11m. Its time period is 1.5×10−16s. The current associated with the electron motion is:-
View SolutionAssertion : The difference in the value of acceleration due to gravity at pole and equator is proportional to square of angular velocity of earth.
Reason : the value of acceleration due to gravity is minimum at the equator and maximum at the pole.
View SolutionA block of material has a density rho_(1) and floats three-fourth submerged in a liquid of unknown density. Show tht the density rho_(2) of the unknown liquid is given by rho_(2)=(4)/(3)rho_(1) .
View SolutionA body weighs W_(1) in a liquid of density rho_(1) and W_(2) in a liquid of density rho_(2) . What is the weight of body in a liquid of density rho_(3) ?
View SolutionA body of density rho floats with a volume V_(1) of its total volume V immersed in one liquid of density rho_(1) and with the remainder of volume V_(2) immersed in another liquid of density rho_(2)," ""where"" "rho_(1)gt rho_(2). Find the relative volumes immersed in two liquids.
View SolutionAssertion : A solid is floating in a liquid of density ρ1. When the solid melts its density becomes ρ2 in liquid state. If ρ1>ρ2 level of liquids will increase after melting.
Reason : In liquid state volume always increases after a solid melts.
View SolutionSolids and liquids both expands on heating. The density of substance decreases on expanding according to the relation ρ2=ρ11+γ(T2−T1), where , ρ1→density atT1,ρ2→density atT2,γ→ coefficient of volume expansion of substances. When a solid is submerged in a liquid , liquid exerts an upward force on solid which is equal to the weight of liquid displaced by submerged part of solid. Solid will float or sink depends on relative densities of solid and liquid . A cubical block of solid floats in a liquid with half ot its volume submerged in liquid as shown in figure (at temperature T) αS→ Coefficient of linear expansion of solid
γL→Coefficient of volume expansion of liquid
ρS→Density of solid at temperatureT
ρL→ Density of liquid at temperatureT
_E01_197_Q01.png)
The relation between densities of solid and liquid at temperature T is
View SolutionSolids and liquids both expands on heating. The density of substance decreases on expanding according to the relation ρ2=ρ11+γ(T2−T1), where , ρ1→density atT1,ρ2→density atT2,γ→ coefficient of volume expansion of substances. When a solid is submerged in a liquid , liquid exerts an upward force on solid which is equal to the weight of liquid displaced by submerged part of solid. Solid will float or sink depends on relative densities of solid and liquid . A cubical block of solid floats in a liquid with half ot its volume submerged in liquid as shown in figure (at temperature T) αS→ Coefficient of linear expansion of solid
γL→Coefficient of volume expansion of liquid
ρS→Density of solid at temperatureT
ρL→ Density of liquid at temperatureT
_E01_199_Q01.png)
Imagine fraction submerged does not change on increasing temperature the relation between γL and αS is
View SolutionSolids and liquids both expands on heating. The density of substance decreases on expanding according to the relation ρ2=ρ11+γ(T2−T1), where , ρ1→density atT1,ρ2→density atT2,γ→ coefficient of volume expansion of substances. When a solid is submerged in a liquid , liquid exerts an upward force on solid which is equal to the weight of liquid displaced by submerged part of solid. Solid will float or sink depends on relative densities of solid and liquid . A cubical block of solid floats in a liquid with half ot its volume submerged in liquid as shown in figure (at temperature T) αS→ Coefficient of linear expansion of solid
γL→Coefficient of volume expansion of liquid
ρS→Density of solid at temperatureT
ρL→ Density of liquid at temperatureT
_E01_200_Q01.png)
Imagine the depth of the block submerged in the liquid ,does not change on increasing temperature then
View SolutionSolids and liquids both expands on heating. The density of substance decreases on expanding according to the relation ρ2=ρ11+γ(T2−T1), where , ρ1→density atT1,ρ2→density atT2,γ→ coefficient of volume expansion of substances. When a solid is submerged in a liquid , liquid exerts an upward force on solid which is equal to the weight of liquid displaced by submerged part of solid. Solid will float or sink depends on relative densities of solid and liquid . A cubical block of solid floats in a liquid with half ot its volume submerged in liquid as shown in figure (at temperature T) αS→ Coefficient of linear expansion of solid
γL→Coefficient of volume expansion of liquid
ρS→Density of solid at temperatureT
ρL→ Density of liquid at temperatureT
_E01_201_Q01.png)
Assume block does not expand on heating . The temperature at which the block just begins to sink in liquid is
View SolutionA block of material has a density ρ1 and floats three-fourth submerged in a liquid of unknown density. Show tht the density ρ2 of the unknown liquid is given by ρ2=43ρ1.
View SolutionAssertion : A solid is floating in a liquid of density ρ1. When the solid melts its density becomes ρ2 in liquid state. If ρ1>ρ2 level of liquids will increase after melting.
Reason : In liquid state volume always increases after a solid melts.
View SolutionA body floats in a liquid A of density ρ1 with a part of it submerged inside liquid while in liquid B of density ρ2 totally submerged inside liquid. The densities ρ1 and ρ2 are related as :
View Solution
