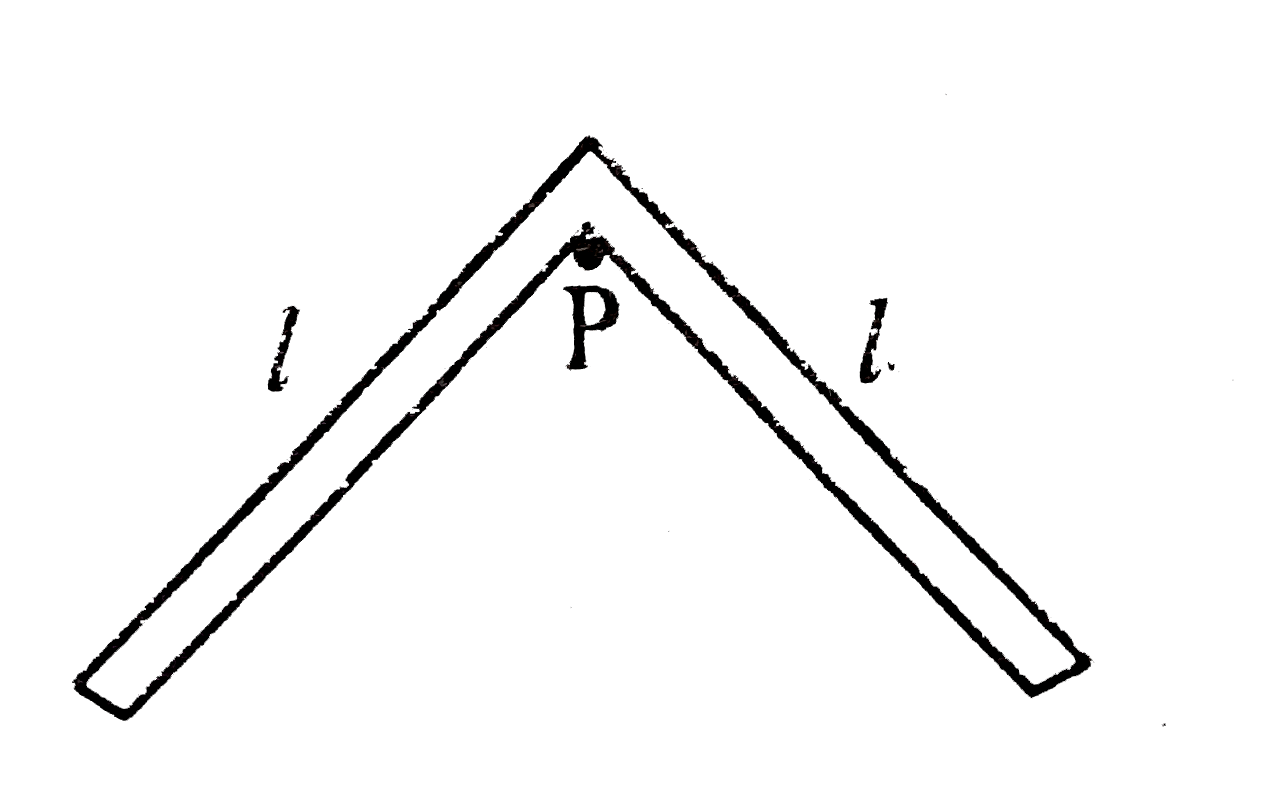
A system of two identical rods (L-shaped) of mass m and length l are resting on a peg P as shown in the figure. If the system is displaced in its plane by a small angle θ,find the period of oscillations:
 Solution in Gujrati
Solution in Gujrati
2π√√2l3g
2π√2√2l3g
2π√2l3g
2π√l3g
The correct Answer is:B
Center of mass 2m of a system is at a distance peg P is l2√2
_E01_027_S01.png)
τ=r×F=−mgsinθl2√2
⇒Iα=−mgsinθ=l2√2(sinθ=0 from small θ)
⇒2ml23α=−mgθl2√2⇒α=−3gθ2s√2l=−ω2θ
⇒ω=√3g2√2l⇒T=2πω=2π√2√2l3g
Topper's Solved these Questions
- BOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-0219videos
SIMPLE HARMONIC MOTION
- BOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise- 3 Match The Column1videos
SIMPLE HARMONIC MOTION
- BOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - SOME WORKED OUT EXAMPLES29videos
SIMPLE HARMONIC MOTION
- BOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)25videos
RACE
- BOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS4videos
TEST PAPER
Similar Questions
A mass M is performing linear simple harmonic motion. Then correct graph for acceleration a and corresponding linear velocity v is
06:38View SolutionColumn-I gives different situtions in which bodes are floating in liquid. Column-II gives correspoding time periods of oscillation.
_E01_069_Q01.png)
A cube floating in liquid.03:34View SolutionA simple pendulum has time period 2s. The point of suspension is now moved upward accoding to relation y=(6t−3.75t2)m where t is in second and y is the vertical displacement in upward direction. The new time period of simple pendulum will be
02:58View SolutionThe frequency of a radar is 780 MHz. After getting reflected from an approaching aeroplane, the apparent frequency is more than the actual frequency by 2.6 kHz. The aeroplane has a speed of
03:36View SolutionChoose the correct statement:
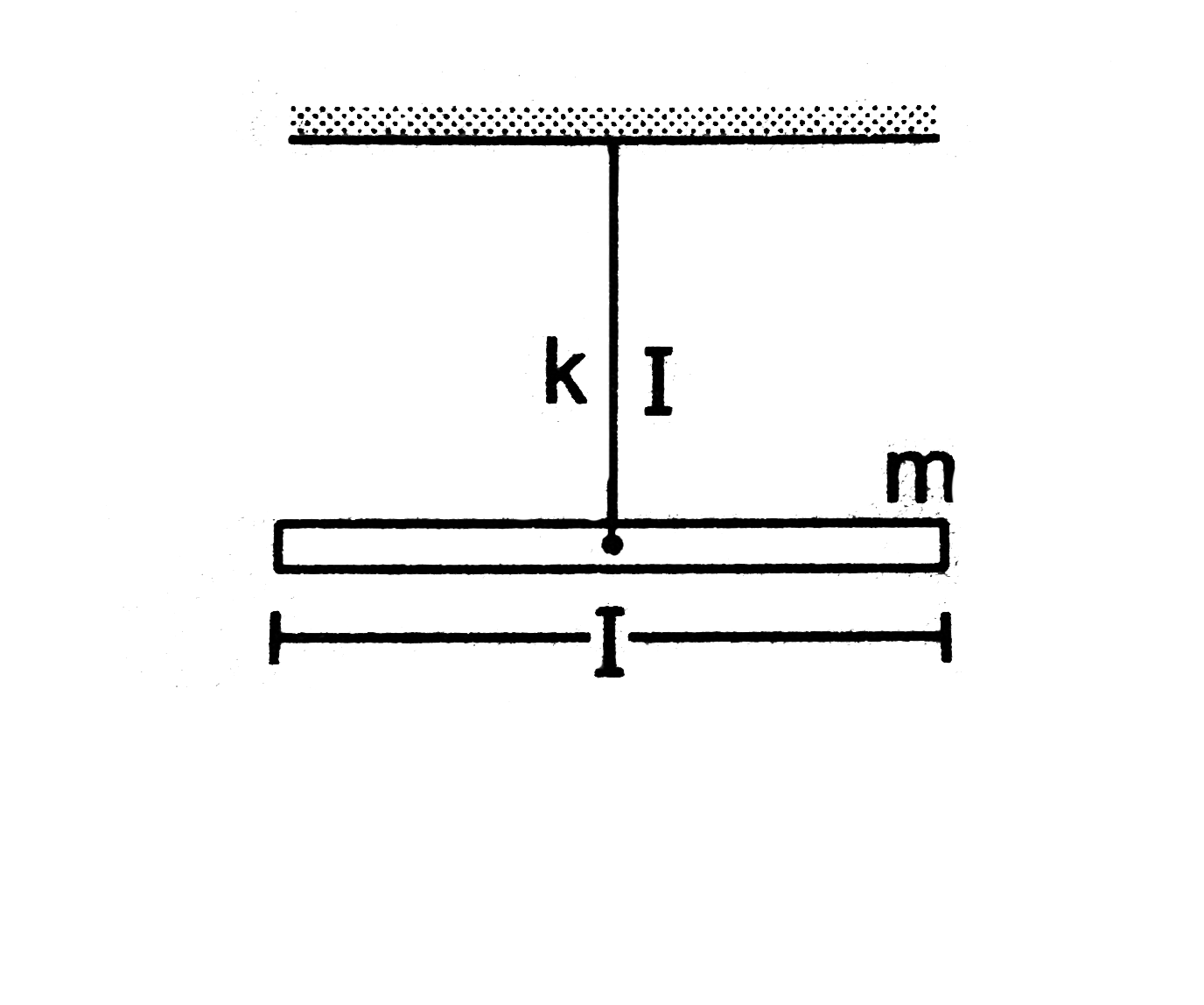
View SolutionA uniform rod of mass m and length l is suspended through a light wire of length l and torsional constant k as shown in figure. Find the time perid iof the system makes a. small oscillations in the vertical plane about the suspension point and b. angular oscillations in the horizontal plane about the centre of the rod.
 04:54View Solution
04:54View SolutionTwo identical ladders, each of mass M and length L are resting on the rough horizontal surface as shown in the figure. A block of mass m hangs from P. if the system is in equilibrium, find the magnitude and the direction of frictional force at A and B.
01:53View SolutionTwo identacal rods each of length l and mass m weided toeather at right angle and edge suspended from a kinetic sides as shown Angular frequency of small oscillation of the system in its then plane about the total of suspension is
09:18View SolutionA uniform rod of length L and mass M is pivotedat the centre. Its two ends are attached to two springs of equal spring constants. k. The springs as shown in the figure, and the rod is free to oscillate in hte horizontal plane. the rod is gently pushed through a small angle θ in one direction and released. the frequency of oscilllation is-
 06:59View Solution
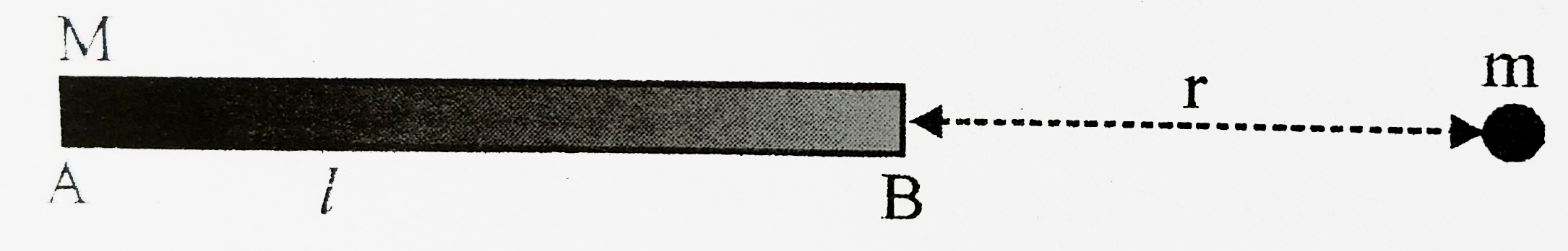
06:59View SolutionFind the gravitational potential energy of system consisting of uniform rod AB of mass M, length l and a point mass m as shown in figure.
 08:17View Solution
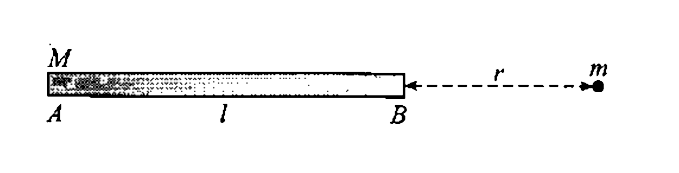
08:17View SolutionFind the gravitational potential energy of a system consisting of a uniform rod AB of mass M, length l and a point mass m as shown in figure.
 04:07View Solution
04:07View SolutionTwo uniform thin rods each of length L and mass m are joined as shown in the figure. Find the distance of centre of mass the system from point O
 01:58View Solution
01:58View Solutionचित्र 22.5 में द्रव्यमान m एवं लम्बाई l वाली एक समरूप छड़ को लम्बाई l वाले पतले तथा नगण्य भार वाले तार द्वारा लटकाया गया है। तार का मरोड़ी नियतांक k है। आवर्तकाल ज्ञात कीजिए यदि (a) छड़ ऊर्ध्वाधर तल में निलंबन-बिंदु के पारित: छोटे दोलन करती है (b) छड़ क्षैतिज तल में अपने केंद्र के पारित: छोटे दोलन करती है।
 06:57View Solution
06:57View SolutionAs shown in diagram there are five identical rods. Length of each rod is l and mass m. Find out distance of C.O.M. of system From (C)
 02:39View Solution
02:39View SolutionTwo identical ladders, each of mass M and length L are resting on the rough horizontal surface as shown in the figure. A block of mass m hangs from P. If the system is in equilibrium, find the magnitude and the direction of frictional force at A and B.
01:54View SolutionA uniform rod of mass m and length l is suspended about its end. Time period of small angular oscillations is
 08:56View Solution
08:56View Solution

