A simple pendulum of length L is constructed form a point object of mass m suspended by a massless tring attached to a fixed pivot point. A small peg is placed a distance 2L/3 directly below the fixed pivot point. A small peg is placed a distance 2L/3 directly below the fixed pivot point so that the pendulum would wing as shown in the figure. the mass is displaced 5 degrees form the vertical and released. How does it take to return to its starting position.
_E01_024_Q01.png) Solution in Gujrati
Solution in Gujrati
π√Lg(1+√23)
π√Lg(2+√23)
π√Lg(1+13)
π√Lg(1+13)
The correct Answer is:D
T∝√L
t=π√Lg+π√L/3g
Topper's Solved these Questions
- BOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-0219videos
SIMPLE HARMONIC MOTION
- BOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise- 3 Match The Column1videos
SIMPLE HARMONIC MOTION
- BOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - SOME WORKED OUT EXAMPLES29videos
SIMPLE HARMONIC MOTION
- BOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)25videos
RACE
- BOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS4videos
TEST PAPER
Similar Questions
A mass M is performing linear simple harmonic motion. Then correct graph for acceleration a and corresponding linear velocity v is
02:27View SolutionColumn-I gives different situtions in which bodes are floating in liquid. Column-II gives correspoding time periods of oscillation.
_E01_069_Q01.png)
A cube floating in liquid.02:58View SolutionA simple pendulum has time period 2s. The point of suspension is now moved upward accoding to relation y=(6t−3.75t2)m where t is in second and y is the vertical displacement in upward direction. The new time period of simple pendulum will be
04:16View SolutionThe frequency of a radar is 780 MHz. After getting reflected from an approaching aeroplane, the apparent frequency is more than the actual frequency by 2.6 kHz. The aeroplane has a speed of
03:20View SolutionChoose the correct statement:
03:29View SolutionA simple pendulum consisting of a mass M attached to a string of length L is released from rest at an angle alpha . A pin is located at a distance l below the pivot point. When the pendulum swings down, the string hits the pin as shown in figure. The maximum angle theta which the string makes with the vertical after hitting the pin is
06:07View SolutionParticle of masses m, 2m,3m,…,nm grams are placed on the same line at distance l,2l,3l,…..,nl cm from a fixed point. The distance of centre of mass of the particles from the fixed point in centimeters is :
View SolutionA simple pendulum of mass m and charge +q is suspended vertically by a massless thread of length l. At the point of suspension, a point charge +q is also fixed. If the pendulum is displaced slightly from equilibrium position, its time period will be
07:25View SolutionA mass is attached to the end of a string of length l which is tied to a fixed point O. The mass is released from the initial horizontal position of the string. Below the point O at what minimum distance a peg P should be fixed so that the mass turns about P and can describe a complete circle in the vertical plane?
View SolutionParticles of masses m, 2m, 3m ... nm gram are placed on the same line at distance l, 2l, 3l, ..., nl cm from a fixed point. The distance of centre of mass of the particles from the fixed point in cm is
03:29View SolutionA uniform disc of mass M and radius R is supported vertically by a pivot at its periphery as shown. A particle of mass M is fixed to the rim and raised to the highest point above the center. The system is released from rest and it can rotate about pivot freely. The angular speed of the system when the attached object is directly beneath the pivot, is
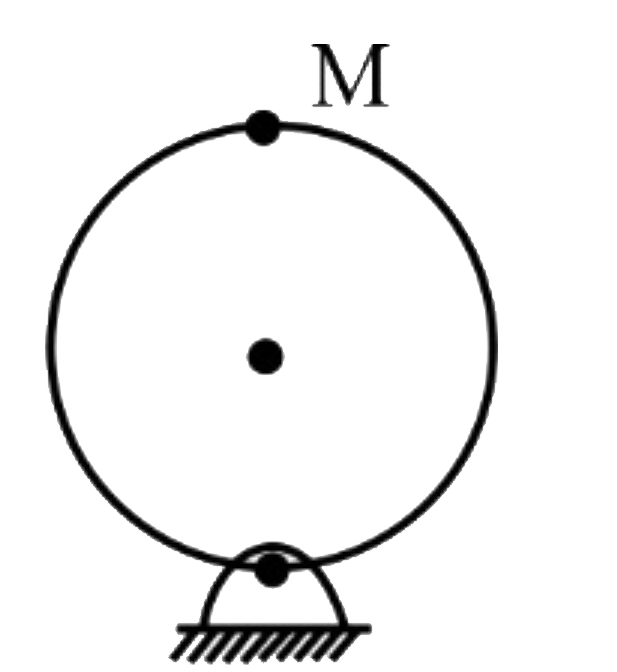 View Solution
View SolutionA pendulum is formed by pivoting a long thin rod of length L and mass m about a point P on the rod which is a distance d above the centre of the rod as shown. The time period of this pendulum when d = L/2 will be
03:29View SolutionA pendulum is formed by pivotting a long thin rod of length L and mass m about a point P on the rod which is a distance d above the centre of the rod as shown.

The time period of this pendulum when d = L/2 will be05:05View SolutionParticles of masses m, 2m, 3m, …….. nm grams are placed on the same line at distances l, 2l, 3l, …… nl cm from a fixed point. The distance of centre of mass of the particles from the fixed point in centimeter is
04:53View SolutionParticles of masses m, 2m, 3m, …….. Nm grams are placed on the same line at distances l, 2l, 3l, ……..nl cm from a fixed point. The distance of centre of mass of the particles from the fixed point in centimeter is
03:33View SolutionParticles of masses m, 2m, 3m, ... , nm grams are placed on the same line at distances l, 2l, 3l, ..., nl cm from a fixed point. The distance of centre of mass of the particles from the fixed point in centimetres is
05:03View Solution

