A block A of mass m is placed over a plank B of mass 2m. Plank B is placed over a smooth horizontal surface. The coefficient of friction between A and B is 12 block A is given a velocity v0 towards right. Accelration of B relative to A is
 Solution in Gujrati
Solution in Gujrati
g2
g.
3g4
zero
The correct Answer is:C
Step by step video & image solution for A block A of mass m is placed over a plank B of mass 2m. Plank B is placed over a smooth horizontal surface. The coefficient of friction between A and B is (1)/(2) block A is given a velocity v_(0) towards right. Accelration of B relative to A is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLEN CHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (JM)
6 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLEN CHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (JA)
4 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLEN CHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (O-1)
50 Videos
- MOTION IN A PALNEBOOK - ALLEN CHAPTER - MOTION IN A PALNEEXERCISE - SOLVED EXAMPLE
28 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLEN CHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
Similar Questions
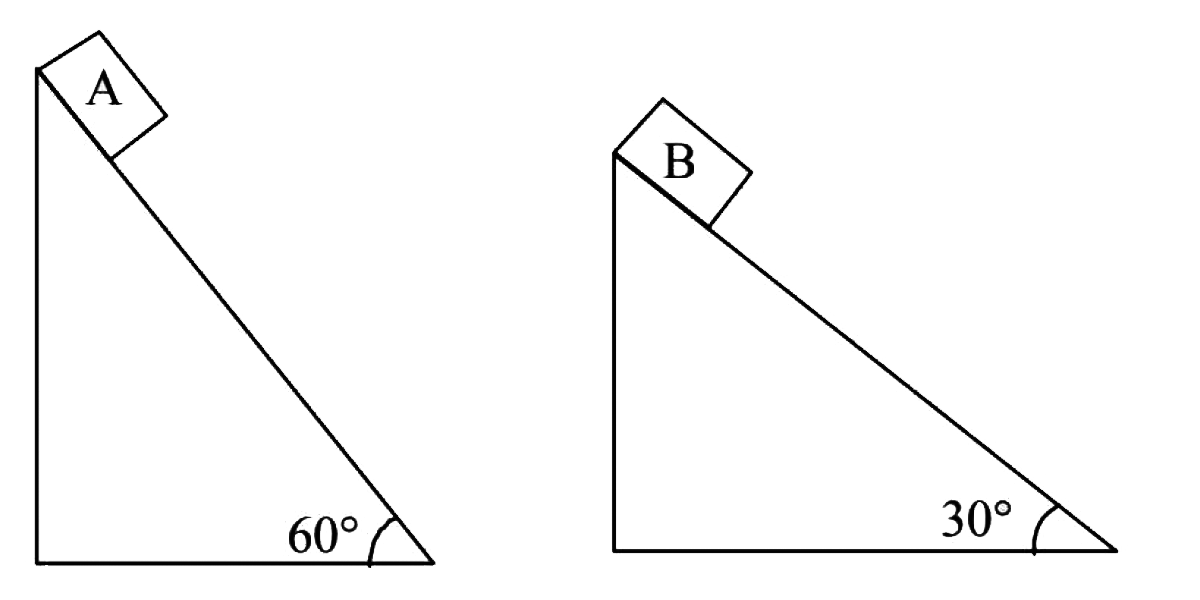
Two fixed frictionless inclined planes making an angle 30∘ and 60∘ with the vertical are shown in figure. Two blocks A and B are planes. What is the relative vertical acceleration of A with respect to B?
View SolutionA piece of wire is bent in the shape of a parabola y=kx2 (y-axis vertical) with a bead of mass m on it. The bead can slide on the wire without friction. It stays at the lowest point of the parabola when the wire is at rest. The wire is now accelerated parallel to the x-axis with a constant acceleration a. The distance of the new equilibrium position of the bead, where the bead can stay at rest with respect to the wire, from the y-axis is:
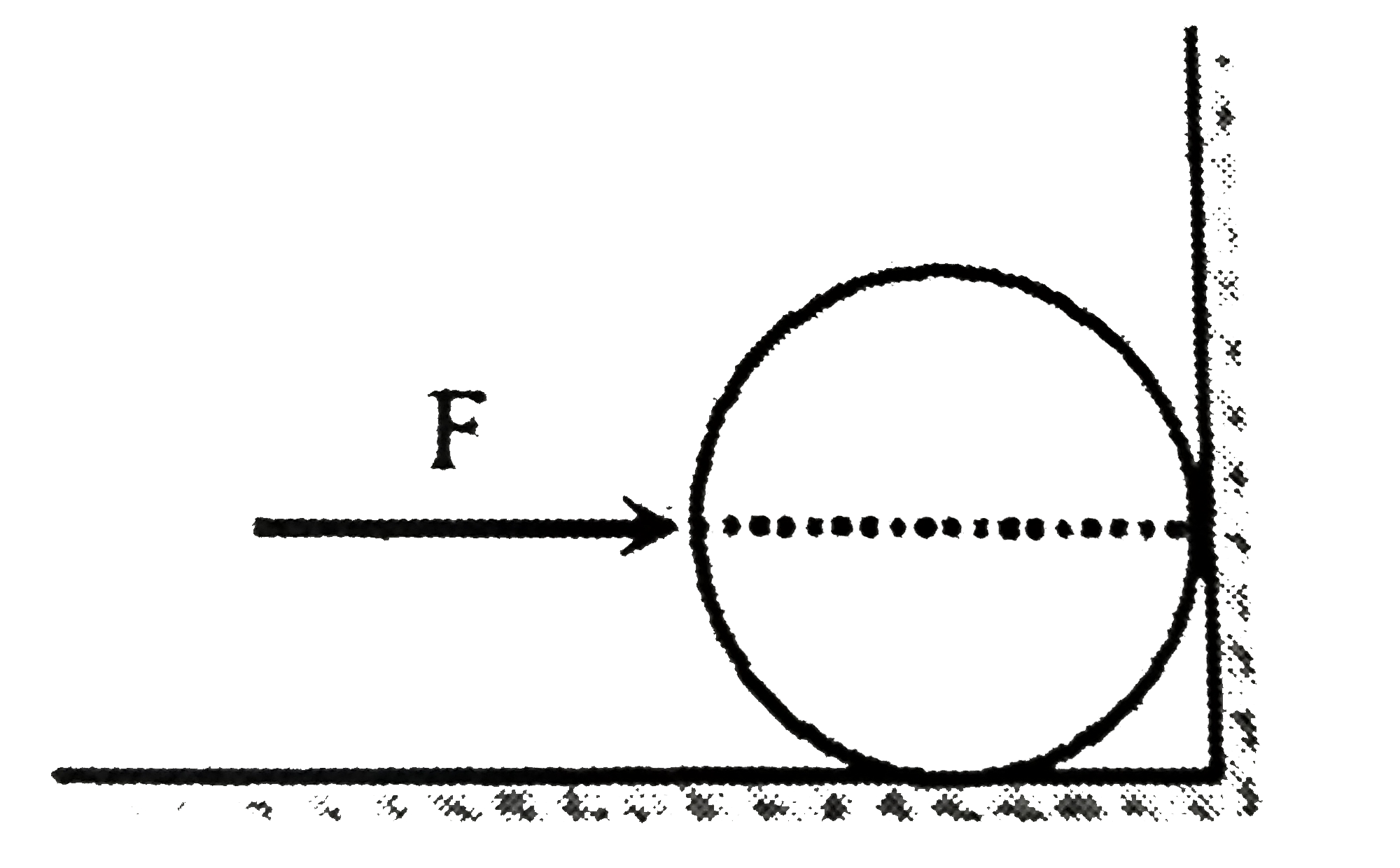
View SolutionA ball of mass m kept at the corner as shown in the figure, is acted by a horizontal force F. The correct free body diagram of ball is
View SolutionA particle thrown over a triangle from one end of a horizontal base falls on the other end of the base after grazing the vertex. If αandβ are the base angles of triangle and angle of projection is θ, then prove that (tanθ=tanα+tanβ)
View SolutionAssertion: The driver in a vechicle moving with a constant speed on a straight road is in a non-inertial frame of referance.
Reason: A reference frame in which Newton's laws of motion are applicable is non-inertial.
View SolutionFigure shown a small block A of mass m kept at the left end of a plank B of mass M = 2m and length l . The system can side on a horizontal rood. The system is started towards right with the initial velocity v . The friction coefficient between the rood and the plank is 1//2 and that between the plank and the block is 1//4 . Find (a) the time elapsed before the block separates from the plank (b) displacement of block and plank relative to ground till that moment.
View SolutionA block A of mass m is placed over a plank B of mass 2m plank B is placed over a smooth horizontal surface .The coefficient of friction between A and B is 0.4 Block A is given a velocity v_(0) toward right Find acceleration (in ms^(-2) of B relative to A
View SolutionA block A of mass m is over a plank B of mass 2m . Plank B si placed over a smooth horizontal surface. The coefficient of friction between A and B is (1)/(2) . Blocks A is given a velocity V_(0) towards right. Then
View SolutionA block A is placed over a long rough plank B same mass as shown below. The plank is placed over a smooth horizontal surface. At time t = 0 , block A is given a velocity v_(0) in horizontal direction. Let v_(1) and v_(2) be the velocity of A & B at time 't'. Then choose the correct graph between v_(1) or v_(2) and t.
View SolutionA block A of mass m is placed over a plank B of mass 2m. Plank B is placed over a smooth horizontal surface. The coefficient of friction between A and B is 12 block A is given a velocity v0 towards right. Accelration of B relative to A is

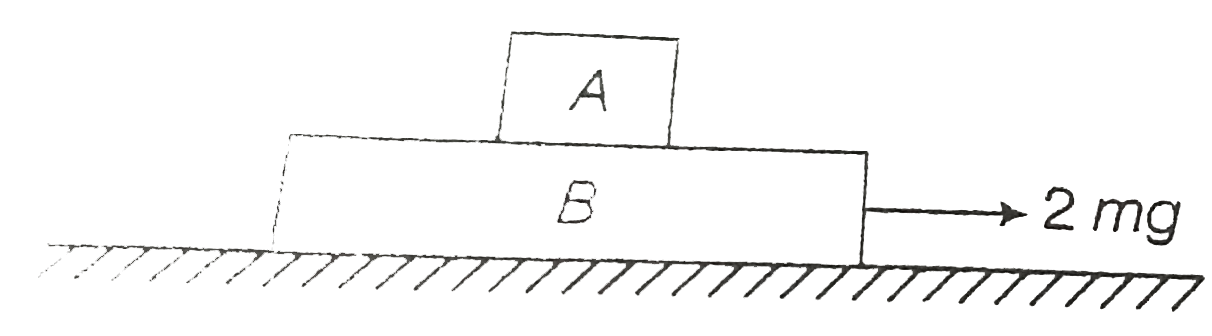
View SolutionBlock of mass m rests on the plank B of mass 3m which is free to slide on a frictionless horizontal surface. The coefficient of friction between the block and plank is 0.2. If a horizontal force of magnitude 2 mg is applied to the plank B, the acceleration of A relative to the plank and relative to the ground respectively, are
View SolutionA block of mass m is gently placed over a massive plank moving horizontal over a smooth surface with velocity 10ms−1. The coefficient of friction between the block and the plank is 0.2. The distance travelled by the block till it slides on the plank is [g=10ms−2]
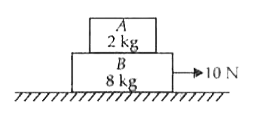
View SolutionBlock A of mass 2 kg is placed over block B of mass 8 kg .The combination is placed over a rough horizonatal surface .Cofficient of friction between B and the floor is 0.5 .Coefficient of friction berween A and B is 0.4 .A horizontal force of 10 N is applied on block B .The force of friction between A and B is
(g=10 ms−2)
View SolutionBlock A of mass m rests on the plank B of mass 3m which is free to slide on a frictionless horizo-ntal surface. The coefficient of friction between the block and plank is 0.2. If a horizontal force of magnitude 2 mg is applied to the plank B, the acceleration of A relative to the plank and relative to the ground respectively, are:

View SolutionBlock A of mass m rests on the plank B of mass 3m which is free to slide on a frictionless horizontal surface. The coefficient of friction between the block and plank is 0.2. If a horizontal force of magnitude 2 mg is applied to the plank B, the acceleration of A relative to the plank and relative to the ground respectively, are :
View SolutionFigure shown a small block A of mass m kept at the left end of a plank B of mass M=2m and length l . The system can slide on a horizontal road. The system is started towards right with the initial velocity v. The friction coefficient between the road and the plank is 1/2 and that between the plank and the block is 1/4 . Find

(a) the time elapsed before the block separates from the plank
(b) displacement of block and plank relative to ground till that moment.
View Solution
