∣∣
∣
∣
∣
∣
∣
∣
∣∣List-I(Orbitals)List-II(Approach axis is z-axis)(Type of bond)(P)py+py(1)σ−bond(Q)py+px(2)π−bond(R)pz+pz(3)δ−(delta)bond(S)dx2−y2+dx2−y2(4)No bond will form∣∣
∣
∣
∣
∣
∣
∣
∣∣
Code :Solution in Gujrati
Number of roots of equation in [−2π,2π]is2form=1
Number of roots of equation in [−2π,2π]is1form=9
Roots are real for m≤1
If sin x =t then t2−(m−3)t+m=0 can not have both roots in [-1,1]
The correct Answer is:A, C, D
Step by step video & image solution for |{:(,"List-I(Orbitals)",,"List-II"),(,"(Approach axis is z-axis)",,"(Type of bond)"),((P),p_(y)+p_(y),(1),sigma-bond),((Q),p_(y)+p_(x),(2),pi-bond),((R ),p_(z)+p_(z),(3),delta-("delta")bond),((S),d_(x^(2)-y^(2))+d_(x^(2)-y^(2)),(4),"No bond will form"):}| Code : by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - MATHEMATICS
34 Videos
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS SECTION-I
20 Videos
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - Chemistry
95 Videos
- SEQUENCE AND PROGRESSIONBOOK - ALLEN CHAPTER - SEQUENCE AND PROGRESSIONEXERCISE - Exercise (JA)
10 Videos
- TEST PAPERSBOOK - ALLEN CHAPTER - TEST PAPERSEXERCISE - MATHEMATICS
136 Videos
Similar Questions
5∑k=01sin[(K+1)θ]sin[(K+2)θ] can be equal to
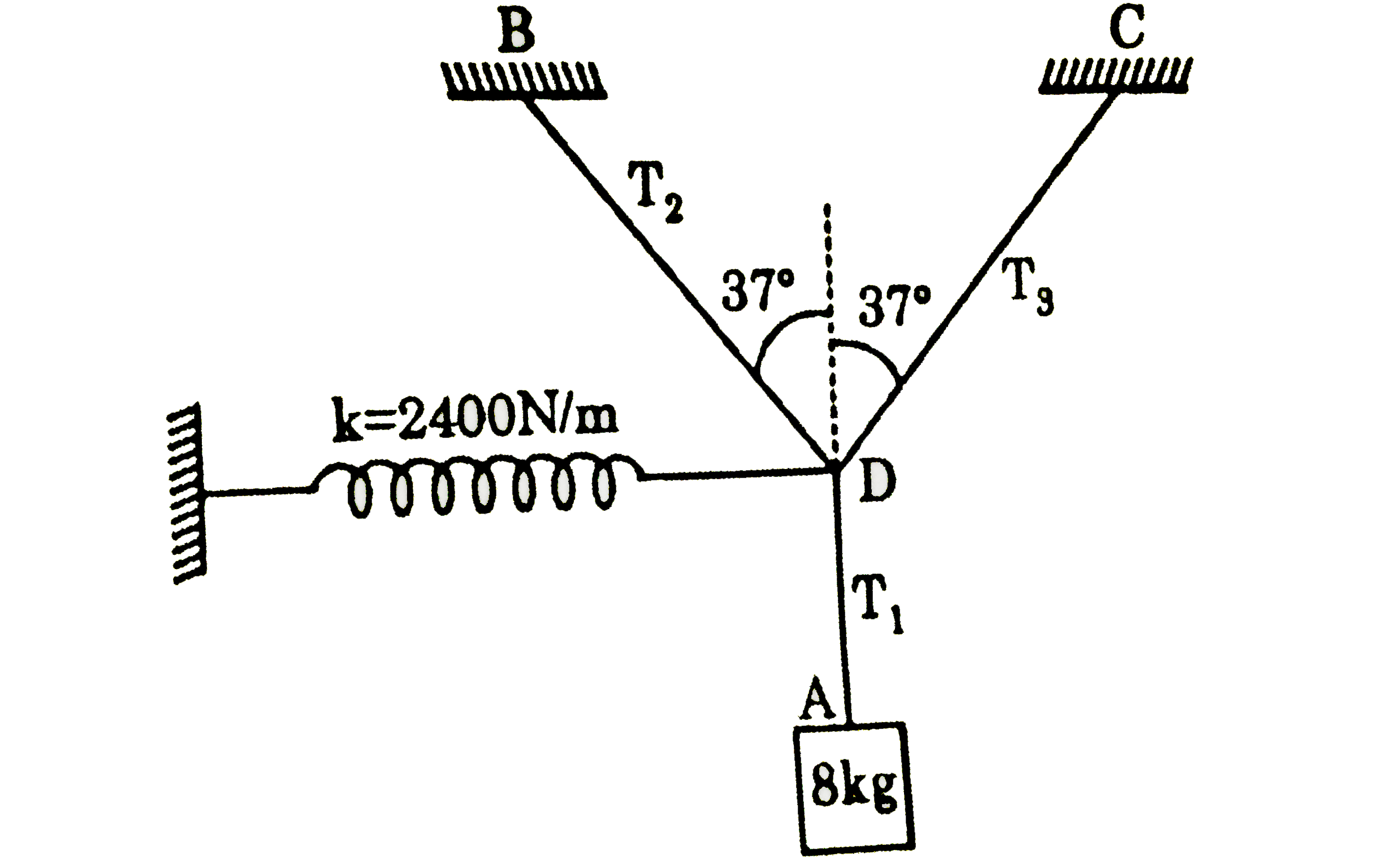
View SolutionGiven arrangement is in equilibrium and elongation in spring is 1cm. Tension in string AD is T1, in string BD is T2 and is string CD is T3.Choose correct values of T1,T2andT3:
View SolutionWhich of the following statement(s) is/are correct
View SolutionThe minimum value of the sum of real numbers a−5,a−4,3a−3,1,a8 and a10 with a>0 is
View SolutionIf a be sum of the odd numbered terms and b the sum of even numbered terms of the expansion (1+x)n,n ε N then (1−x2)n is equal to
View SolutionA molecule (X) has (i) four sigma bonds formed by hte overlap of sp^(2) and s orbitals, (ii) one sigma bond formed by sp^(2) and sp^(2) -orbitals nad (iii) on pi -bond formed by p_(z) and p_(z) orbitals. Which of the following is X?
View SolutionHow many of these orbitals have maximum orbital angular distribution is maximum at an angle of 45∘ to the axial direction.
dxy,dx2−y2dyz,dxz,dz2,PxPyPz,s
View SolutionWhich of the following molecules has both p_(pi)-p_(pi) and p_(pi)-d_(pi) bonds
View SolutionWhich has p_(pi) - d_(pi) bonding ?
View SolutionWhich type of overlapping is shown by p(Px,Pyand Pz) - orbitals ?
View Solutionp_(y) -orbital can not form pi -bond by lateral overlap with :
View SolutionCondsider the following combination of atomic orbitals : combinding orbitals (internuclear axis ) Combindig orbitals (internuclear axis). (i) s+p_(x)(x) (ii) d_(xy)+d_(xy)(x) (iii) d_(xy)+P_(x)(z) (iv) s+s(z) (v) d_(yz)+d_(yz)(x) (vi) p_(x)+p_(x)(z) (vii) d_(z^(2))+d_(z^(2))(z) (viii) d_(xy)+d_(xy)(z) (ix) p_(x)+p_(x)(y) (x) s+p_(z)(x) Then calculate value of a^(2)+b^(2)+2cd . (where a=sigma M.O.,b=pi M.O. , C=delta M.O., d=non-bonding M.O.)
View SolutionIF molecular axis is X then which of the following overlapping will form pi bond? p_(z)+p_(z),p_(x)+p_(x),p_(x),p_(y),s+p_(z),p_(y)+p_(y)
View SolutionDraw dx2−y2 orbital and Pz orbital.
View Solutionpy-orbital can not form π -bond by lateral overlap with :
View SolutionCondsider the following combination of atomic orbitals : combinding orbitals (internuclear axis ) Combindig orbitals (internuclear axis). (i) s+p_(x)(x) (ii) d_(xy)+d_(xy)(x) (iii) d_(xy)+P_(x)(z) (iv) s+s(z) (v) d_(yz)+d_(yz)(x) (vi) p_(x)+p_(x)(z) (vii) d_(z^(2))+d_(z^(2))(z) (viii) d_(xy)+d_(xy)(z) (ix) p_(x)+p_(x)(y) (x) s+p_(z)(x) Then calculate value of "a^(2)+b^(2)+2cs" . (where a=sigma M.O.,b=pi M.O. , C=delta M.O., d=non-bonding M.O.)
View Solution
