A missile is fired for maximum range with an initial velocity of 20m/s. If g=10m/s2, the range of the missile is Solution in Gujrati
40m
50m
60m
20m
The correct Answer is:A
Maximum range Rmax=u2g=(20)210=40m
Topper's Solved these Questions
- MOTION IN A PALNEBOOK - ALLEN CHAPTER - MOTION IN A PALNEEXERCISE - EXERCISE-2
170 Videos
- MOTION IN A PALNEBOOK - ALLEN CHAPTER - MOTION IN A PALNEEXERCISE - EXERCISE-3
41 Videos
- MOTION IN A PALNEBOOK - ALLEN CHAPTER - MOTION IN A PALNEEXERCISE - PHY
75 Videos
- KINEMATICS-2DBOOK - ALLEN CHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)
47 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLEN CHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (JA)
4 Videos
Similar Questions
The velocity of a particle moving with constant acceleration at an instant t0 is 10m/s After 5 seconds of that instant the velocity of the particle is 20m/s. The velocity at 3 second before t0 is:-
View SolutionAssertion : A body can have acceleration even if its velocity is zero at a given instant of time.
Reason : A body is momentarily at rest when it reverses its direction of motion.
View SolutionA particle goes along a quadrant of a circle of radius 10m from A to B as shown in fig. Find the magnitude of displacement and distance along the path AB, and angle between displacement vector and x-axis ?
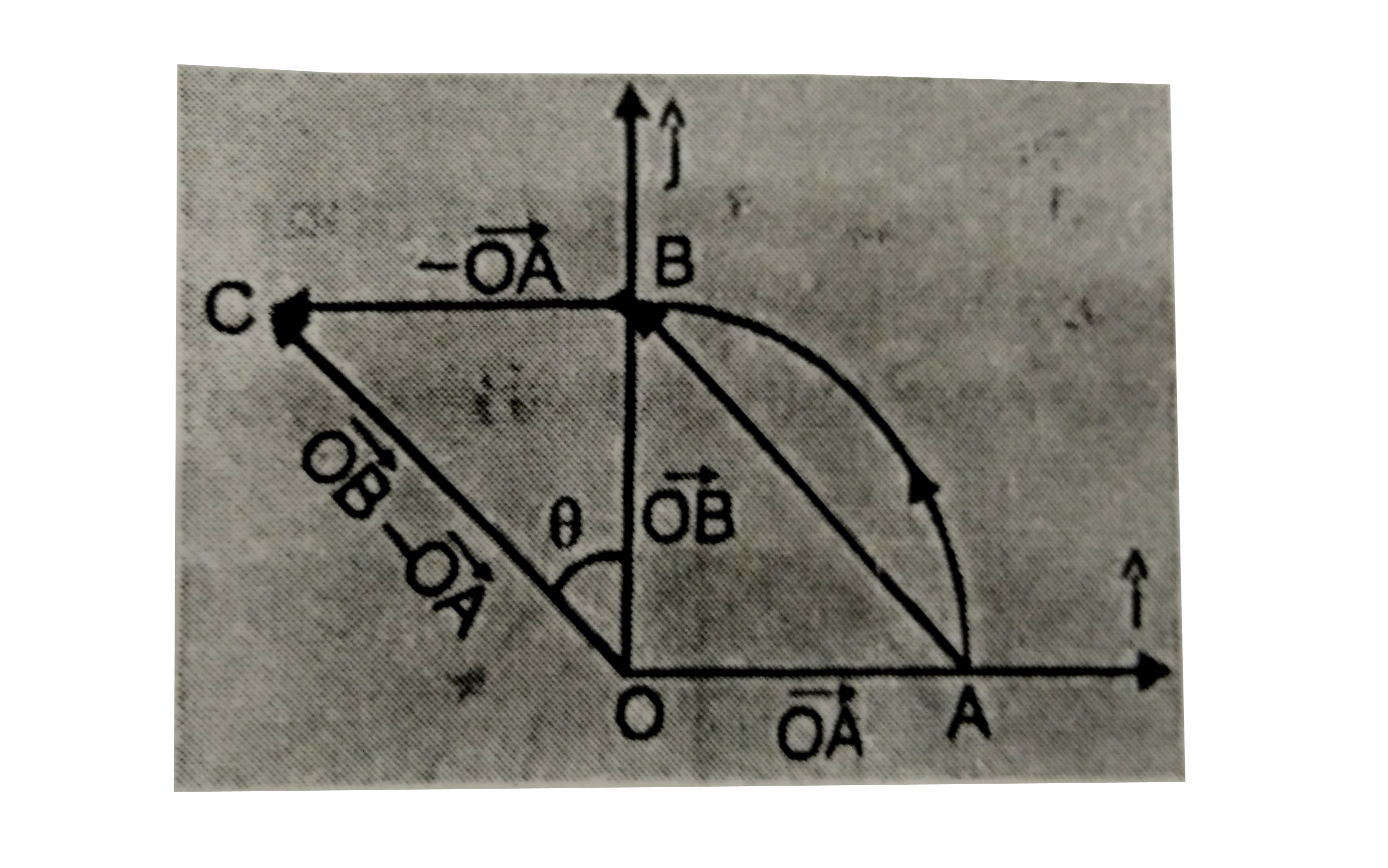
View SolutionA particle is moving in xy-plane .At certain instant the components of its velocity and acceleration are as follows ux=3m/s,uy=4m/s,ax=2m/s2anday=1m/s2. The rate of change of speed at this moment is
View SolutionA piece of wire is bent in the shape of a parabola y=kx2 (y-axis vertical) with a bead of mass m on it. The bead can slide on the wire without friction. It stays at the lowest point of the parabola when the wire is at rest. The wire is now accelerated parallel to the x-axis with a constant acceleration a. The distance of the new equilibrium position of the bead, where the bead can stay at rest with respect to the wire, from the y-axis is:
View SolutionA missile is fired for maximum range with an initial velocity of 20m//s . If g=10m//s^(2) , the range of the missile is
View SolutionChoose the correct option (i) The speed of a projectile at its maximum height is half of its initial speed. The angle of projection is 60^(@) . (ii) A missile is fired for maximum range with an initial velocity of 20 m//s , the range of the missile is 40 m . (iii) A ball is projected with a kinetic energy E at an angle of 45^(@) to the horizontal. At the highest point during its flight, its kinetic energy will be E//2 . (iv) An object is projected at an angle of 45^(@) with horizontal. The horizontal range and the maximum height will be in the ratio 4:1
View SolutionA bullet is fired from a cannon with velocity 500 m/s. If the angle of projection is 15∘ and g=10m/s2. Then the range is
View SolutionThe maximum range of a bullet fired from a toy pistol mounted on a car at rest is R_(0)=40m . What will be the acute angle of inclination of the pistol for maximum range when the car is moving in the direction of firing with uniform velocity V=20m//s , on a horizontal surface? (g=10m//s^(2))
View SolutionIndia successfully test-fired its indigenously developed HeliNa missile recently at the Pokhran Range in Rajasthan, the missile is categorised as ……missile?
View Solution20 मी/से के प्रारंभिक वेग से एक मिसाइल अधिकतम दूरी तक प्रक्षेपित होती है | यदि g=10 मी/से2 हो , तो मिसाइल की प्रक्षेपित दूरी है -
View SolutionA body projected at 45^(@) with a velocity of "20m/s" has a range of 10m.The decrease in range due to air resistance is g=10ms^(-2)
View Solutionएक प्रक्षेपणास्त्र को अधिकतम परास के लिये प्रणोदित किया गया है। इसका प्रारंभिक वेग 20 m/s है। यदि g=10m/s हो तो प्रक्षेपणास्त्रकी परास.होगी
View SolutionThe minimum and maximum velocities of a projectile are 10ms−1 and 20ms−1 respectively. The horizontal range and maximum height are respectively (g=10 ms−2)
View Solution20 मी/से के प्रारंभिक वेग से एक मिसाइल अधिकतम दूरी तक प्रक्षेपित होती है | यदि g=10 मी/से2 हो , तो मिसाइल की प्रक्षेपित दूरी है -
View SolutionThe minimum and maximum velocities of a projectile are 10ms^(-1) and 20ms^(-1) respectively. Find the horizontal range and maximum height (g=10ms^(-2))
View Solution
