A swimmer starts to swim from point a to cross a river. He wants to reach point B on the opposite side of the river. The line AB makes an angle with the river flow as shown. The velocity of the swimmer in still water is same as that of the water
(i) In what direction should he try to direct his velocity ? Calculate angle between his velocity and river velocity.
(ii) Find the ratio of the time taken to cross the river in this situation to the minimum time in which he can cross this river.
 Solution in Gujrati
Solution in Gujrati
The correct Answer is:(i) (ii)
Step by step video & image solution for A swimmer starts to swim from point a to cross a river. He wants to reach point B on the opposite side of the river. The line AB makes an angle 60^(@) with the river flow as shown. The velocity of the swimmer in still water is same as that of the water (i) In what direction should he try to direct his velocity ? Calculate angle between his velocity and river velocity. (ii) Find the ratio of the time taken to cross the river in this situation to the minimum time in which he can cross this river. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- KINEMATICS-2DBOOK - ALLEN CHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-1)
40 Videos
- KINEMATICS-2DBOOK - ALLEN CHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)
47 Videos
- KINEMATICS-2DBOOK - ALLEN CHAPTER - KINEMATICS-2DEXERCISE - Exercise (S-1)
30 Videos
- GRAVITATIONBOOK - ALLEN CHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
- MOTION IN A PALNEBOOK - ALLEN CHAPTER - MOTION IN A PALNEEXERCISE - SOLVED EXAMPLE
28 Videos
Similar Questions
A particle is projected form a horizontal plane (x−z plane) such that its velocity vector at time t is gives by →V=aˆi+(b−ct)ˆj. Its range on the horizontal plane is given by
View SolutionA particle is moving in xy-plane .At certain instant the components of its velocity and acceleration are as follows ux=3m/s,uy=4m/s,ax=2m/s2anday=1m/s2. The rate of change of speed at this moment is
View SolutionThe vertical height y and horizontal distance x of a projectile on a certain planet are given by x=(3t)m,y=(4t−6t2) m where t is in seconds, Find the speed of projection (in m/s).
View SolutionAssertion : The difference in the value of acceleration due to gravity at pole and equator is proportional to square of angular velocity of earth.
Reason : the value of acceleration due to gravity is minimum at the equator and maximum at the pole.
View SolutionA particle thrown over a triangle from one end of a horizontal base falls on the other end of the base after grazing the vertex. If αandβ are the base angles of triangle and angle of projection is θ, then prove that (tanθ=tanα+tanβ)
View SolutionA swimmer wands to cross a river and reach point B directly from A. The speed of the wimmer in still river and that of river flow are same. The dotted line AN is normal to flow direction of river. For swimmer to reach point B, the angle his velocity relative to river will make with line AN is given by θ. then the value of θ is :
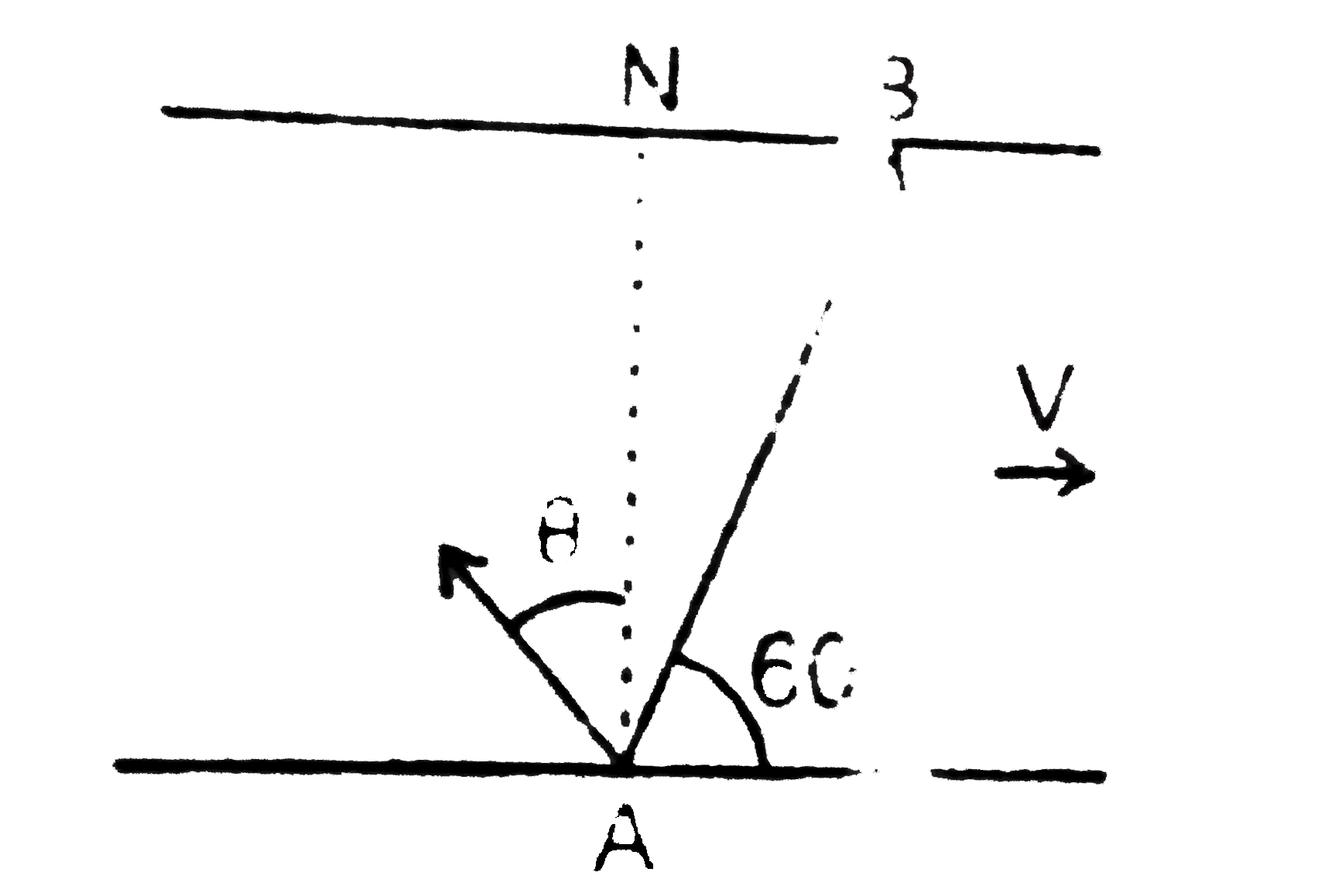
View SolutionA swimmer starts to swim from point a to cross a river. He wants to reach point B on the opposite side of the river. The line AB makes an angle 60∘ with the river flow as hown. The velocity of the swimmer in still water is same as that of the water

(i) In what direction should he try to direct his velocity ? Calculate angle between his velocity anf river velocity.
(ii) Find the ratio of the time taken to cross the river in this situation to the minimum time in which he can cross this river.
View SolutionA swimmer starts to swim from point a to cross a river. He wants to reach point B on the opposite side of the river. The line AB makes an angle 60^(@) with the river flow as shown. The velocity of the swimmer in still water is same as that of the water (i) In what direction should he try to direct his velocity ? Calculate angle between his velocity ? Calculate angle between his velocity and river velocity. (ii) Find the ratio of the time taken to cross the river in this situation to the minimum time in which he can cross this river.
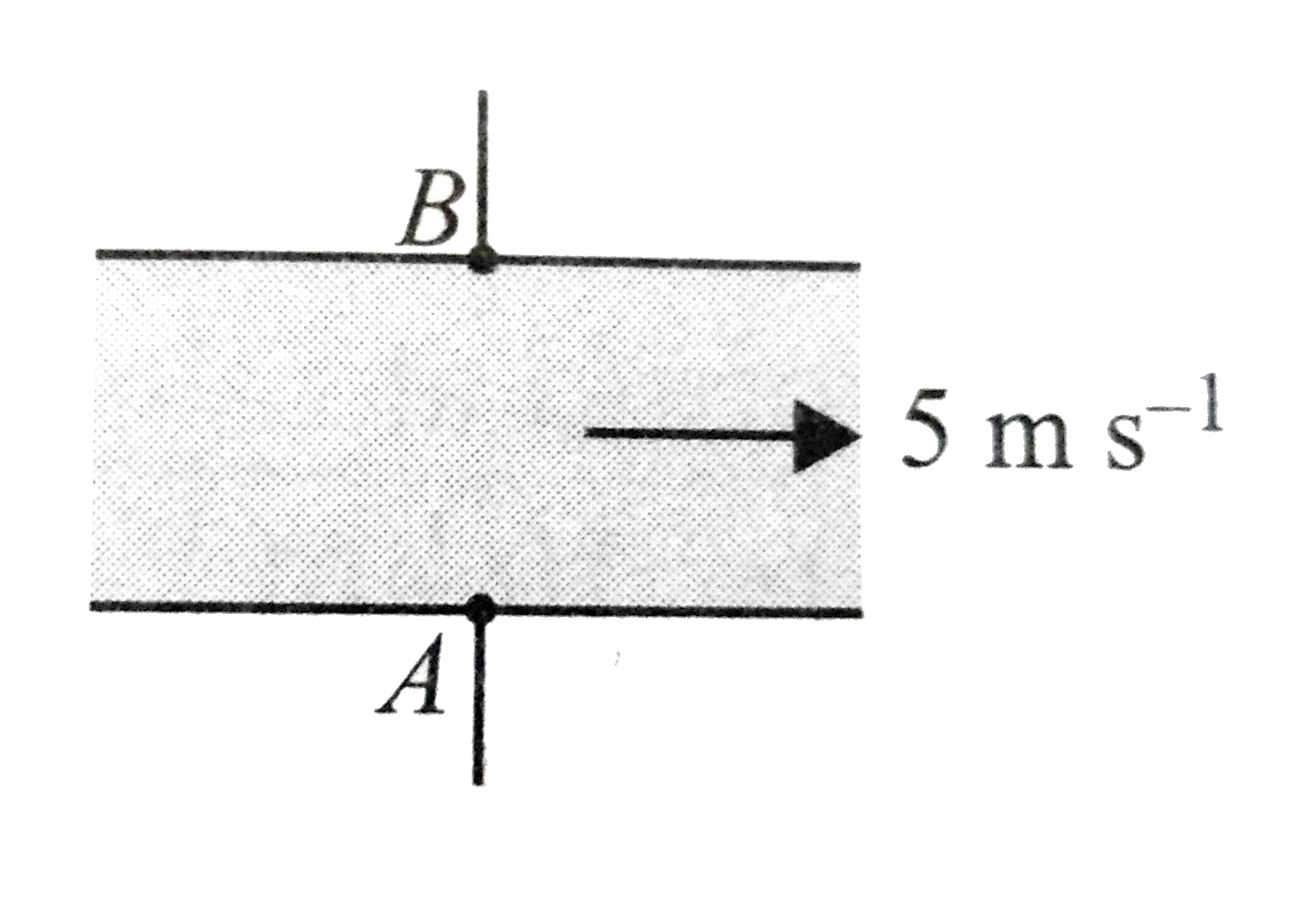
View SolutionA river is flowing from west to east with a speed 5 m s−1. A swimmer can swim in still water at a speed of 10 m s−1 .
If he wants to start from point A on south bank and reach opposite point B on north bank. In what direction direction should he swim ?
View SolutionA swimmer crosses the river along the line making an angle of 45^(@) with the direction of flow. Velocity of the river is 5 m/s. Swimmer takes 6 seconds to cross the river of width 60 m. The velocity of the swimmer with respect to water will be :
View SolutionA swimmer can swim with a velocity of 10 kmph w.r.t the water flowing in a river with a velocity of 5 kmph. In what direction should he swim to reach the point on the other bank just opposite to his starting point ?
View SolutionA river is flowing with a velocity of 2m/s. If the width of river in 100 m and swimmer wants to cross river is shortest time, what time (in sec) would he take if velocity of swimmer in still water is 4 m/s ?
View SolutionA river is flowing from west to east direction with velocity v. A swimmer standing at the south bank wants to cross the river in order to reach a point just in front of the starting point. If the swimmer can swim at a speed of u w.r.t. the river flow, the angle with the north direction, required to swim is equal to?
View SolutionA river is flowing from west to east with a speed 5m/s. A swimmer can swim in still water at a speed of 10m/s .
If he wants to start from point A on south bank and reach opposite point B on north bank. In what direction direction should he swim ?

View SolutionA person wants to reach point B on the other side of the river. The river is flowing in the direction shown in the picture. What should be the velocity of man relative to water. So that it reaches point B? In which direction does he swim?
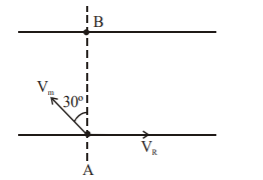
View SolutionA swimmer swims with a speed of 10ms at angle of 1200 from direction of river flow. Find velocity of river flow such that swimmer reach exactly opposite point of bank.
View Solution
