Show that projection angle θ0 for a projectile launched from the origin is given by:
θ0=tan−1[4HmR] Where Hm= Maximum height, R=RangeSolution in Gujrati
Hm=u2sin2θ02g,R=u2gsin2θ0=2u2gsinθ0.cosθ0
HmR=14tanθ0⇒θ0=tan−1(4HmR)
Topper's Solved these Questions
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLEN CHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-6
9 Videos
- KINEMATICSBOOK - ALLEN CHAPTER - KINEMATICSEXERCISE - EXERCISE-2
89 Videos
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - Question
1 Videos
Similar Questions
A particle is projected vertically upwards from ground with velocity 10 m/s. Find the time taken by it to reach at the highest point ?
View SolutionA student performs an experiment to determine how the range of a ball depends on the velocity with which it is projected. The "range" is the distance between the points where the ball lends and from where it was projected, assuming it lands at the same height from which it was projected. It each trial, the student uses the same baseball, and launches it at the same angle. Table shows the experimental results.
∣∣ ∣ ∣ ∣ ∣ ∣∣TrailLaunch speed(m/s)Range(m)110822031.833070.7440122.5∣∣ ∣ ∣ ∣ ∣ ∣∣
Based on this data, the student then hypothesizes that the range, R, depends on the initial speed v0 according to the following equation : R=Cvn0, where C is a constant and n is another constant.
Based on this data, the best guess for the value of n is :-
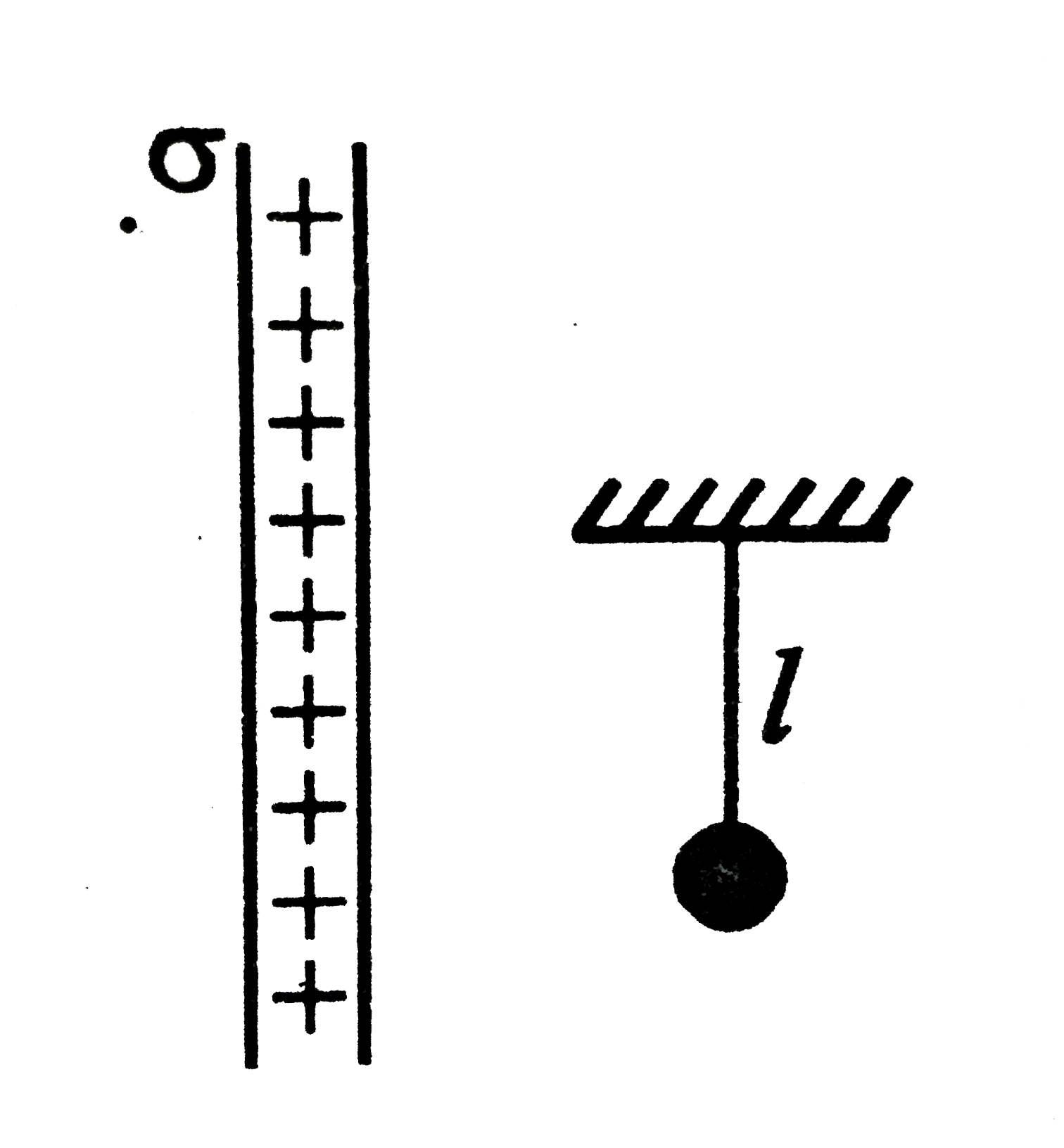
View SolutionA simple pendulum of length l and bob mass m is hanging in front of a large nonconducting sheet having surface charge density σ. If suddenly a charge +q is given to the bob & it is released from the position shown in figure. Find the maximum angle through which the string is deflected from vertical .
 .
.
View SolutionShow that projection angle θ0 for a projectile launched from the origin is given by:
θ0=tan−1[4HmR] Where Hm= Maximum height, R=Range
View SolutionFind the ratio of maximum horizontal range and the maximum height attained by the projectile. i.e. for theta_(0) = 45^(@)
View SolutionA body is projected at an angle theta with the horizontal. Derive an expression for its horizontal range. Show that there are two angles theta_(1) and theta_(2) projections for the same horizontal range, such that theta_(1)+theta_(2)=90^(@) .
View SolutionThe distance of the roots of the equation tanθ0zn+tanθ1zn−1+…+tanθn=3 from z=0, where θ0,θ1,θ2,…,θn∈[0,π4] satisfy
View SolutionShow that the projection angle theta_0 for a projectile launched from the origin is given by theta_0=tan^(-1)((4H)/R) Where the symbols have their usual meaning.
View Solution(a) Show that for a projectile the angle between the velocity and the X-axis as a function of time is given by
θ(t)=tan−1[voy−gtvox]
(b) Show that the projection angle θ0 for a projectile lunched from the origin is given by θ0=tan−1(4hmR),
where the symbols have their usual meanings .
View SolutionA particle is projected with velocity u at angle θ1 with horizontal .The ratio of range and maximum height is 4. When it is projected at angle θ2 with horizontal with same speed,the ratio of range and maximum height is 2.Then tanθ1tanθ2 will be equal to
View SolutionA projectile's launch speed is 6.00 times that of its speed at its maximum height. Find the launch angle theta_(0) .
View Solution(a) Show that for a projectile the angle between the velocity and the x - axis as a function of time is given by
θ(t)=tan−1(v0y−gtv∘x)
(b) Shows that the projection angle θ∘ for a projectile launched from the origin given by
θ0=tan−1(4hmR)
where the symbols have their usual meaning.
View Solution(a) Show that for a projectile the angle between the velocity and the x - axis as a function of time is given by
θ(t)=tan−1(v0y−gtv∘x)
(b) Shows that the projection angle θ∘ for a projectile launched from the origin given by
θ0=tan−1(4hmR)
where the symbols have their usual meaning.
View Solution(a) Show that for a projectile the angle between the velocity and the x - axis as a function of time is given by
θ(t)=tan−1(v0y−gtv∘x)
(b) Shows that the projection angle θ∘ for a projectile launched from the origin given by
θ0=tan−1(4hmR)
where the symbols have their usual meaning.
View SolutionShow that for a projectile the angle between the velocity and the x-axis as function of time is given
by θt=tan−1(v0y−gtvox)
(b) Shows that the projection angle θ0 for a projectile launched from the origin is given by θt=tan−1(4hmR)
where the symbols have their usual meanings.
View SolutionProve that, projectile from the origin theta_(0) Projection angle when projected at projection angle theta_(0)=tan^(-1)[(4H_(m))/(R)] it happens. Where H_(m) = Maximum height, R = Horizontal range
View Solution
