Relation between displacement x and time t is x=2−5t+6t2, the initial acceleration will be :-Solution in Gujrati
−3ms−2
12ms−2
2ms−2
−5ms−2
The correct Answer is:B
Step by step video & image solution for Relation between displacement x and time t is x=2-5t+6t^(2), the initial acceleration will be :- by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Integration)
12 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Graphs))
28 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Trigonometry)
22 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLEN CHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
Find the value of x1, so that ∫x10ydx=5
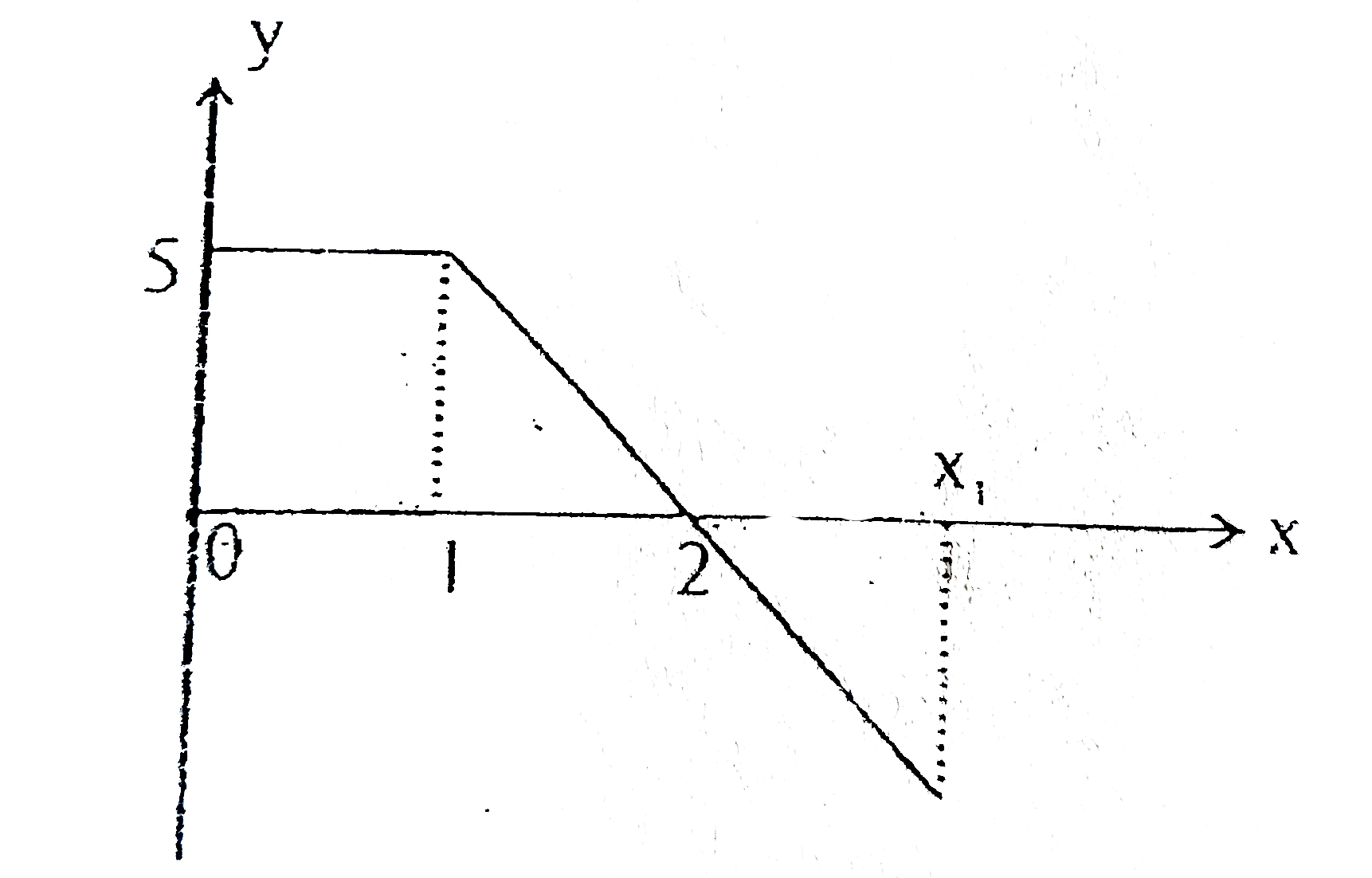
View SolutionWhich of the following equation is best representation of following graph's?
View SolutionConvert the angle from degree to radian :
(a) 30∘(b) 45∘(c) 60∘(d) 90∘(e) 120∘(f) 135∘(g) 210∘(h) 270∘(i) 315∘
View SolutionAssertion: The driver in a vechicle moving with a constant speed on a straight road is in a non-inertial frame of referance.
Reason: A reference frame in which Newton's laws of motion are applicable is non-inertial.
View SolutionA rod with rectangular cross section oscillates about a horizontal axis passing through one of its ends and it behaves like a seconds pendulum, its length will be
View SolutionA particle starts from rest with a unifor acceleration. Its displacement x after x seconds is given in metres by the relation x = 5 + 6t +7t^2 Calculate the magnitude of its (i) initial velocity (ii) velocity at t = 3 s (iii) uniform acceleration and (iv) displacement at t= 5 as.
View SolutionA particle moves along X-axis in such a way that its coordinate X varies with time t according to the equation x=(2−5t+6t2)m. The initial velocity of the particle is
View SolutionA particle moves along the x-axis such that its co-ordinate (x) varies with time (t), according to the expression x=2−5t+6t2, where x is in metres and t is in seconds. The initial velocity of the particle is :–
View Solutionएक कण X - अक्ष के अनुदिश इस प्रकार गति करता है कि उसका x - निर्देशांक समय t के साथ निम्न समीकरण के अनुसार बदलता है −x=(2−5t+6t2),
जहाँ x, मीटर में तथा t सेकंड में है। कण का प्रारंभिक वेग ज्ञात कीजिये ।
View SolutionIf the displacement of a particle is x=t3−4t2−5t, then the acceleration of particle at t=2 is
View SolutionIf displacement x at time t is x=√1+t2, then acceleration is
View SolutionIf displacement x at time t is x=√1+t2 , then acceleration is
View Solutionविस्थापन S व समय t सूत्र S=2t2+5t+9 से सम्बन्ध है। समय t=2 सेकण्ड पर त्वरण होता है-
View SolutionA particle moves along the x-axis in such a way that its x-co-ordinate varies with time as x = 2 – 5t + 6t^(2) . The initial velocity and acceleration of particle will respectively be-
View SolutionIf x = 4t^(2) - 5t and y = 6t , then acceleration of a body is given by
View SolutionA particle moves along the x-axis in such a way that its coordinates x varies with time 't' according to the equation x=2-5t+6t^(2) . What is the initial velocity of the particle?
View Solution
