The figure shows a modified Young’s double slit experimental set-up. Here SS2−SS1=λ/4.
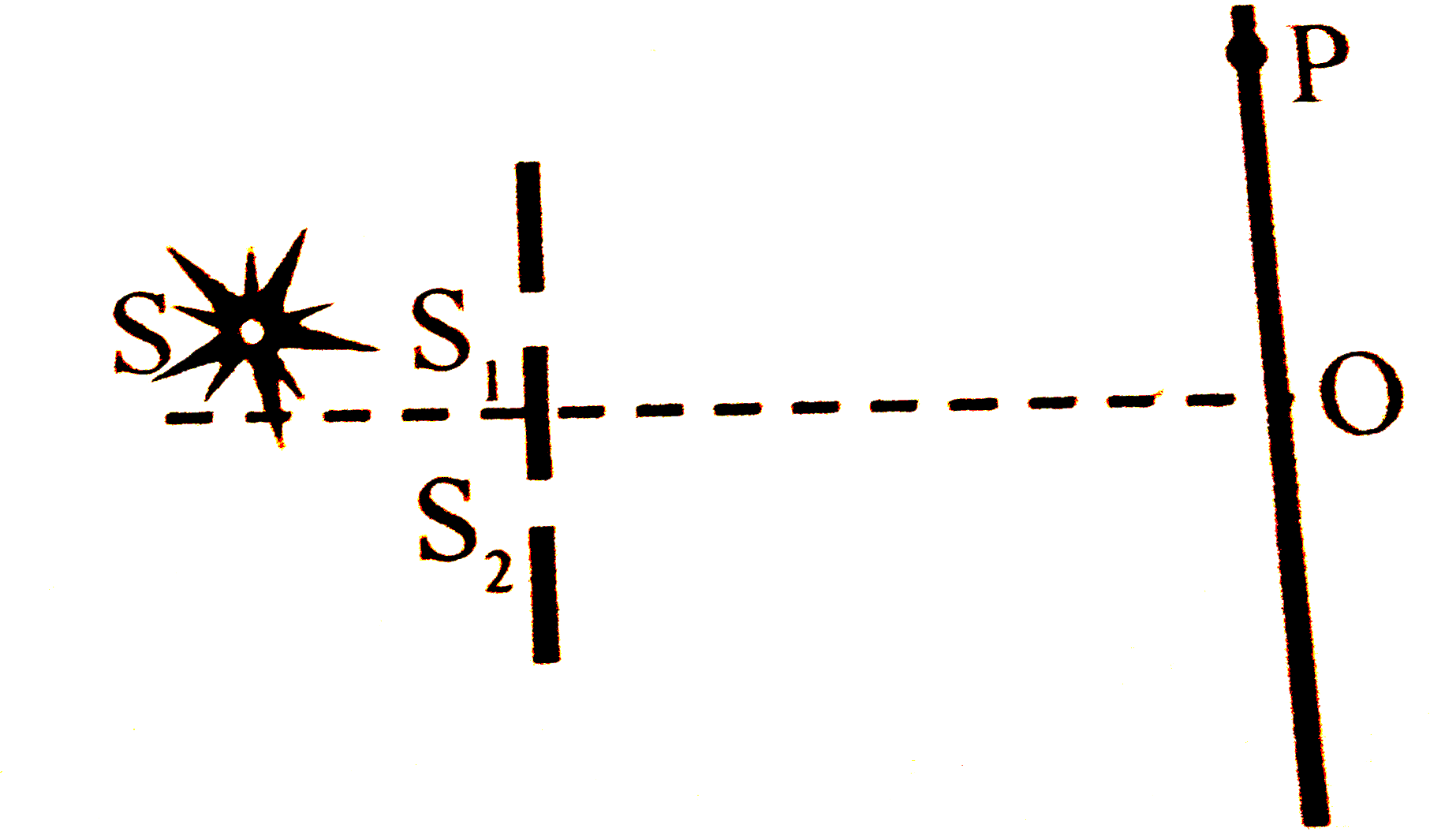
(a) Write the condition for constructive interference.
(b) Obtain an expression for the fringe width. Solution in Gujrati
The path difference S2P−S1P=[yndD+λ4]
For constructive interference , path difference =nλ,
Where n=0,1,2,3,…..
[yndD+λ4]=nλ,
So, position of nth bright fringe is =yn=(n−14)λDd
Fringe widthβ=yn−yn−1
Or β=λDd
Topper's Solved these Questions
Similar Questions
Choose the correct statement:
View SolutionA particle is projected vertically upwards with a velocity v. It returns to the ground in time T. Which of the following graphs correctly represents the motion.
View SolutionLet S1 and S2 be the two slits in Young's double-slit experiment. If central maxima is observed at P and angle ∠S1PS2=θ, then fringe width for the light of wavelength λ will be
View SolutionFig. shows an experimental set up simillar to Young's double slit experiment to observe interference of light. Here SS_(2) - SS_(1) = lambda//4 . Write down the conditions of (i) Contstructive interference (ii) Destructive interference at any point P in terms of path diff. (S_(2)P - S_(1)P) . Does the central fringe observed in the above set up lie above or below O ? Give reason.
View SolutionIn a Young's double slit experiment, let S_(1) and S_(2) be the two slits, and C be the centre of the screen. If angle S_(1)CS_(2)=theta and lambda is the wavelength, the fringe width will be
View SolutionThe figure shows a modified Young's double slit experimental set-up. Here SS_(2)-SS1=lamda//4. (a) Write the condition for constructive interference. (b) Obtain an expression for the fringe width.
View SolutionThe figure shows a modified Young’s double slit experimental set-up. Here S S_(2)-S S_(1)=lambda//4 . (a) Write the condition for constructive interference. (b) Obtain an expression for the fringe width.
View SolutionIn an interference experiment, the two slits S1andS2 are not equidistant from the source S. then the central fringe at 0 will be
View SolutionObtain the expression for fringe width in the case of interference of light waves.
View SolutionIn Young’s double slit experiment, if width (aperture) of the slit S is increased keeping other parameters constant, then the interference frings will :
View SolutionObtain the expression for fringe width of interference fringes in Young's double slit experiment.
View SolutionIn a modified set up of Young's double slit experiment, it is given that SS2−SS1=λ4 i.e., the source S is not equidistant from the slits S1andS2.
(a) Obtain the condition for constructive and destructive interference at any point P on the screen in terms of the path difference Δ=S2P−S1P.
(b) Does the observed central bright fringe lie above or below O ? Give reason in support of your answer.
View SolutionObtain the expression for fringe width in the case of interference of light waves.
View SolutionThe figure shows a modified Young's double slit experimental set-up.
Here SS2−SS1=λ/4.
(a) Write the condition for constructive interference.
(b) Obtain an expression for the fringe width.
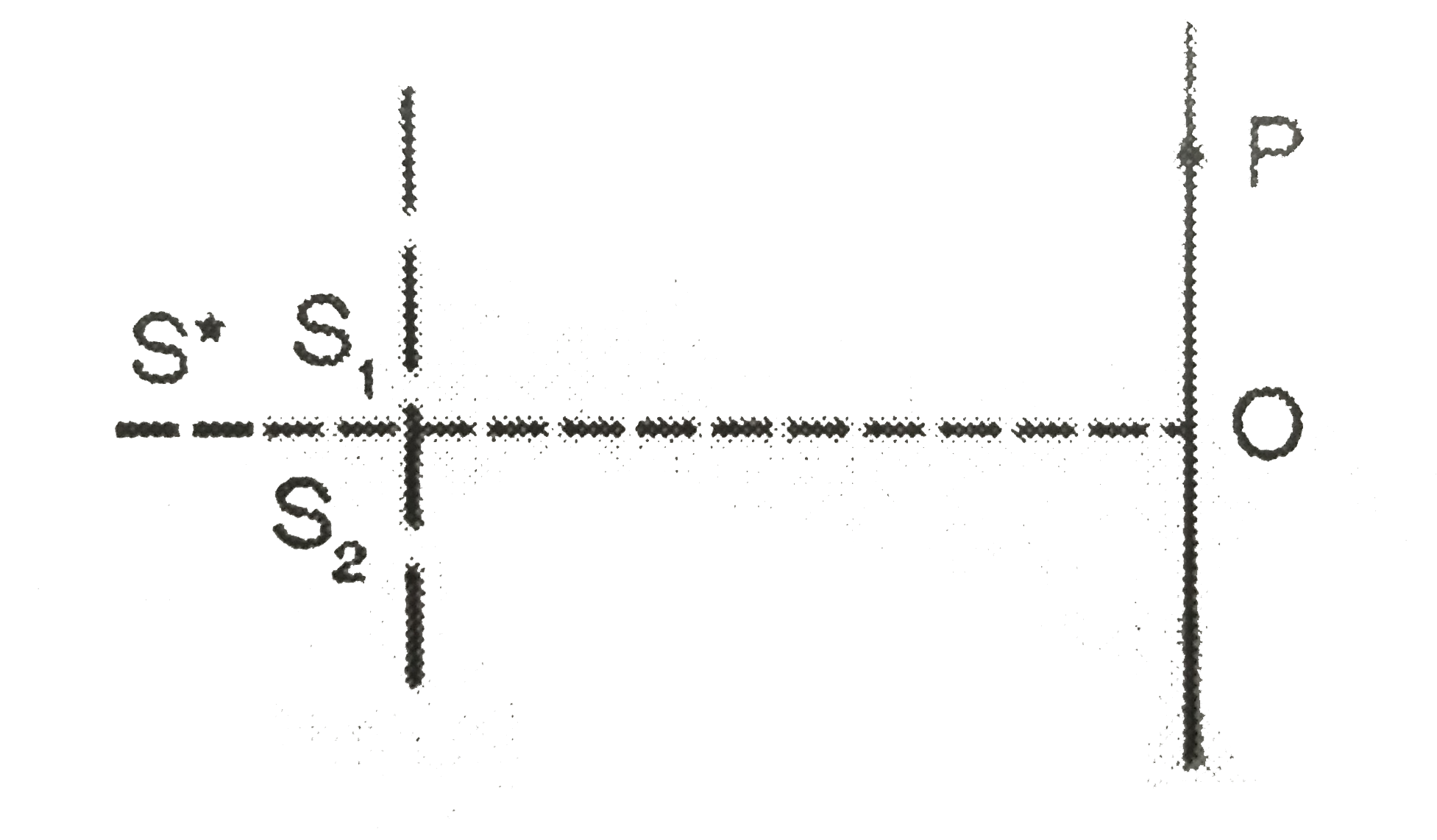
View SolutionIn a Young's double slit experiment, let S1 and S2 be the two slits, and C be the centre of the screen. If ∠S1CS2=θ and λ is the wavelength, the fringe width will be
View SolutionObtain the expression for fringe width in the case of interference of light waves.
View Solution