Question

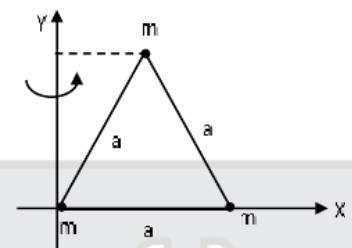
Side of an equilateral triangle is I . Three point masses, each of magnitude m, are palced at the three vertices of the triangle . Momment of inertia of this system about one side of the triangle as axis is given by

Options

A. `(3ml^2)/4`
B. `4/3 mt^2`
C. `3/2 ml^2`
D. `2/3 ml^2`

Answer

A

Explanation

To find the moment of inertia of a system of three point masses placed at the vertices of an equilateral triangle about one of its sides, we can follow these steps:

1. Understand the Configuration:
- We have an equilateral triangle with side length \( L \).
- Three point masses, each of mass \( m \), are placed at the vertices of the triangle.

2. Identify the Axis of Rotation:
- The axis of rotation is along one of the sides of the triangle. Let's denote the vertices of the triangle as \( A \), \( B \), and \( C \) with the side \( AB \) being the axis.

3. Calculate the Moment of Inertia for Each Mass:
- The moment of inertia \( I \) about an axis is given by the formula:
\( I = \sum m_i r_i^2 \)
- Where \( m_i \) is the mass and \( r_i \) is the distance from the axis of rotation.

4. Calculate for Mass at Vertex A and B:
- The masses at vertices \( A \) and \( B \) are on the axis of rotation. Therefore, their distances from the axis are zero:
\( I_A = m \cdot 0^2 = 0 \)
\( I_B = m \cdot 0^2 = 0 \)

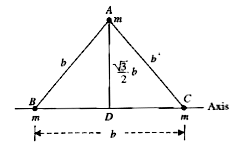
5. Calculate for Mass at Vertex C:
- The distance of mass \( m \) at vertex \( C \) from the axis \( AB \) can be calculated using the height of the triangle. The height \( h \) of an equilateral triangle is given by:
\( h = \frac{\sqrt{3}}{2} L \)
- The distance from the side \( AB \) to vertex \( C \) is \( h \):
\( r_C = h = \frac{\sqrt{3}}{2} L \)
- Therefore, the moment of inertia for mass \( C \) is:
\( I_C = m \cdot \left(\frac{\sqrt{3}}{2} L\right)^2 = m \cdot \frac{3}{4} L^2 \)

6. Total Moment of Inertia:
- Now, we can sum up the moments of inertia:
\( I_{total} = I_A + I_B + I_C = 0 + 0 + m \cdot \frac{3}{4} L^2 = \frac{3}{4} m L^2 \)

Final Result:
The moment of inertia of the system about one side of the triangle is:
\( I = \frac{3}{4} m L^2 \)