Electric field at centre of a uniforly charged semicirlce of radius a is
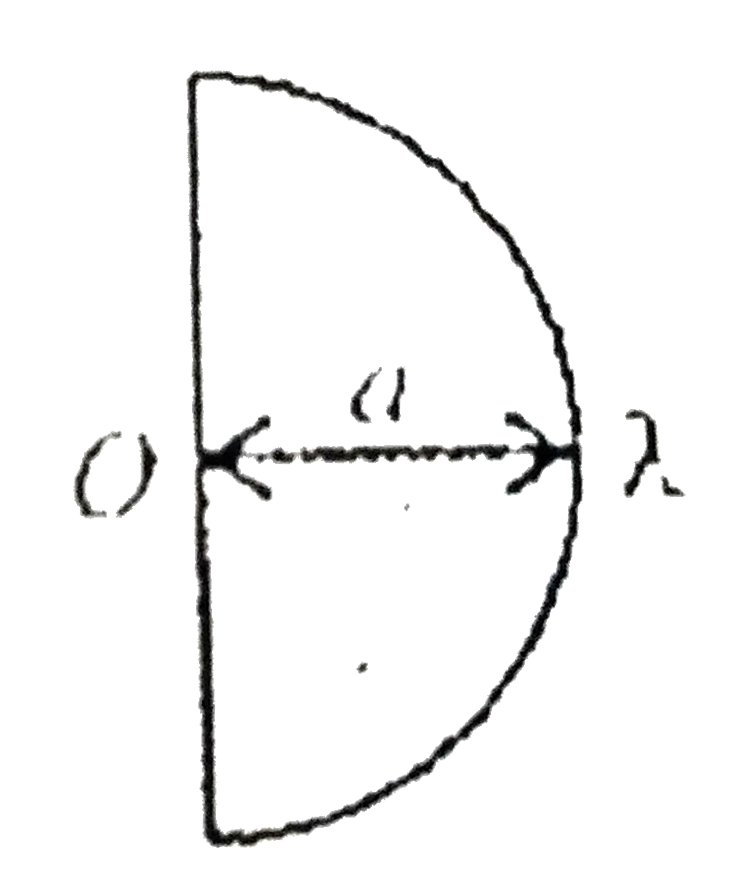
λ2πε0a3
λ2πε0a2
λ22πε0a
λ2πε0a
The correct Answer is:D
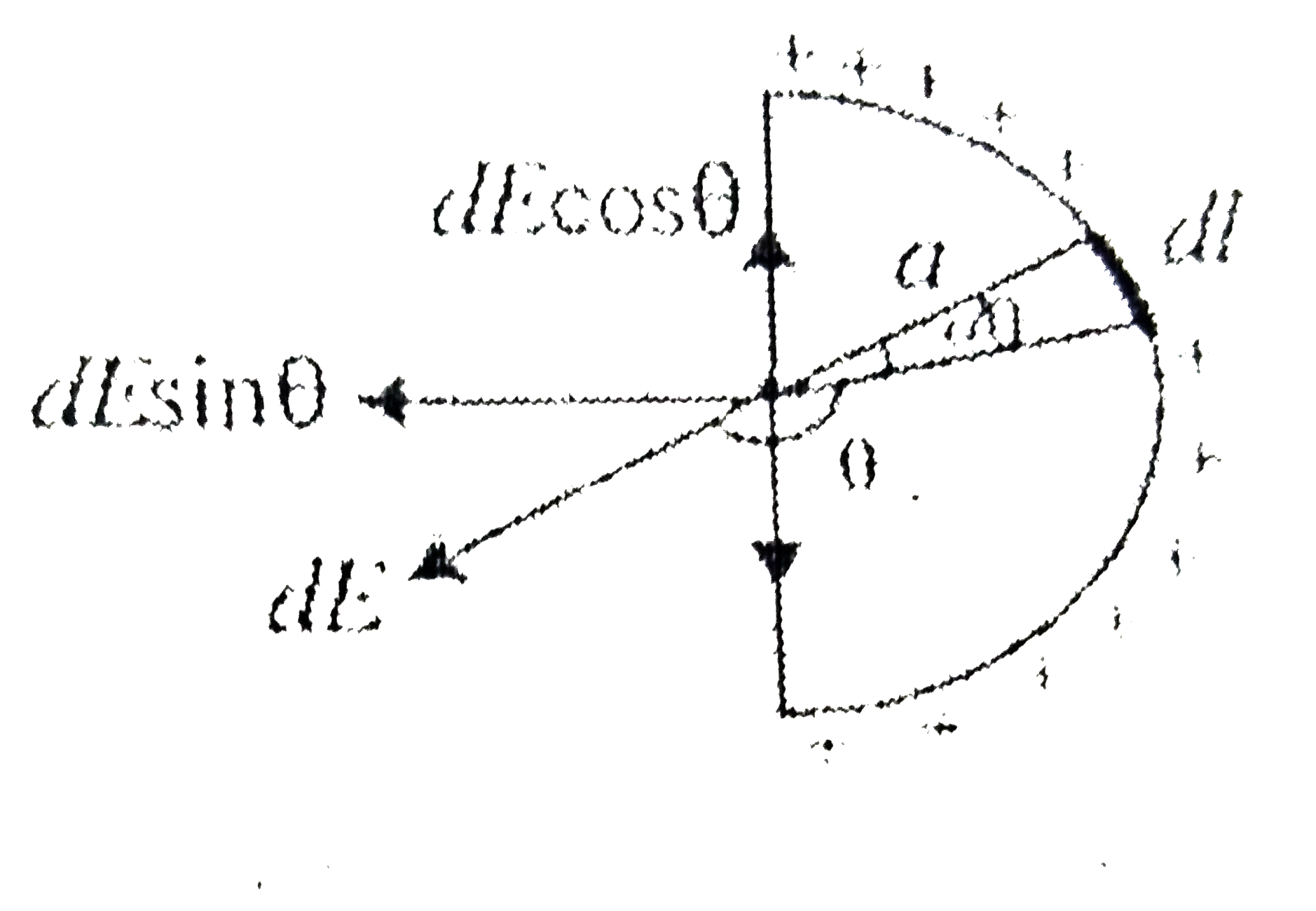
Electtric field intensity at O due to small elemental length dl of charged ring .
dE=14πε0⋅λdla2=14πε0⋅λadθa2
dE=14πε0⋅λadθ
∴ Net electric field at centre O is
E = ∫dEsinθ=π∫014πε0λasinθdθ
=λ4πε0a[−cosθ]π0
∴E=λ2πε0a.
Similar Questions
Find the electric field at the centre of a uniformly charged semicircular ring of radius R. Linear charge density is λ
View SolutionIf the potential at the centre of a uniformly charged hollow sphere of radius R is V, then electric field at a distance r from the centre of sphere will be (r>R).
 View Solution
View SolutionThe electric field due to uniformly charged sphere of radius R as a function of the distance from its centre is represented graphically by
View SolutionElectric field at the centre of uniformly charge hemispherical shell of surface charge density σ is σnε0 then find the value of n.
View SolutionAt which distance along the centre axis of a uniformaly charged plastic disc of radius R is the magnitude of the electric field equal to one-half the magnitude of the field at the centre of the surface of the disc ?
View SolutionElectric field at centre O of semicircule of radius 'a' having linear charge density λ given is given by
 View Solution
View SolutionAt a point 20cm from the centre of a uniformly charged dielectric sphere of radius 10cm, the electric field is 100V/m. The electric field at 3cm from the centre of the sphere will be
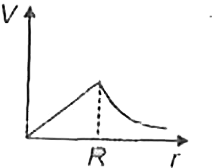
View SolutionWhich graph best represents the variation of electric potential as a function of distance from the centre of a uniformly charged solid sphere of charge of radius R ?
 View Solution
View SolutionThe electric field at 2R from the centre of a uniformly charged non - conducting sphere of rarius R is E. The electric field at a distance R2 from the centre will be
View SolutionAssertion: Electric potential on the surface of a charged sphere of radius R is V. Then electric field at distance r=R2 from centre is V2R. Charge is distributed uniformly over the volume.
Reason: From centre to surface, electric field varies linearly with r. Here r is distance from centre.View Solution
