An injfinite large sheet has charg density σC/m2 Find electric field at a distance d perendicular to the sheet.
E=σ2ε0
E=σε0
E=2σε0
None of these
The correct Answer is:A
To solve the problem of finding the electric field at a distance d perpendicular to an infinite sheet with charge density σ (in C/m²), we can follow these steps:
Step 1: Understand the Configuration
We have an infinite sheet with a uniform charge density σ. The electric field produced by such a sheet is uniform and directed away from the sheet if the charge is positive and towards the sheet if the charge is negative.
Hint: Visualize the infinite sheet and the direction of the electric field lines.
Step 2: Apply Gauss's Law
According to Gauss's Law, the electric flux Φ through a closed surface is equal to the charge enclosed Q divided by the permittivity of free space ϵ0:
Φ=Qϵ0
Hint: Remember that the electric flux through a surface is the product of the electric field E and the area A of the surface.
Step 3: Choose a Gaussian Surface
For an infinite sheet, we can choose a Gaussian surface in the form of a cylindrical pillbox that straddles the sheet. The area of the top and bottom faces of the pillbox is A.
Hint: The electric field is constant and perpendicular to the surface of the sheet, so the flux through the curved surface of the cylinder is zero.
Step 4: Calculate the Electric Flux
The electric flux through the top and bottom faces of the pillbox is given by:
Φ=E⋅A+E⋅A=2EA
where E is the magnitude of the electric field at distance d from the sheet.
Hint: Since the electric field is uniform, you can treat it as constant over the area A.
Step 5: Determine the Charge Enclosed
The charge enclosed by the Gaussian surface is:
Q=σA
Hint: The charge density σ is the amount of charge per unit area.
Step 6: Set Up the Equation Using Gauss's Law
Substituting the expressions for electric flux and charge into Gauss's Law gives:
2EA=σAϵ0
Hint: Notice that the area A cancels out from both sides.
Step 7: Solve for the Electric Field
Now, we can solve for E:
2E=σϵ0
E=σ2ϵ0
Hint: This result shows that the electric field is independent of the distance d from the sheet.
Final Answer
Thus, the electric field at a distance d perpendicular to the infinite sheet is:
E=σ2ϵ0
We have an infinite sheet with a uniform charge density σ. The electric field produced by such a sheet is uniform and directed away from the sheet if the charge is positive and towards the sheet if the charge is negative.
According to Gauss's Law, the electric flux Φ through a closed surface is equal to the charge enclosed Q divided by the permittivity of free space ϵ0:
Φ=Qϵ0
For an infinite sheet, we can choose a Gaussian surface in the form of a cylindrical pillbox that straddles the sheet. The area of the top and bottom faces of the pillbox is A.
The electric flux through the top and bottom faces of the pillbox is given by:
Φ=E⋅A+E⋅A=2EA
where E is the magnitude of the electric field at distance d from the sheet.
The charge enclosed by the Gaussian surface is:
Q=σA
Substituting the expressions for electric flux and charge into Gauss's Law gives:
2EA=σAϵ0
Now, we can solve for E:
2E=σϵ0
E=σ2ϵ0
Thus, the electric field at a distance d perpendicular to the infinite sheet is:
E=σ2ϵ0
Similar Questions
Knowledge Check
Two infinite plane parallel sheets, separated by a distance d have equal and opposite uniform charge densities σ. Electric field at a point between the sheets is
Aσ2ε0Bσε0CzeroDdepends on the location of the point
An infinite thin plane sheet of charge density 7.08×10−11(C/m2) is held in air. Find the electric field at a point very close to the sheet.
View SolutionA charged disc of radius 3 m has charge density σ. Electric field at a distance of 4 m from its centre on axis is _______
View Solution
let there be a non conducting infinite large sheet of charge of surface charge density 136πnC/m2 An uncharged conducting spherical shell of radius 20 cm is placed in from of sheet. A point charge 6 m C is placed at centre of the shell. force on point charge due to shell is x mN. Find velue of x.
View SolutionA uniform rod AB of mass m and length l is hinged at its mid point C. The left half (AC) of the rod has linear charge density −λ and the right half (CB) has +λ where λ is constant. A large non conducting sheet of uniform surface charge density σ is also present near the rod. Initially the rod is kept perpendicular to the sheet. The end A of the rod is initialy at a distance d. Now the rod is rotated by a small angle in the plane of the paper and released. Prove that the rod will perform SHM and find its time period.

View SolutionTwo large parallel sheets charged uniformly with surfasce charge density σ and −σ are located as shown in the figure. Which one of the following graphs shows the variation of electric field along a line perpendicular to the sheets as one moves from A to B ?

View SolutionThere are two infinite plane sheets each having uniform surface charge density +σC/m2 They are inclined to each other at an angle 30∘as shown in the figure. Electric field at any arbitrary point P is:
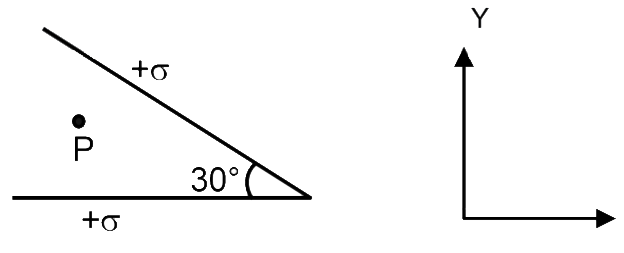
View SolutionThere are two infinite plane sheets each having uniform surface charge density +σC/m2 They are inclined to each other at an angle 30∘as shown in the figure. Electric field at any arbitrary point P is:

View Solution
