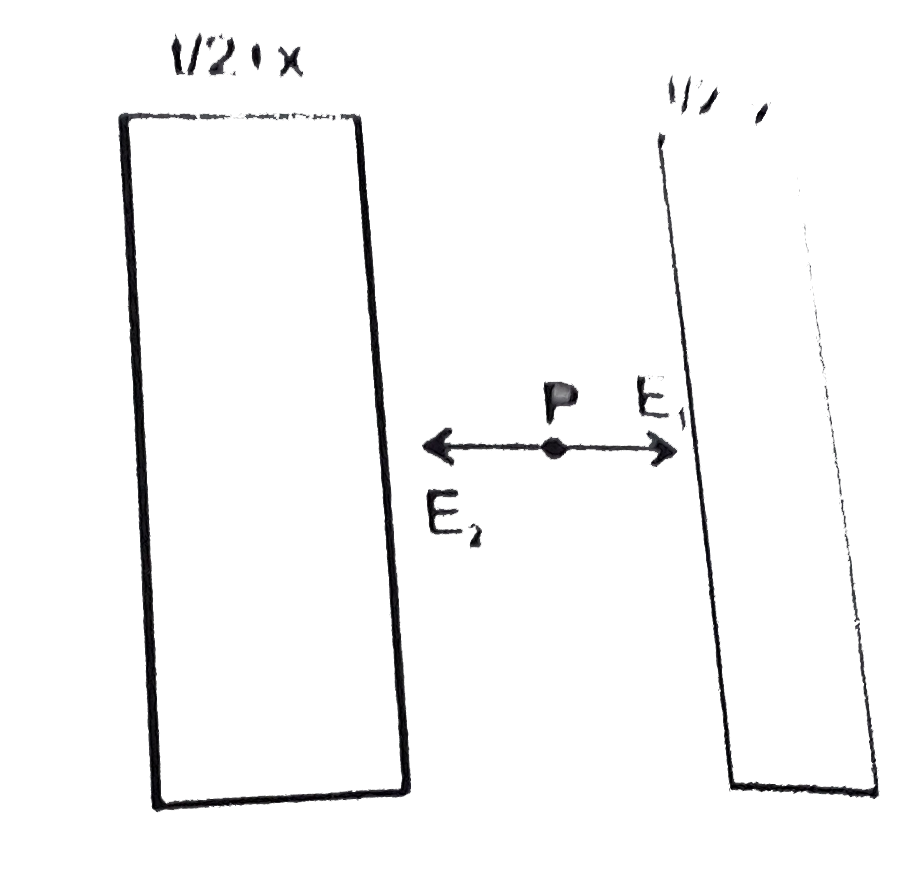
An infinitely large non-conducting sheet of thickness t and uniform volume charge density ρ is given in which left half og the sheet contains charge density ρ and right half contains charge density. Find the electric field at the symmetry plane of this sheet
We can consider two sheets of thickness (t2−x) and (t2+x)
When a point lies inside the sheet.
Net electric field at point P:E=E1−E2=Q12Aε0−Q22Aε0
[Q1 : charge on left sheet, Q2= change of right sheet]
=AP(t2+x)−2ρA(t2−x)2Aε0=ρ[3x−t2]2ε0
At the symmetry plane, x=0 So, E=−ρt4ε0
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Exercise-1 Section (A)9videos
ELECTROSTATICS
- BOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Exercise-1 Section (B)12videos
ELECTROSTATICS
- BOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - HLP39videos
ELECTROSTATICS
- BOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - A.l.P19videos
ELECTROMAGNETIC INDUCTION
- BOOK - RESONANCECHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II10videos
EXPERIMENTAL PHYSICS
Similar Questions
An infinite thin plane sheet of charge density 7.08×10−11(C/m2) is held in air. Find the electric field at a point very close to the sheet.
View SolutionAn infinite thin plane sheet of charge density 7.08×10−11(C/m2) is held in air. Find the electric field at a point very close to the sheet.
View Solution
Knowledge Check
- Question 1 - Select One
A large nonconducting sheet M is given a uniform charge density. Two unchared small metal rods A and B are placed near the sheet as shown in figure
 AM attracts ABA attract BCM attracts BDB repels A
AM attracts ABA attract BCM attracts BDB repels A - Question 2 - Select One
An injfinite large sheet has charg density σC/m2 Find electric field at a distance d perendicular to the sheet.
AE=σ2ε0BE=σε0CE=2σε0DNone of these - Question 3 - Select One
Two infinite sheets of charge carry equal and opposite uniform charge densities ±σ.The electric field in the free space between the two sheets is
Aσε0Bσ2ε0C2σε0DZero
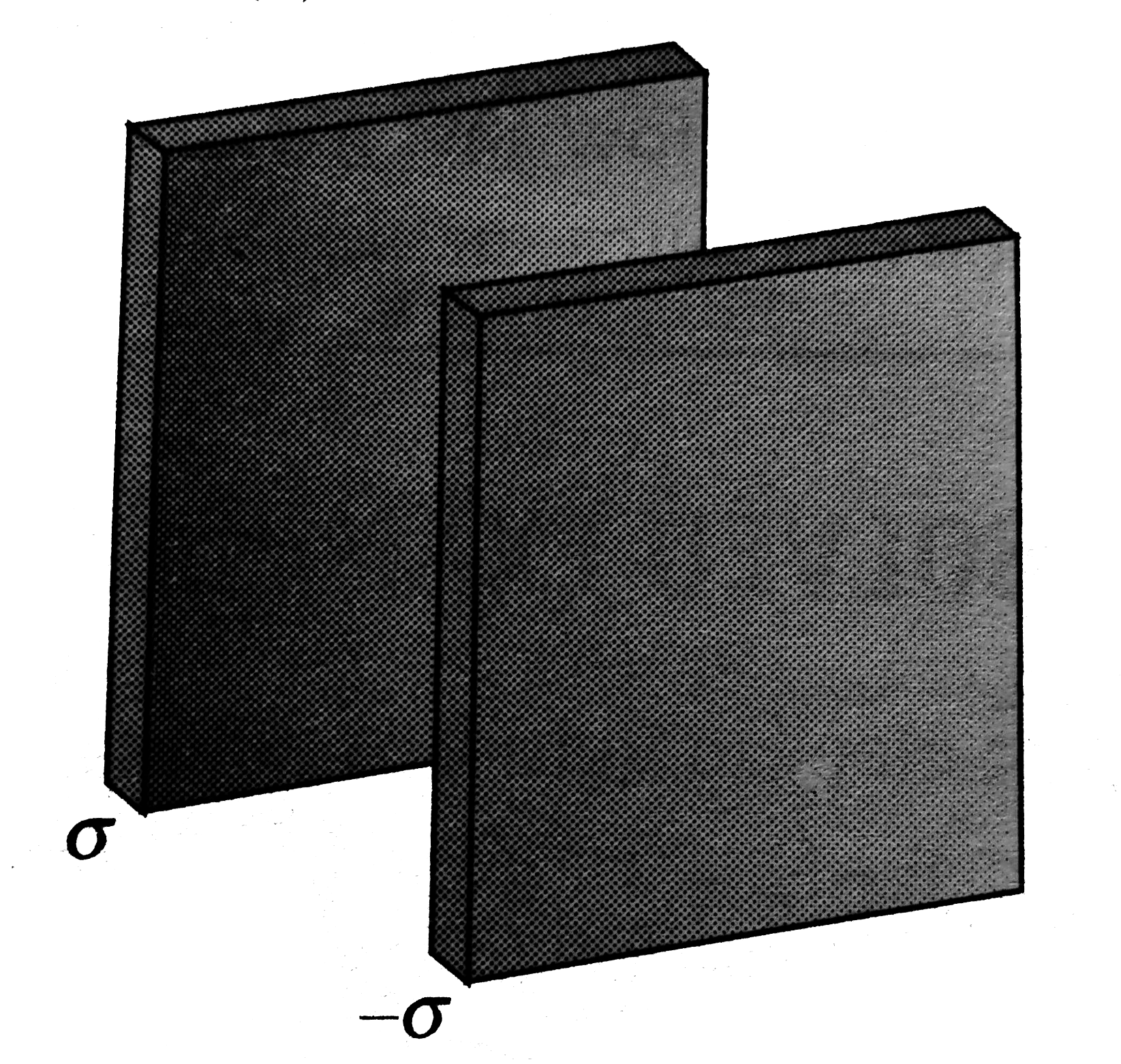
Two infinite, non-conducting sheets of charge are parallel to each other, as shown in figure. The sheet on the left has a uniform surface charge density σ, and the one on the right has a uniform charge density −σ. Calculate the electric field at points (a) to the left of, (b) in between, and (c) to the right of the two sheets.
 View Solution
View SolutionThere is a hemisphere of radius R having a uniform volume charge density ρ. Find the electric potential and field at the centre
View SolutionDetermine and draw the graph of electric field due to infinitely large non-conducting sheet of thickness t and uniform volume charge density ρ as a function of distance x from its symmetry plane.
(a) x<t2 (b) x≥t2View SolutionTwo infinite sheets of charge carry equal and opposite uniform charge densities ±σ.The electric field in the free space between the two sheets is
View SolutionA ring carries a uniform linear charge density on one half and the linear charge density of same magnitude but opposite sign on the other half.
View Solution

