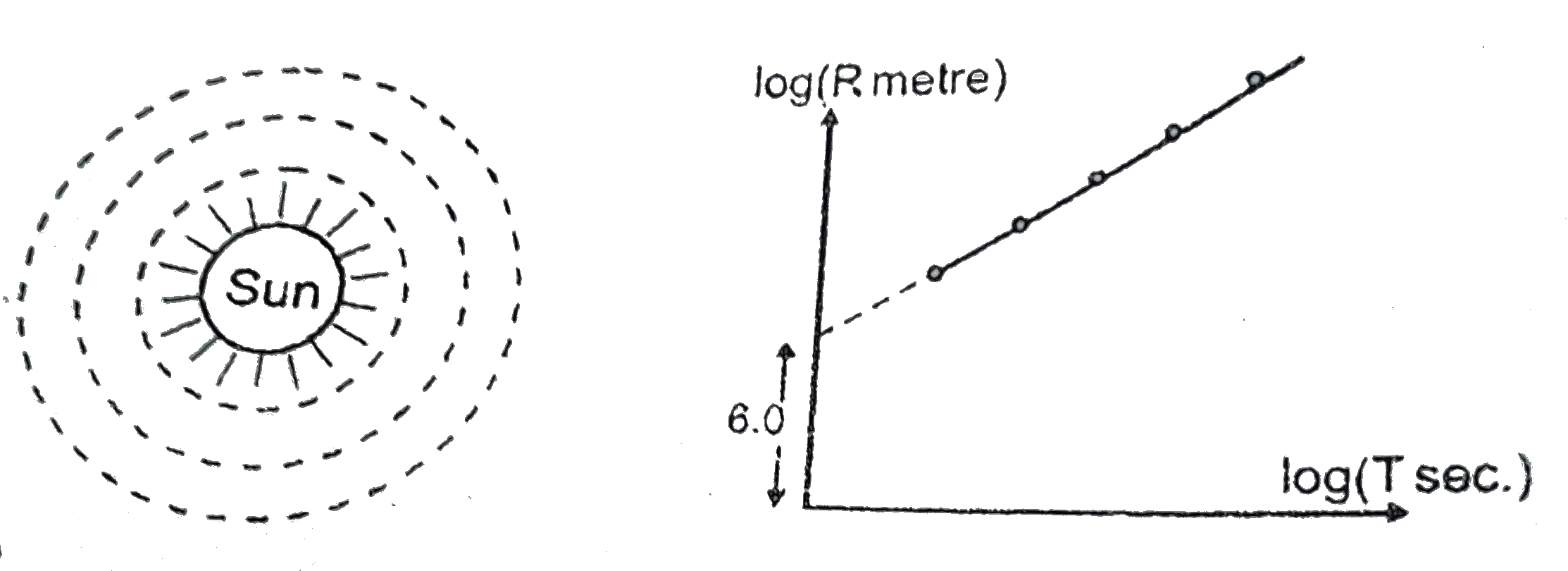
For many planets revolving around the stationary sun in circular orbits of different radii (R), the time periods (T) were noted. Then log (g ) v/s log (T) curve was plotted
[G=203×10−11 in M.K.S. system, π2=10]
Estimate the mass of the sun.
![For many planets revolving around the stationary sun in circular orbits of different radii (R), the time periods (T) were noted. Then log (g ) v/s log (T) curve was plotted
[G=20/3 xx 10^-11 in M.K.S. system, pi^2=10]
Estimate the mass of the sun.](https://d10lpgp6xz60nq.cloudfront.net/physics_images/TAR_MHT_CET_PHY_MCQ_XI_C03_E10_005_Q01.png) Solution in Marathi
Solution in Marathi
6×1029kg
5×1020kg
8×1025kg
5×1025kg
Step by step video & image solution for For many planets revolving around the stationary sun in circular orbits of different radii (R), the time periods (T) were noted. Then log (g ) v/s log (T) curve was plotted [G=20/3 xx 10^-11 in M.K.S. system, pi^2=10] Estimate the mass of the sun. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A body while sliding went straight down an incline of 45∘ to the horizontal and was subjected to a coefficient of kinetic friction of 0.20. Starting from rest, hwo long did it take him to reach a speed of 50km/hr? (Ignore air resistance and take h=10m/s2)
View SolutionWhen a bus suddenly takes a turn, the passengers are thrown outwards because of
View SolutionIf a planet of mass m is revolving around the sun in a circular orbit of radius r with time period, T then mass of the sun is
View SolutionA planet is revolving around the sun in a circular orbit with a radius r. The time period is T .If the force between the planet and star is proportional to r^(-3//2) then the quare of time period is proportional to
View SolutionMany planets are revolving around the fixed sun in circular orbits of different radius (R) and difference time period (T) To estimate the mass of the sun the orbital radius (R) and time period (T) of planets were noted. Then "log"_(10) T v/s "log"_(10)R curve was plotted. The curve was found to be approximately straight line (as shown in) having y intercept =6.0 (Neglect the gravitational interaction among the planets) [Take G = (20)/(3) xx 10^(-11) in MKS, pi^(2)= 10 ] The slope of the line should be .
View SolutionMany planets are revolving around the fixed sun in circular orbits of different radius (R) and difference time period (T) To estimate the mass of the sun the orbital radius (R) and time period (T) of planets were noted. Then "log"_(10) T v/s "log"_(10)R curve was plotted. The curve was found to be approximately straight line (as shown in) having y intercept =6.0 (Neglect the gravitational interaction among the planets) [Take G = (20)/(3) xx 10^(-11) in MKS, pi^(2)= 10 ] Estimate the mass of the sun .
View SolutionMany planets are revolving around the fixed sun in circular orbits of different radius (R) and difference time period (T) To estimate the mass of the sun the orbital radius (R) and time period (T) of planets were noted. Then log10 T v/s log10R curve was plotted.
The curve was found to be approximately straight line (as shown in) having y intercept =6.0 (Neglect the gravitational interaction among the planets) [Take G=203×10−11 in MKS, π2=10]
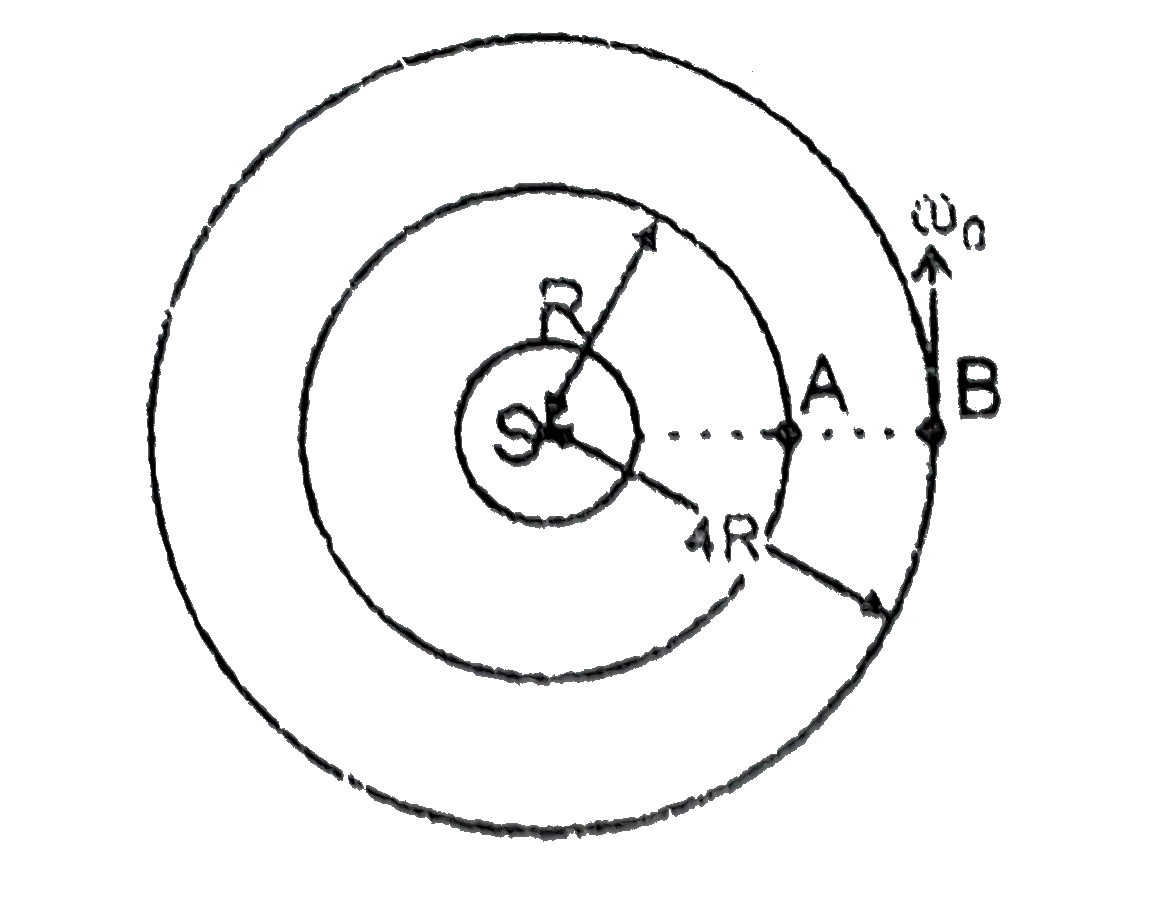
Two plantes A and B having orbital radius R and 4R are initally at the closest position and rotating in the same direction if angular velocity of planet B is ω0 then after how much time will both the planets be again in the closest postion? (Neglect the interaction between planets)
 .
.
View SolutionThe period of revolution of a planet around the sun in a circular orbit is T. If a similar planet is revolving in a circular orbit of the same radius around the sun with its mass twice as that of planet, its period of revolution would be
View SolutionFor many planets revolving around the stationary sun in circular orbits of different radii (R), the time periods (T) were noted. Then log(R) v/s log· (T) curve was plotted
[G=203×10−11 in M.K.S system, π2=10]
Estimate the mass of the sun.

View SolutionUsing orbital radius r and the corresponding periodic time T of different satellite revolving around a planet, what would be the slope of graph of log T rarr log r ?
View SolutionIf a planet of mass .m. is revolving around the sun in a circular orbit of radius .r. with time period T, then the mass of the sun is
View SolutionIf a planet of radius R and density D is revolving around the sun in a circular orbit of radius r with time period T, then the mass of the sun is
View SolutionIf a planet of mass .m. is revolving around the sun in a circular orbit of radius .r. with time period T, then the mass of the sun is
View SolutionIf a planet of radius R and density D is revolving around the sun in a circular orbit of radius r with time period T, then the mass of the sun is
View SolutionIf a planet of mass 'm' is revolving around the sun in a circular orbit of radius 'r' with time period T, then the mass of the sun is
View SolutionIf a planet of mass 'm' is revolving around the sun in a circular orbit of radius 'r' with time period T, then the mass of the sun is
View Solution
