The bisector of ∠A in △ABC meets BC in D. If AB = 15 cm, AC = 13 cm and BC = 14 cm, then DC =?
8.5 cm
8 cm
6.5 cm
7.5 cm
To find the length of segment DC in triangle ABC where the angle bisector of angle A meets side BC at point D, we can use the Angle Bisector Theorem.
1. Identify the Given Values:
- AB = 15 cm
- AC = 13 cm
- BC = 14 cm
- Let DC = x cm. Therefore, BD = BC - DC = 14 - x cm.
2. Apply the Angle Bisector Theorem:
According to the Angle Bisector Theorem, we have:
ABAC=BDDC
Substituting the known values:
1513=14−xx
3. Cross-Multiply to Solve for x:
Cross-multiplying gives us:
15x=13(14−x)
Expanding the right side:
15x=182−13x
4. Combine Like Terms:
Adding 13x to both sides:
15x+13x=182
This simplifies to:
28x=182
5. Solve for x:
Dividing both sides by 28:
x=18228=6.5 cm
6. Conclusion:
Therefore, the length of segment DC is:
DC=6.5 cm
- AB = 15 cm
- AC = 13 cm
- BC = 14 cm
- Let DC = x cm. Therefore, BD = BC - DC = 14 - x cm.
According to the Angle Bisector Theorem, we have:
ABAC=BDDC
Substituting the known values:
1513=14−xx
Cross-multiplying gives us:
15x=13(14−x)
Expanding the right side:
15x=182−13x
Adding 13x to both sides:
15x+13x=182
This simplifies to:
28x=182
Dividing both sides by 28:
x=18228=6.5 cm
Therefore, the length of segment DC is:
DC=6.5 cm
Topper's Solved these Questions
Similar Questions
The bisector of ∠A in △ABC meets BC in D. If AB = 15 cm, AC = 13 cm and BC = 14cm, then DC =?
त्रिभुज ABC में, कोण A का समद्विभाजक BC से D पर मिलता है | यदि AB = 15 सेमी, AC = 13 सेमी और BC = 14 सेमी है, तो DC =?
View SolutionThe bisector of ∠A in △???meets BC in D. If AB = 15cm, AC = 13cm and BC = 14cm, then DC=? △??? में ∠A का सम-द्विमाजक BC से D पर मिलता है। यदि AB = 15 सेमी,AC = 13 सेमी और BC = 14 सेमी है, तो DC =?
View Solution
Knowledge Check
The internal /external bisector of an angled of a triangle divides the opposite side internally / externally in the ratio of the sides containing the angle .
In the given figure ,Ae is the bisector of the exterior ∠CAD meeting BC produced in E . If AB=15 cm , AC = 8 cm and BC =14 cm , find CE .
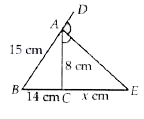 A12 cmB16 cmC20 cmD18 cm
A12 cmB16 cmC20 cmD18 cmAssertion (A) : AD is angle bisector of ∠A of the triangle ABC. If AB = 6 cm, BC = 7 cm, AC = 8 cm then BD = 3 cm and CD = 4 cm.
Reason (R) : The angle bisector AD of the triangle divides base BC in the ratio AB : AC.ABoth Assertion A and Reason R are correct and Reason R is a correct explanation of Assertion A.BBoth Assertion A and Reason R are correct but Reason R is not the correct explanation of Assertion A.CAssertion A is correct, Reason R is wrong.DAssertion A is wrong, Reason R is correct.
The bisector of ∠B in △ABC meets AC at D. If AB = 10 cm, BC= 11 cm and AC = 14 cm, then the length of AD is:
△ABC में ∠B का समद्विभाजक AC से D पर मिलता है | यदि AB =10 सेमी, BC = 11 सेमी और AC =14 सेमी है, तो AD की लंबाई क्या होगी ?
View SolutionIn the figure, AE is the bisector of the exterior ∠CAD meeting BC produced in E. If AB=10cm,AC=6cmandBC=12cm, then CE is
View SolutionIn △ABC,the bisector of ∠A intersect side BC at D. If AB=12cm, AC =15cm and BC=18cm , then the length of BD is:
△ABC में, ∠A का द्विभाजक BC को D पर प्रतिच्छेदित करता है| यदि AB = 12cm, AC = 15cm और BC= 18cm है, तो BD की लंबाई है:
View SolutionIn a ΔABC, angle bisector of interior angle A meets BC at D . if AB = 25 cm & AC = 20 cm If length of CD = 16 cm find length of BC ?
View SolutionIn △ABC, AB = 6 cm, AC = 8 cm, and BC = 9 cm. The length of median AD is:
View SolutionIn △ABC, ∠A=90∘, AD is the bisector of ∠A meeting BC at D and DE⊥AC at E. If AB =10cm and AC = 15 cm, then the length of DE, in cm is:
△ABCमें ∠A=90∘, AD, ∠A का द्विभाजक है, जो BC से D पर मिलता है, और DE⊥AC से E पर मिलता है। यदि AB = 10 cm और AC= 15 cm है, तो DE की लंबाई(cm) है,
View Solution
