In the figure, AE is the bisector of the exterior $∠ C A D$ meeting BC produced in E. If $A B = 10 c m, A C = 6 c m and B C = 12 c m$ , then CE is

Video Solution

Answer

Step by step video & image solution for In the figure, AE is the bisector of the exterior /_CAD meeting BC produced in E. If AB = 10 cm, AC = 6cm and BC = 12 cm, then CE is by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.

Updated on:21/07/2023

Class 10 MATHS TRIANGLES

Knowledge Check

The internal /external bisector of an angled of a triangle divides the opposite side internally / externally in the ratio of the sides containing the angle .
In the given figure ,Ae is the bisector of the exterior $∠ C A D$ meeting BC produced in E . If AB=15 cm , AC = 8 cm and BC =14 cm , find CE .

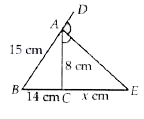A12 cm
B16 cm
C20 cm
D18 cm
In the figure AD is the external bisector of $∠ E A C$ , intersects BC produced to D. IF $A B = 12 c m, A C = 8 c m and B C = 4 c m$ , find CD :

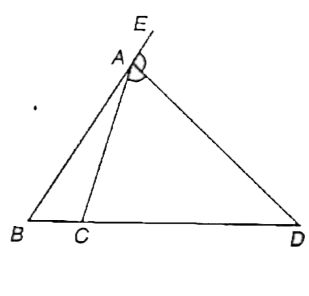A10 cm
B6 cm
C8 cm
D9 cm
In the given figure , Ad is the bisector of $∠ B A C$ . If AB = 10 cm , AC = 14 cm and BC = 8 cm , find BD and DC respectively .

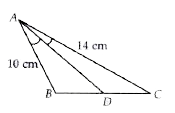A $4.7 c m, 3.3 c m$
B $3.3 c m, 4.7 c m$
C $4.3 c m, 3, 5 c m$
D $3.5 c m, 4.3 c m$

In the figure, AE is the bisector of the exterior $∠ C A D$ meeting BC produced in E. If $A B = 10 c m, A C = 6 c m and B C = 12 c m$ , then CE is

Similar Questions

Knowledge Check

In the figure AD is the external bisector of $∠ E A C$ , intersects BC produced to D. IF $A B = 12 c m, A C = 8 c m and B C = 4 c m$ , find CD :

In the given figure , Ad is the bisector of $∠ B A C$ . If AB = 10 cm , AC = 14 cm and BC = 8 cm , find BD and DC respectively .

Recommended Questions

In the figure, AE is the bisector of the exterior ∠CAD meeting BC produced in E. If AB=10cm,AC=6cmandBC=12cm, then CE is

Similar Questions

Knowledge Check

The internal /external bisector of an angled of a triangle divides the opposite side internally / externally in the ratio of the sides containing the angle . In the given figure ,Ae is the bisector of the exterior ∠CAD meeting BC produced in E . If AB=15 cm , AC = 8 cm and BC =14 cm , find CE .

In the figure AD is the external bisector of ∠EAC, intersects BC produced to D. IF AB=12cm,AC=8cmandBC=4cm, find CD :

In the given figure , Ad is the bisector of ∠BAC. If AB = 10 cm , AC = 14 cm and BC = 8 cm , find BD and DC respectively .

Recommended Questions

In the figure, AE is the bisector of the exterior $∠ C A D$ meeting BC produced in E. If $A B = 10 c m, A C = 6 c m and B C = 12 c m$ , then CE is

In the figure AD is the external bisector of $∠ E A C$ , intersects BC produced to D. IF $A B = 12 c m, A C = 8 c m and B C = 4 c m$ , find CD :

In the given figure , Ad is the bisector of $∠ B A C$ . If AB = 10 cm , AC = 14 cm and BC = 8 cm , find BD and DC respectively .