[Bengali] Let O be the circumcenter, H be the orthocentre, I be the in

The pair of lines joining the origin to the points of intersection of the curves
$a x^{2} + 2 h x y + b y^{2} + 2 g x = 0$ and
$a' x^{2} + 2 h' x y + b' y^{2} + 2 g' x = 0$
will be at right angles to one another , if

View Solution

If the chord y=mx+c subtends a right angle at the vertex of the parabola y^(2)=4ax thenthe value of c is

View Solution

If the segment joining the points (a, b), (c, d) subtends a right angle at the origin, then

View Solution

Let O be the circumcenter, H be the orthocenter, I be the incenter, and $I_{1}, I_{2}, I_{3}$ be the excenters of acute-angled $Δ A B C$
ocr_image

View Solution

If the chord y = mx +c subtends a right angle at the vertex of the parabola $y^{2} = 4 a x$ then the value of c is

View Solution

Vertex A of a variable triangle ABC, inscribed in a circle of radius R, is a fixed point. If the angles subtended by the side BC at orthocentre (H), circumcentre (O) and incentre (I) are equal than identify the locus of orthocentre of triangle ABC.

View Solution

Let $S$ be the circumcentre and $I$ be the incentre of acute angled $∠ A B C$ then the angle subtended by $S I$ at vertex $A$ is

View Solution

The tangents of the angles subtended by a tower at four points A, B, C and D on the ground are in H.P. If O be the foot of the tower on the ground, then

View Solution

Let $O$ be the circumcenter, $H$ be the orthocentre, $I$ be the incenter, and $I_{1}, I_{2}, I_{3}$ be the excenters of acute-angled $A B C .$ Column I Column II Angle subtended by $O I$ at vertex $A$ p. $| B - C |$ Angle subtended by $H I$ at vertex $A$ q. $∣ ∣ ∣ \frac{B - C}{2} ∣ ∣ ∣$ Angle subtended by $O H$ at vertex $A$ r. $\frac{B + C}{2}$ Angle subtended by $I_{2} I_{3} a t I_{1}$ $A$ q. $\frac{B}{2} - C$

View Solution

If normal at point $P$ on the parabola $y^{2} = 4 a x, (a > 0),$ meets it again at $Q$ in such a way that $O Q$ is of minimum length, where $O$ is the vertex of parabola, then $O P Q$ is
(a)a right angled triangle (b)an obtuse angled triangle (c)an acute angle triangle (d)none of these

View Solution

In $A B C, A B = A C,$ and the bisectors of angles $B$ and $C$ intersect at point $O$ . Prove that $B O = C O$ and the ray $A O$ is the bisector of angles $B A C .$

View Solution

The equation to the locus of P such that the join of (a, b) and (b, a) subtend a right angle at P, is

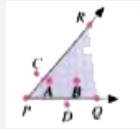
View Solution

Which of these statements is not true ?
(a) Point A and B lie in the interior of the angle
(b) Points C and D lie in the exterior of the angle.
( c )Point P is the vertex of the angle .
(d) Point R is the vertex of the angle.
ocr_image

View Solution

If the chord $y = m x + c$ subtends a right angle at the vertex of the parabola $y^{2} = 4 a x$ , thenthe value of c is

View Solution

Let $A B C$ be a triangle having $O and I$ as its circumcenter and incentre, respectively. If $R and r$ are the circumradius and the inradius, respectively, then prove that ${(O I)}^{2} = R^{2} - 2 R r .$ Further show that the triangle $B I O$ is a right angled triangle if and only if $b$ is arithmetic mean of $a and c .$

View Solution

Let $O$ be the circumcenter, $H$ be the orthocentre, $I$ be the incenter, and $I_{1}, I_{2}, I_{3}$ be the excenters of acute-angled $A B C .$ Column I Column II Angle subtended by $O I$ at vertex $A$ p. $| B - C |$ Angle subtended by $H I$ at vertex $A$ q. $∣ ∣ ∣ \frac{B - C}{2} ∣ ∣ ∣$ Angle subtended by $O H$ at vertex $A$ r. $\frac{B + C}{2}$ Angle subtended by $I_{2} I_{3} a t I_{1}$ $A$ q. $\frac{B}{2} - C$

View Solution

Topper's Solved these Questions

Similar Questions

CENGAGE PUBLICATION-TRIGONOMETRIC FUNCTIONS-All Questions