If is a variable point on the line lying between the lines and , then (b) (d) Solution in Bengali
The correct Answer is:B, D
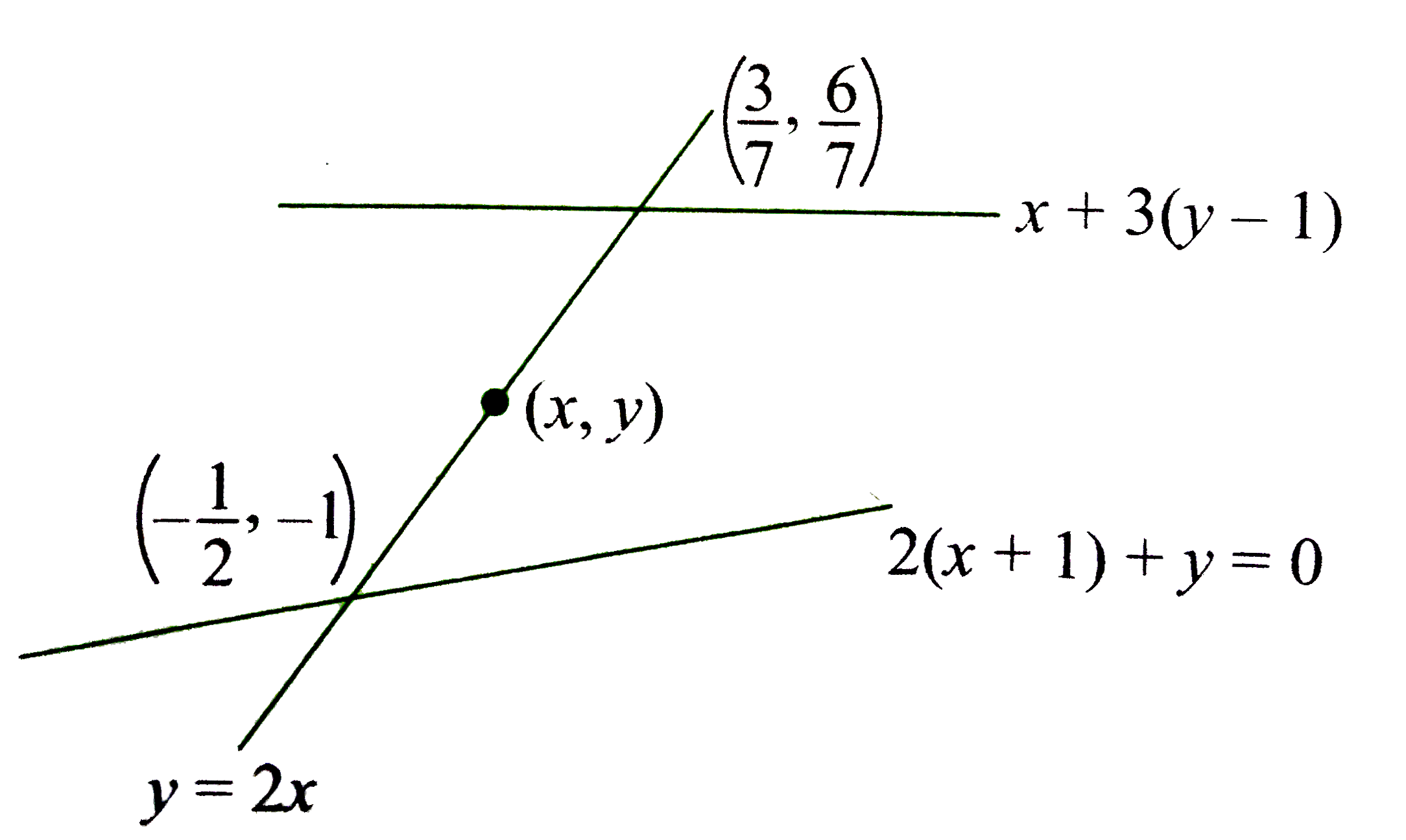
Solving y=2x, 2(x+1) + y = 0, we get x = -1/2, y=-1. Solving y=2x, x+3(y-1)=0, we get x=3/7, y=6/7.
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (LINKED COMPREHENSION TYPE)27videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MATRIX MATCH TYPE)8videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (SINGLE CORRECT ANSWER TYPE)82videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type8videos
STRAIGHT LINE
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )1videos
THEORY OF EQUATIONS
Similar Questions
Let l be the line belonging to the family of straight lines (a+2b)x+(a−3b)y+a−8b=0,a,b∈R, which is farthest from the point (2,2), then area enclosed by the line L and the coordinate axes is
02:15View SolutionMatch the following lists:
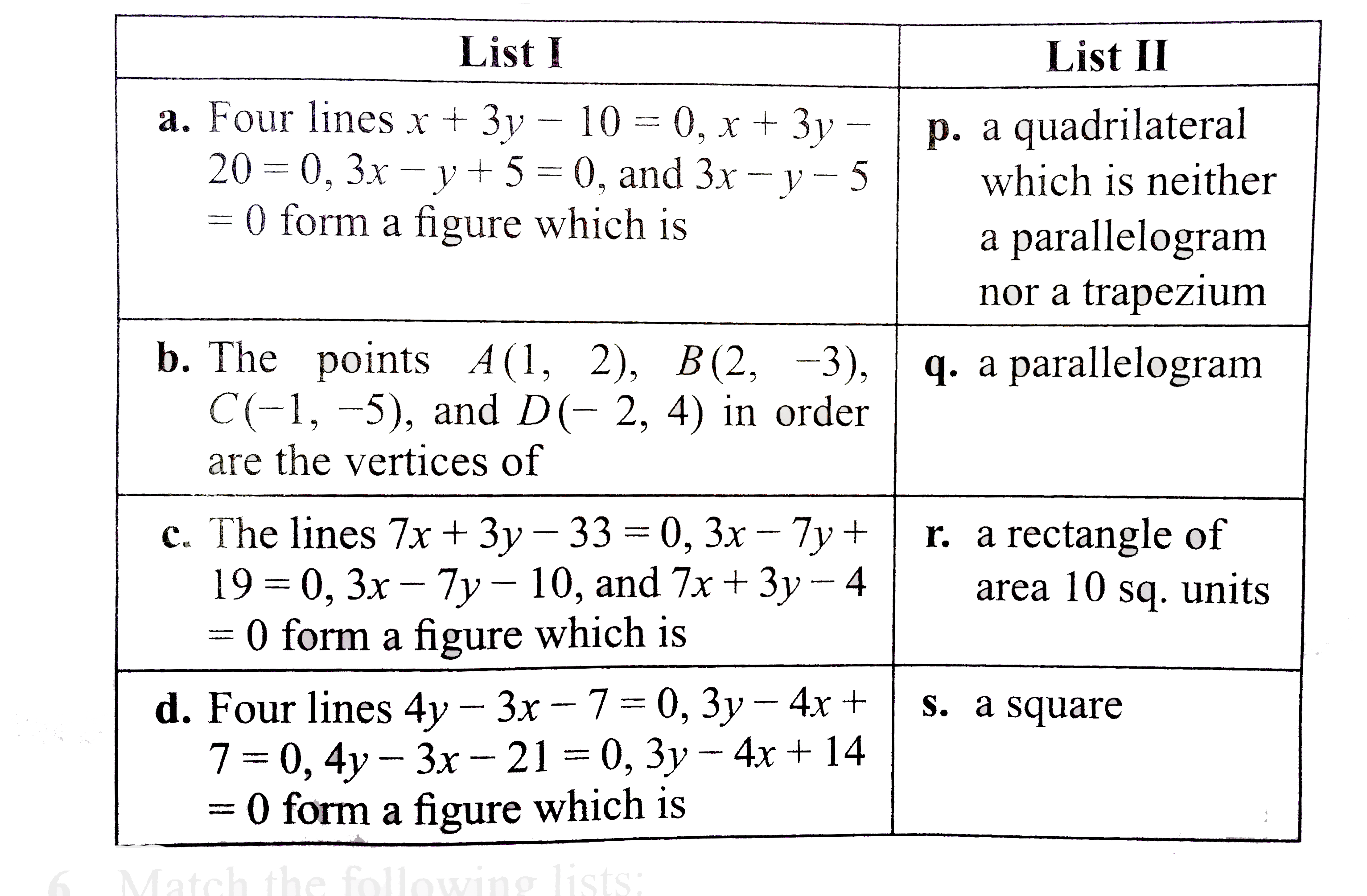 03:16View Solution
03:16View SolutionFind the equations of the diagonals of the square formed by the lines x=o,y=0,x=1andy=1.
01:49View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
05:16View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
01:11View SolutionIf (x,y) is a variable point on the line y=2x lying between the lines 2(x+1)+y=0 and x+3(y−1)=0 , then (a) x∈(−12,67) (b) x∈(−12,37) (c) y∈(−1,37) (d) y∈(−1,67)
02:03View SolutionThe equation of the plane through the line x+y+z+3=0=2x−y+3z+1 and parallel to the line x1=y2=z3 is a.x−5y+3=7 b. x−5y+3z=7 c. x+5y=3z=7 d. x+5y+3z=−7
03:54View SolutionSolve for x and y:(xy)/(x+y)=(1)/(5),x+y!=0;(xy)/(x-y)=(1)/(7),x-y!=0
03:20View SolutionThe image of line 2x+y=1 in line x+y+2=0 is : (A) x+2y−7=0 (B) 2x+y−7=0 (C) x+2y+7=0 (D) 2x+y+7=0
04:47View Solution1x+1y=7,
2x+3y=17(x≠0,y≠0) .07:27View SolutionIf x+y=7,x-y=1 then 2x =………..
06:03View SolutionColumn A0<x<yColumn B71x−1y7x−y
04:04View SolutionThe equation of the line passing through the point of intersection of the lines 2x+y+1=0,x−y−7=0 and the point (3, -2) is
03:13View Solution(x, y), (1, 2) ಮತ್ತು (7, 0) ಬಿಂದುಗಳು ಸರಳರೇಖಾಗತವಾಗಿದ್ದರೆ, x ಮತ್ತು y ಗಳ ನಡುವೆ ಒಂದು ಸಂಬಂಧವನ್ನು ಕಂಡುಹಿಡಿಯಿರಿ.
05:37View Solution(x,y),(1,2) ಮತ್ತು(7,0) x ಮತ್ತುy ಬಿಂದುಗಳಿಂದ ಉಂಟಾಗುವ ರೇಖಾಖಂಡವನ್ನು ವಿಭಾಗಿಸುವ ಅನುಪಾತವನ್ನು ನಿರ್ಧರಿಸಿ.
03:13View SolutionThe angles between the lines x+1−3=y−32=z+21 and x=y−7−3=z+72 are
05:05View Solution

