Prove that the image of point in the line having slope and passing through origin is .Solution in Bengali
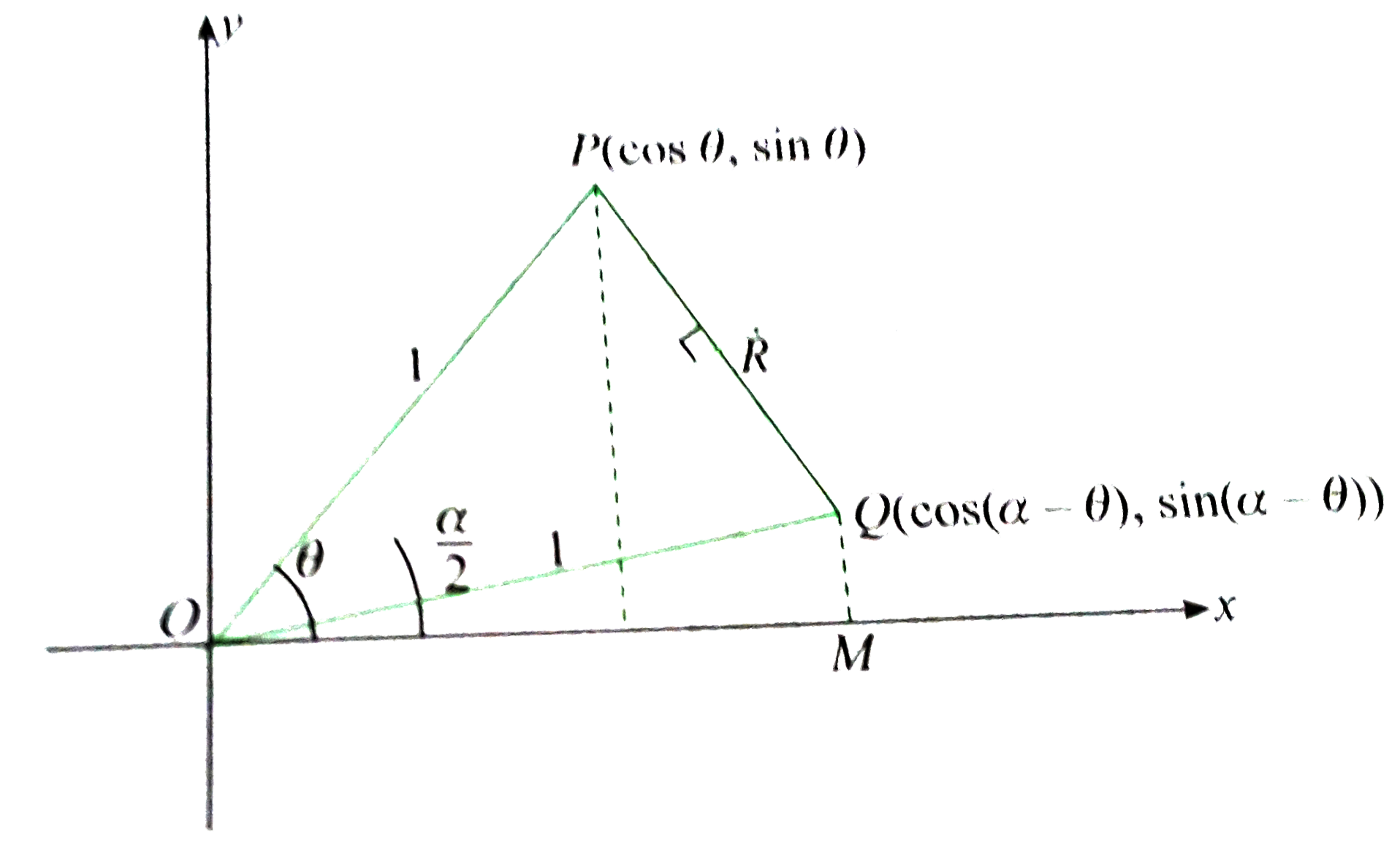
Clearly,
Now, we have to prove that Q is the image of P in the line OR which has slope tan .
Triangle is isosceles triangle.
If Q is the image of P in line OR, then OR is the perpendicular bisector of PQ.
We have to prove that .
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.1
6 Videos
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.2
8 Videos
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Illustration1.67
1 Videos
- COORDINATE SYSTEMBOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGE PUBLICATIONCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
What is the minimum area of a triangle with integral vertices ?
View SolutionFind the distance between the points P(asinα,acosα) and Q(acosα,−asinα)
View SolutionIf A(cosα,sinα),B(sinα,−cosα),C(1,2) are the vertices of ABC, then as α varies, find the locus of its centroid.
View SolutionCoordinates of points on curve 5x2−6xy+5y2−4=0 which are nearest to origin are
View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
View Solutiontanθ=sinα−cosαsinα+cosα
View SolutionLet 0
View SolutionIf tan theta=(sin alpha-cos alpha)/(sin alpha+cos alpha), prove that 2cos^(2)theta=1+sin2 alpha
View SolutionIf tan theta=(cos alpha-sin alpha)/(cos alpha+sin alpha) and theta is acute then theta+alpha=
View Solutionsin(3 theta+alpha)+sin(3 theta-alpha)+sin(alpha-theta)-sin(alpha+theta)=cos alpha
View SolutionIf tan alpha=(sin theta-cos theta)/(sin theta+cos theta) then sin alpha
View SolutionProve that the image of point P(cosθ,sinθ) in the line having slope tan(α/2) and passing through origin is Q(cos(α−θ),sin(α−θ)).
View SolutionIf tanθ=sinα−cosαsinα+cosα, then
View SolutionIftanθ=QsinαP+Qcosα,prove that,tan(α−θ)=PsinαQ+Pcosα.
View SolutionIf tanθ=sinα−cosαsinα+cosα,prove that,2cos2θ=1+sin2α.
View SolutionIf tan alpha =(sin theta - cos theta)/(sin theta + cos theta) If so sin alpha The value of
View Solution
