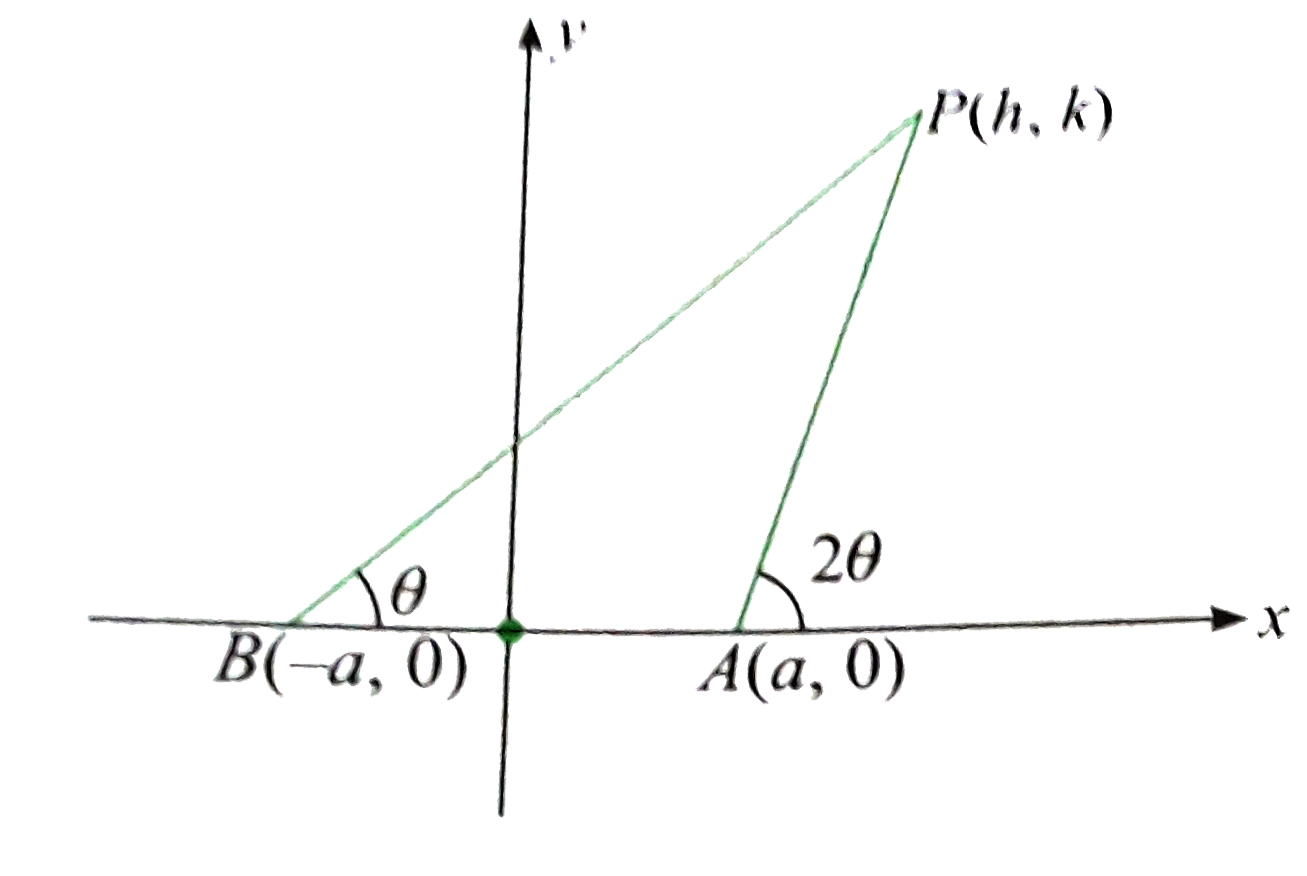
Two straight lines rotate about two fixed points. If they start from their position of coincidence such that one rotates at the rate double that of the other. Prove that the locus of their point of intersection is a circle.Solution in Bengali
Let PB make angle θ and PA make angle 2θ with x-axis.
Let P be (h,k).
tanθ=kha (1)
tan2θ=kh−a (2)
From (2), we get
2tanθ1−tan2θ=kh−a
Putting value of tanθ from (1),we get
2(kh+a)1−(kh+a)2=kh−a
⇒h2k2−2ah−3a2=0
⇒x2+y62−2ax−2a2=0
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.16videos
COORDINATE SYSYEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.28videos
COORDINATE SYSYEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Illustration1.671videos
COORDINATE SYSYEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type2videos
COORDINATE SYSTEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.213videos
CROSS PRODUCTS
Similar Questions
What is the minimum area of a triangle with integral vertices ?
05:15View SolutionFind the distance between the points P(asinα,acosα) and Q(acosα,−asinα)
04:45View SolutionIf A(cosα,sinα),B(sinα,−cosα),C(1,2) are the vertices of ABC, then as α varies, find the locus of its centroid.
04:59View SolutionCoordinates of points on curve 5x2−6xy+5y2−4=0 which are nearest to origin are
02:31View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
09:27View SolutionTwo rods are rotating about two fixed points in opposite directions. If they start from their position of coincidence and one rotates at the rate double that of the other, then find the locus of point of the intersection of the two rods.
01:14View SolutionTwo straight lines rotate about two fixed points (-a, 0) and (a, 0) in antic clockwise direction. If they start from their position of coincidence such that one rotates at a rate double of the other, then locus of curve is
06:03View SolutionTwo circles intersect each other at the points P and Q. Two straight lines through P and Q intersect one circle at the points A and C and the other circle at B and D. Prove the AC|| BD
08:51View SolutionA तथा B दो स्थिर बिन्दु है, तथा रेखा AB किसी चर बिन्दु P पर यदि समकोण अंतरित करती है, तो सिद्ध कीजिए कि चर बिन्दु P का बिन्दुपथ एक वृत्त है।
04:30View SolutionTwo circles intersect each other at the points G and H . A straight line is drawn through the point G which intersect two circles at the points P and Q and the straight line through the point H parallel to PQ intersects the two circles at the points R and S . Prove that PQ=RS .
07:22View SolutionIf the straight line intersects the circle at two points, then the straight line is called a _____ to the circle.
04:52View SolutionTwo straight lines rotate about two fixed points (−a,0)and(a,o). If they start from their position of coincidence such that oen rotates at the rate double that of the other, then
The point(−a,0) always lies06:31View SolutionTwo equal circles are centered on each other and the two circles intersect at points A and B. By intersecting two straight line circles at points C and points D, we prove that, triangleBCD Equilateral triangle.
06:22View SolutionProve that if the two tangents drawn at the two points on the top of the circle intersect, then the lengths of the two straight lines drawn from the point of intersection to the point of tangent will be equal.
05:51View SolutionTwo rods are rotating about two fixed points in opposite directions. If they start from their position of coincidence and one rotates at the rate double that of the other, then find the locus of point of the intersection of the two rods.
View SolutionTwo straight lines rotate about two fixed points (-a, 0) and (a, 0) in antic clockwise direction. If they start from their position of coincidence such that one rotates at a rate double of the other, then locus of curve is
06:52View Solution

