Draw and discuss the graph of f(x)=x2/3−x4/3Solution in Bengali
We have f(x)=x2/3−x4/3
Clearly, the domain of the function is R.
f(x)=x2/3(1−x2/3)=x2/3(1−x1/3)(1+x1/3)
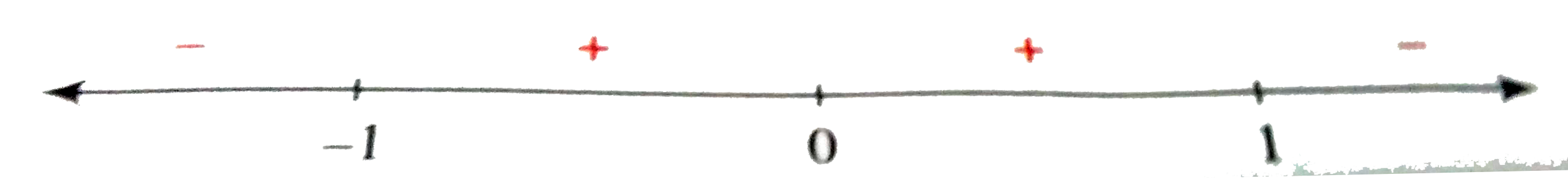
Sign of scheme of y=f(x) is as follows:
f′(x)=23x−13−43x13
=231−2x2/3x1/3
=−43(x1/3−1√2)x13+1√2x13
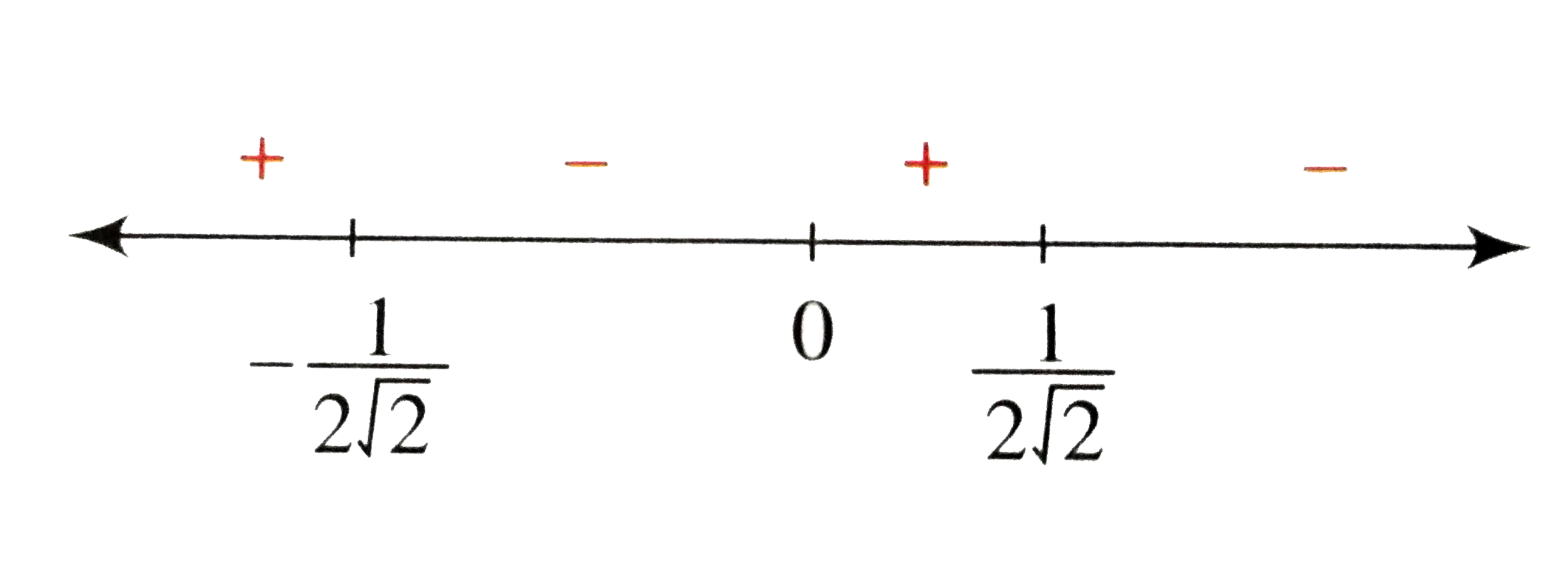
f′(x)=0∴x=±12√2
Also, f′(x) does not exist at x=0
it is also a critical point.
Sign scheme of f′(x) is as follows:

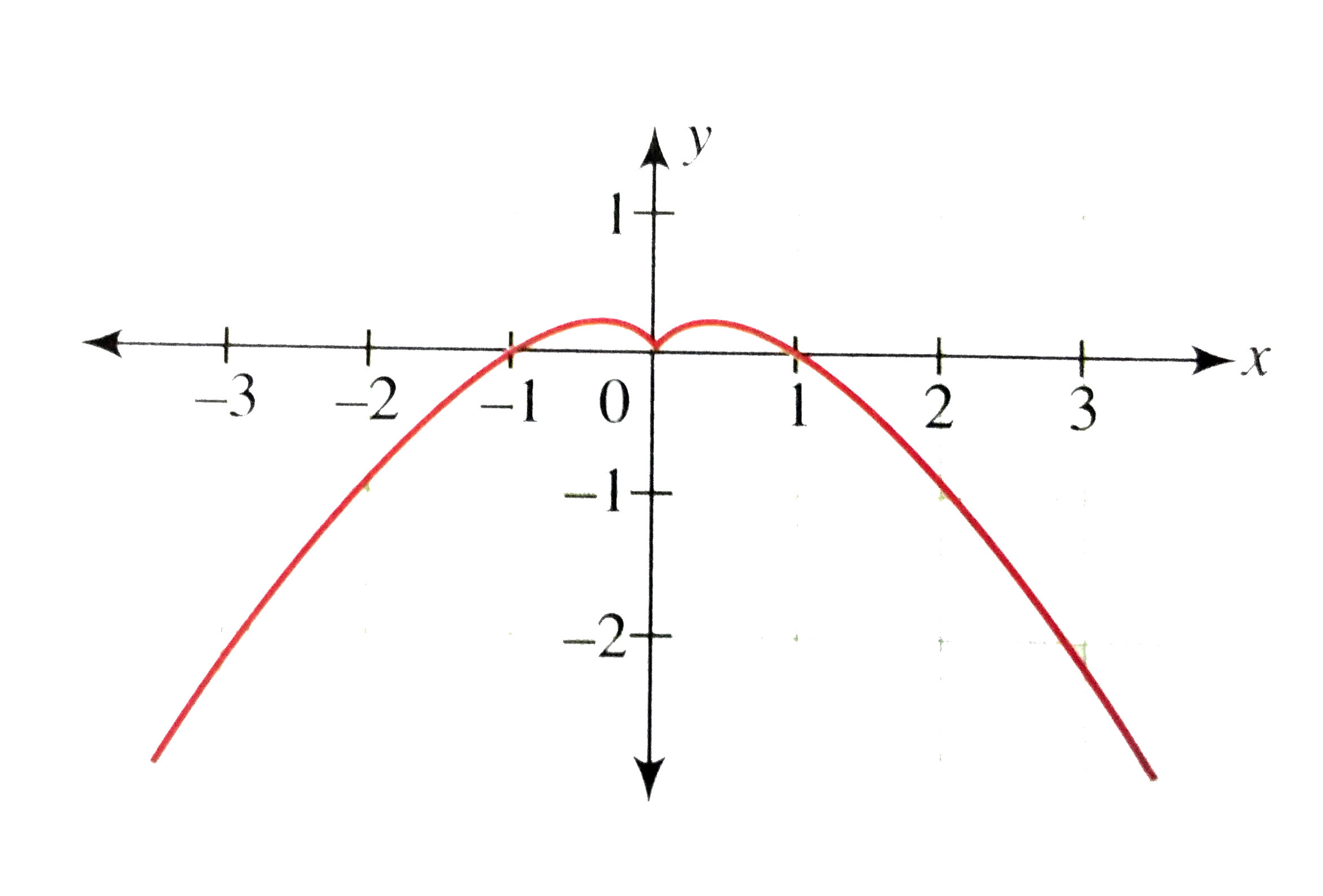
Thus, x=0 is the point of minima and x=±12√2 are the points of maxima.
f(0)=0,f(x)=0∴x=0,±1
f(±1)=0
Also when, x→±∞,f(x)→−∞
y=f(x) is an even function, so the graph is symmetrical about the y-axis.
At x=0, the graph has vertical tangent line x=0, where the function is non-differentiable.
From the above discussion, the graph of the function is as shown in the following figure.
Topper's Solved these Questions
Similar Questions
Find the derivative of y=sin2x−cosx
04:13View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
01:53View SolutionLet f be a non-negative function defined on the interval [0,1]. If ∫x0√1−(f′(t))2dt=∫x0f(t)dt,0≤x≤1,and f(0)=0, then
01:13View SolutionDiscuss the maxima and minima of the function f(x)=x23−x43. Draw the graph of y=f(x) and find the range of f(x).
05:15View SolutionDraw the graph of f(x)=(x−1)|(x−2)(x−3)|.
02:50View SolutionDraw the graph of f(x)=x3−xx2−1.
06:28View SolutionDraw and discuss the graph of f(x)=x2/3−x4/3
03:11View SolutionDraw the graph of f(x)=e−x2. Discuss the concavity of the graph.
01:39View SolutionDiscuss the maxima and minima of the function f(x)=x23−x43. Draw the graph of y=f(x) and find the range of f(x).
05:37View SolutionDraw the graph of f(x)=(x−1)|(x−2)(x−3)|.
03:13View SolutionDraw the graph of f(x)=x3−xx2−1.
01:59View SolutionDraw and discuss the graph of f(x)=x2/3−x4/3
05:20View SolutionDraw the graph of f(x)=e−x2. Discuss the concavity of the graph.
06:41View SolutionDraw the graph of f(x)=x2−3
02:33View SolutionLet f(x)=2x3=9x2+12x+6. Discuss the global maxima and minima of f(x)∈[0,2]and(1,3) and, hence, find the range of f(x) for corresponding intervals.
04:36View SolutionDraw and discuss the graph of f(x) = x^(2//3)-x^(4//3)
01:52View Solution

