Suppose a parabola y=x2−ax−1 intersects the coordinate axes at three points A,B and C, respectively. The circumcircle of ΔABC intersects the y-axis again at the point D(0,t). Then the value of t is
1/2
1
3/2
2
The correct Answer is:B
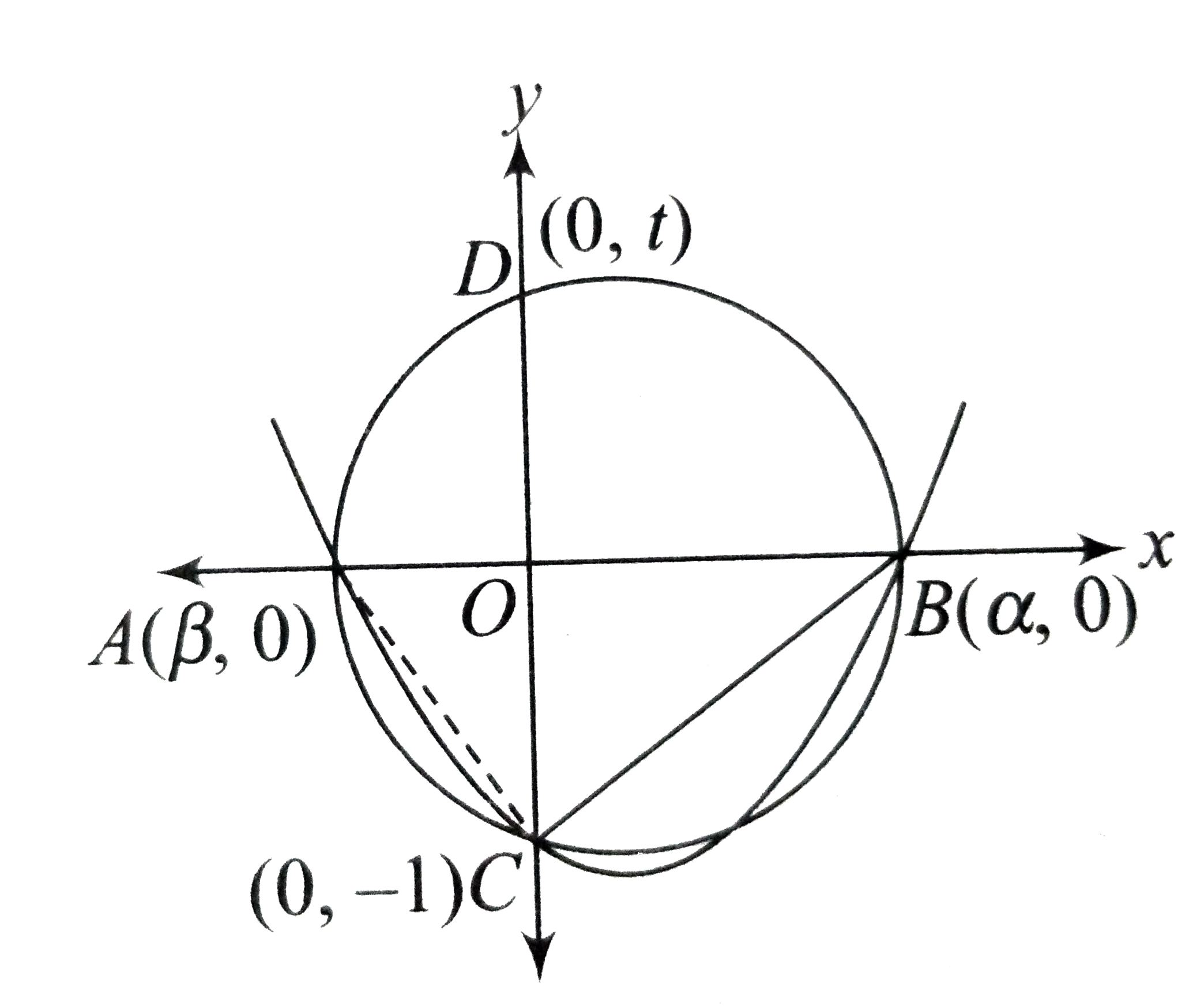
We have x=a±√a2+42
∴α=a+√a2+42,β=a−√a2+42
Equation of family of circles through A and B is
(x−α)(x−β)+y2+λy=0
As it passes through C(0,−1) we have
αβ+1−λ=0 (But αβ=−1)
⇒−1+1−λ=0
⇒λ=0
∴ Equation of circle through A,B and C is
(x−α)(x−β)+y2=0
It cuts the y-axis when x=0, so
αβ+y2=0
y2=1 (Put αβ=−1)
⇒y=1 or −1
Hence t=1
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - PARABOLAEXERCISE - Multiple Correct Answers Type10videos
PARABOLA
- BOOK - CENGAGE PUBLICATIONCHAPTER - PARABOLAEXERCISE - Matching Column Type1videos
PARABOLA
- BOOK - CENGAGE PUBLICATIONCHAPTER - PARABOLAEXERCISE - Comprehension Type2videos
PARABOLA
- BOOK - CENGAGE PUBLICATIONCHAPTER - PAIR OF STRAIGHT LINESEXERCISE - Numberical Value Type5videos
PAIR OF STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - PERMUTATION AND COMBINATIONEXERCISE - Comprehension8videos
PERMUTATION AND COMBINATION
Similar Questions
The tangent and the normal at the point A≡(4,4) to the parabola y2=4x, intersect the x-axis at the point BandC respectively. The equation to the circumcircle of ΔABC is (A) x2+y2−4x−6y=0 (B) x2+y2−4x+6y=0 (C) x2+y2+4x−6y=0 (D) none of these
View SolutionIf the lines 2x+3y−1=0andkx−y+4=0 intersect the coordinate axes in concyclic points then k−1=
View Solution
Knowledge Check
- Question 1 - Select One
A line L1=x10+y8=1 intersects the coordinate axes at points A and B. Another line L2 perpendicular to L1 intersects the coordinate axes at C and D. The locus of circumcentre of ΔABD is
A5x−4y=9B5x−4y=18C4x−5y=9D4x−5y=18 - Question 2 - Select One
Ordinates of three points A,B,C on the parabola y2=4ax are in G.P. Tangents at A and C intersect on
Aline through B parallel to x-axisBline through B|| to y-axisCline through B and vertex of parabolaDline through B and focus of parabola - Question 3 - Select One
If the circles x2+y2+5x−6y−1=0andx2+y2+ax−y+1=0 intersect orthogonally (the tangents at the point of intersection of the circles are at right angles), the value of a is
A6/5B5/6C−6/5D−5/6
If the normal to the parabola y2=4ax at point t1 cuts the parabola again at point t2.Then the minimum value of t22 is
View SolutionThe line y=x−2 cuts the parabola y2=8x in the points A and B. The normals drawn to the parabola at A and B intersect at G. A line passing through G intersects the parabola at right angles at the point C, and the tangents at A and B intersect at point T.
View SolutionTangents PTandQT to the parabola y2=4x intersect at T and the normal drawn at the point PandQ intersect at the point R(9,6) on the parabola. Find the coordinates of the point T. Show that the equation to the circle circumscribing the quadrilateral PTQR, is (x−2)(x−9)+(y+3)(y−6)=0.
View SolutionLet lx−2y=1 intersects the parabola y2=4ax at points P and Q. If PS and QS meet the parabola again at R and T respectively (where S is the focus of y2=4ax) then the slope of RT is equal to
View SolutionIf a tangent to the parabola y2=4ax intersects the x2a2+y2b2=1 at Aand B, then the locus of the point of intersection of tangents at A and B to the ellipse is
View Solution

