If a circle passes through the point $(a, b)$ and cuts the circle $x^{2} + y^{2} = 4$ orthogonally, then the locus of its centre is (a) $2 a x + 2 b y - (a^{2} + b^{2} + 4) = 0$ (b) $2 a x + 2 b y - (a^{2} - b^{2} + k^{2}) = 0$ (c) $x^{2} + y^{2} - 3 a x - 4 b y + (a^{2} + b^{2} - k^{2}) = 0$ (d) $x^{2} + y^{2} - 2 a x - 3 b y + (a^{2} - b^{2} - k^{2}) = 0$

A

$2 a x + 2 b y - (a^{2} + b^{2} + k^{2}) = 0$

B

$2 a x + 2 b y - (a^{2} - b^{2} + k^{2}) = 0$

C

$x^{2} + y^{2} - 3 a x - 4 b y + (a^{2} + b^{2} - k^{2}) = 0$

D

$x^{2} + y^{2} - 2 a x - 3 b y + (a^{2} - b^{2} - k^{2}) = 0$

Text Solution

Verified by Experts

The correct Answer is:1

Let the equation of the circle through (a,b) be
$x^{2} + y^{2} + 2 g x + 2 f y + c = 0$ (1)
SO, $a^{2} + b^{2} + 2 a g + 2 b f + c = 0$ (2)
Since circle (1) cuts $x^{2} + y^{2} = k^{2}$ orthogonally, we have
$2 g (0) + 2 f (0) = c - k^{2}$ or $c = k^{2}$
Putting $c = k^{2}$ in (2) ,we get
$2 a g + 2 b f + (a^{2} + b^{2} + k^{2}) = 0$
So, the locus of the center $(- g, - f)$ is
$- 2 a x - 2 b y + (a^{2} + b^{2} + k^{2}) = 0$ ltbrlt or $2 a x + 2 b y - (a^{2} + b^{2} + k^{2}) = 0$
Alternate Methode $:$

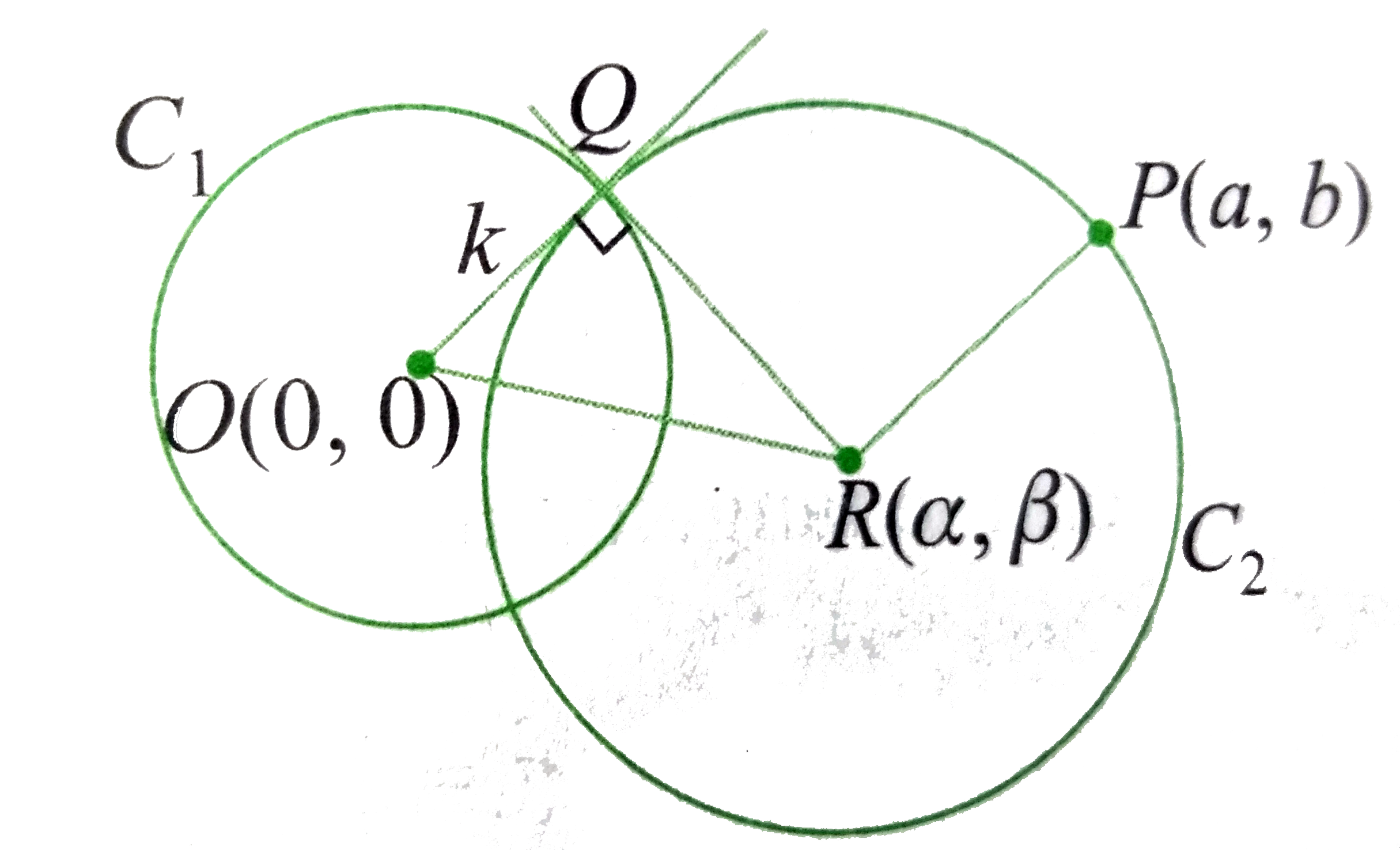
As shown in the figure, circle $C_{20}$ intersect the circle $C_{1} : x^{2} + y^{2} = k^{2}$ orthogonally at point Q.
Circle $C_{2}$ passes through the point P(a,b) . We have to fnd the locus of center $R (α, β)$ of circle $C_{2}$
Now from the figure, $R Q = P =$ radiua of the circle $C_{2}$
$∴ R P^{2} = R Q^{2}$
$∴ R P^{2} = {or}^{2} - O Q^{2}$
$∴ {(α - a)}^{2} + {(β - b)}^{2} = (α^{2} + β^{2}) - k^{2}$
$∴$ locus is $2 a x + 2 b y - (a^{2} + b^{2} + k^{2}) = 0$

|

Updated on:21/07/2023

Class 12 MATHS CIRCLE

Topper's Solved these Questions

Similar Questions

CENGAGE PUBLICATION-CIRCLE -Excercises (Single Correct Answer Type)

Home
Ask Doubt
Profile