Question

Area bounded by the ellipse $\frac{x^2}{4}+\frac{y^2}{9}=1$ is equal to

Options

A. `x^(2)+y^(2)=6.5`
B. `x^(2)+y^(2)=5`
C. `x^(2)+y^(2)=4.5`
D. none of these

Explanation

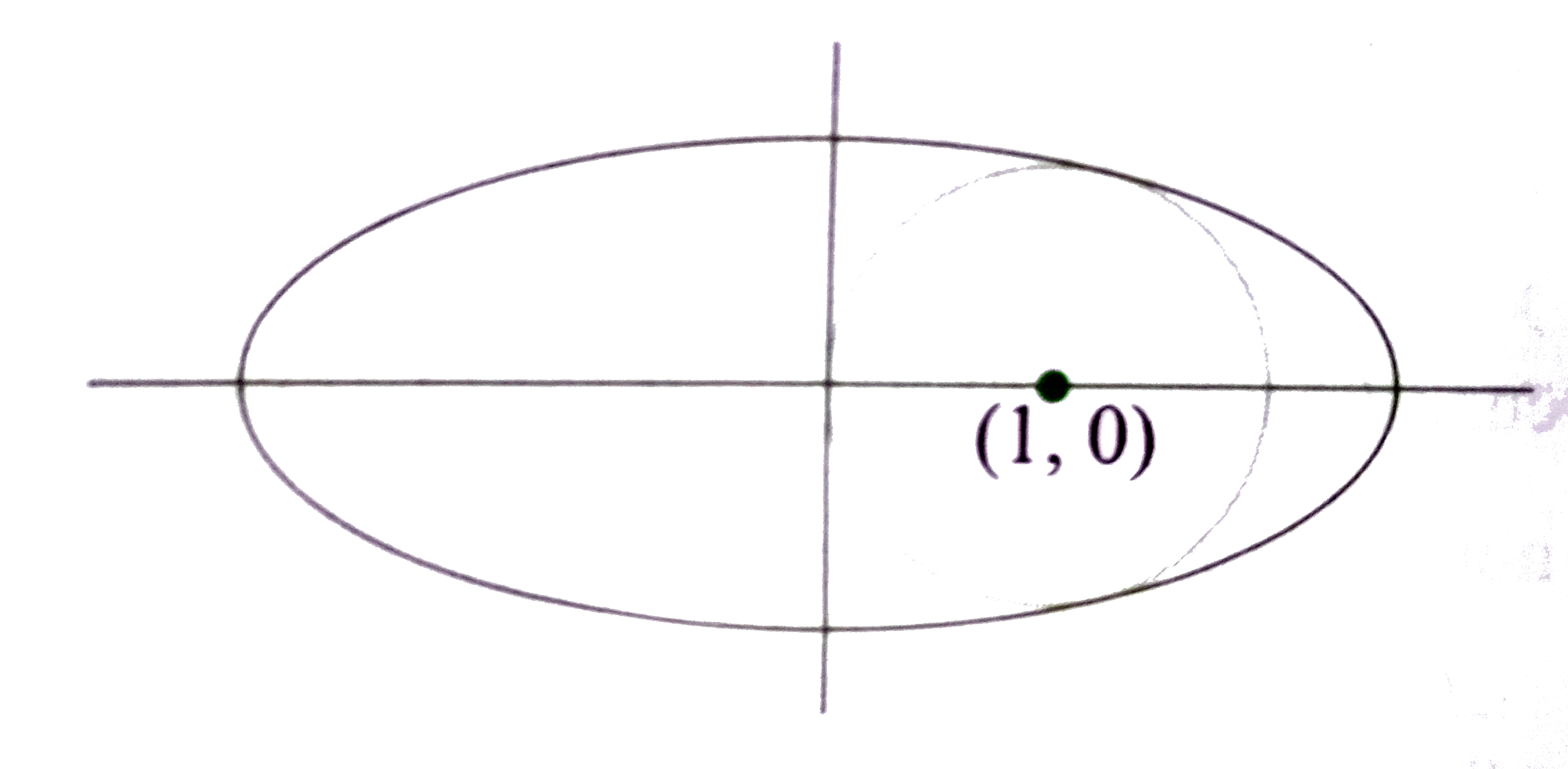
Solving both equations we have
`(x^(2))/(a^(2))+(1-(x-1)^(2))/(b^(2))=1`
or `b^(2)x^(2)+a^(2)[1-(x-1)^(2)]=a^(2)b^(2)`
or `(b^(2)-a^(2))x^(2)+2a^(2)x-a^(2)b^(2)=0" "(1)`
For leat area, the circle.
Therefore, the discriminant of (1) is zero. Thus,
`4a^(2)+4a^(2)b^(2)(b^(2)-a^(2))=0`
or `a^(2)+b^(2)(b^(2)-a^(2))=0`
or `a^(2)+b^(2)(-a^(2)e^(2))=0`
or `1-be^(2)e^(2)=0`
`or b=(1)/(e)`
Also, `a^(2)=(b^(2))/(1-e^(2))=(1)/(e^(2)(1-e^(2)))`
`or a=(1)/(esqrt(1-e^(2)))`
Let S be the area of the ellipse. Then,
`S=piab=(pi)/(e^(2)sqrt(1-e^(2)))=(pi)/(sqrt(e^(4)-e^(6)))`
The area is minimum if `(f)(e)=e^(2)-e^(6)` is maximum, i.e., when `f'(e)=4e^(3)-6e^(5)=0`
or `sqrt((2)/(3))` [ which is the point of maxima for f(e)]
So,S is the least when `e=sqrt(2//3)`
Therfore, the ellipse is `x^(2)+y^(2)=4.5`
Length of loatus rectum of ellipe `=(2b^(2))/(a)=(2xx(9)/(6))/((3)/(sqrt(2)))=sqrt(2)`