In cyclic quadrilateral ABCD (none of these being 90∘), which of the following is not true ?
tan A cot C = -1
sec B cos D = -1
cosec B sin D = 1
none of these
The correct Answer is:D
∠A=π−C
⇒tanA=−tanC
⇒AcotC=−1
∠B=π−D
⇒secB=−secD
⇒secBcosD=−1
⇒∠B=π−D
⇒cosecBsinD=1
Topper's Solved these Questions
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Archives JEE Advanced Multiple correct answers type
1 Videos
- TRIGONOMETRIC EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Numerical value type)
4 Videos
- TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASBOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Archives (Numerical Value Type)
2 Videos
Similar Questions
Knowledge Check
In cyclic quadrilateral ABCD, if cotA=34 and tanB=−125, then which of the following is (are) correct ?
AsinD=1213Bsin(A+B)=1665CcosD=−1513Dsin(C+D)=−1665ABCD is a cyclic quadrilateral and A+B=2(C+D). If ∠C>30∘, then which of the following is true
AA)∠D≥90∘BB)∠D<90∘CC)∠D≤90∘DD)∠D>90∘In a cyclic quadrilateral ABCD, the value of 2+∑cosAsocB, is
Asin2A+sin2B, isBsin2B+sin2, DCsin2A+sin2CDsin2B+sin2C
In a cyclic quadrilateral ABCD, the value of 2+∑cosAcosB, is
View SolutionFor the cyclic quadrilateral shown below, which of the following is always true?
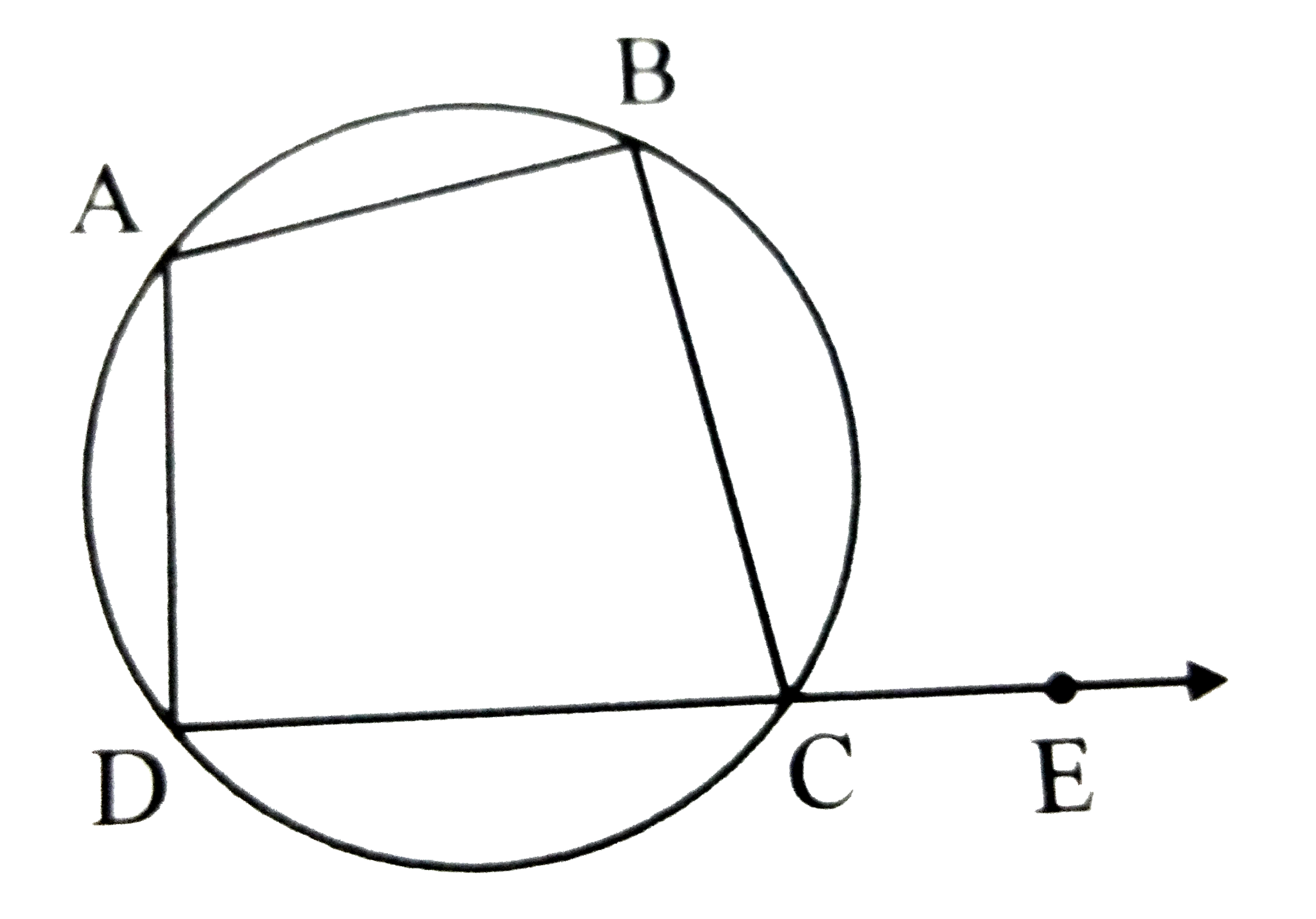
View SolutionThe area of any cyclic quadrilateral ABCD is given by A2=(s−a)(s−b)(s−c)(s−d), where 2s=a+b++c+d,a,b,candd are the sides of the quadrilateral
Now consider a cyclic quadrilateral ABCD of area 1 sq. unit and answer the following question
The minium perimeter of the quadrilateral is
View SolutionThe diagonals AC and BD of a cyclic quadrilateral ABCD intersect each other at the point P. Then,it is always true that
View SolutionThe area of any cyclic quadrilateral ABCD is given by A2=(s−a)(s−b)(s−c)(s−d), where 2s=a+b++c+d,a,b,candd are the sides of the quadrilateral
Now consider a cyclic quadrilateral ABCD of area 1 sq. unit and answer the following question
The minium perimeter of the quadrilateral is
View Solution
